Forced Oscillation-Definition, Equation, & Concept of Resonance in Forced Oscillation

Definition of Forced Oscillation

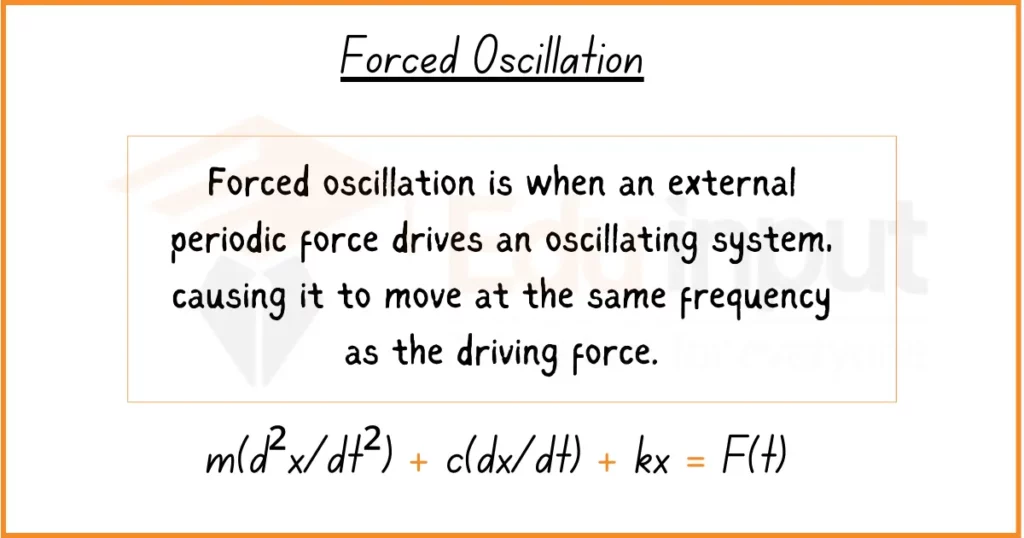
A forced oscillation is a type of Oscillation that occurs when an external force repeatedly pushes or pulls on an object at a specific rhythm. It causes the object to move back and forth. This external force keeps adding energy to the system, and maintains the object’s motion even if forces are trying to slow it down.

Analogy to Understand Forced Oscillation

Imagine you’re pushing a child on a swing in a playground. This scenario is a perfect analogy for forced oscillation:

1. The Swing (Oscillator): The swing naturally wants to move back and forth at a certain speed. This is like its natural frequency.
2. The Child (Mass): The child on the swing represents the mass in the system.
3. You Pushing (Driving Force): When you push the child, you’re applying a forced oscillation. You’re the external force adding energy to the system.
4. Timing of Pushes (Frequency): If you push randomly, the swing might not go very high. But if you time your pushes just right – pushing when the swing is at its furthest point from you – you can make the swing go higher and higher. This is like matching the driving force’s frequency to the swing’s natural frequency.
5. Resistance (Damping): Air resistance and friction at the swing’s pivot point try to slow the swing down. This is like damping in a forced oscillation system.
6. Resonance: When you push at just the right timing (matching the swing’s natural back-and-forth rhythm), the swing goes highest. This is resonance – when the driving force’s frequency matches the natural frequency of the system.
7. Amplitude: How high the swing goes is like the amplitude of the oscillation. With well-timed pushes, even gentle pushes can make the swing go quite high over time.

Forced Oscillation Equation

1. General form (Driven Harmonic Oscillator Equation)

m(d²x/dt²) + c(dx/dt) + kx = F(t)

Where:

• m is the mass of the oscillating object
• x is the displacement from the equilibrium
• t is time
• c is the damping coefficient
• k is the spring constant
• F(t) is the external forcing function

2. Steady-state solution for sinusoidal forcing

x(t) = Acos(w_d t) + Bsin(w_d t)

Where:

• x(t) is the position of the oscillating object in terms of time, t
• A and B are the amplitudes of oscillation
• w_d is the angular driving frequency

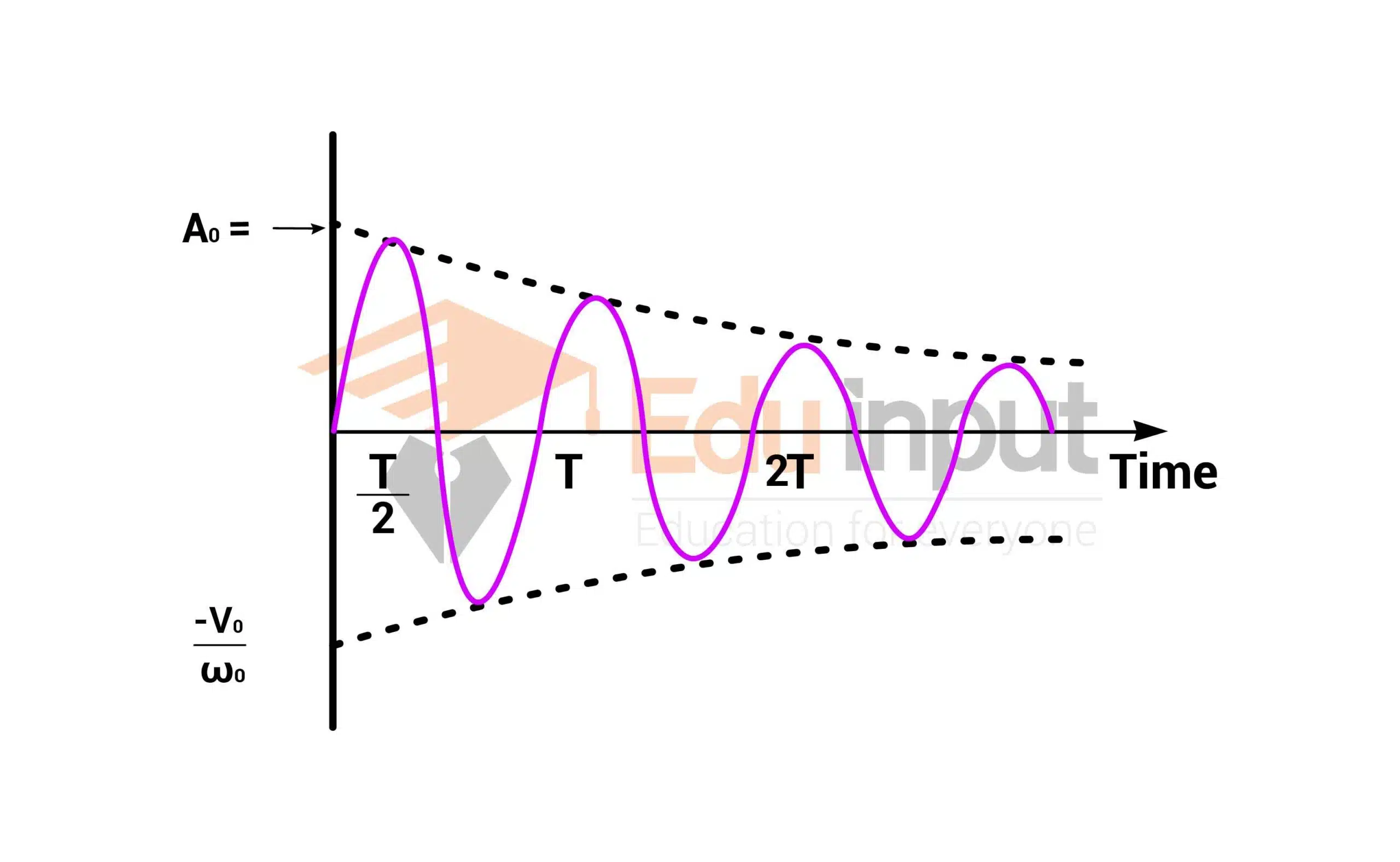
This second form represents the steady-state solution when the forcing function is sinusoidal and damping effects are negligible or ignored. It’s important to note that the complete solution in most real-world scenarios would include both transient and steady-state components.

Concept of Resonance in Forced Oscillation

Resonance is a special condition that occurs in forced oscillations when the external driving force’s frequency matches the oscillating system’s natural frequency. It’s like finding the perfect rhythm to push a swing. When resonance happens, the system responds dramatically, reaching its maximum swing or vibration.

Characteristics of a System Oscillating in Resonance

1. At resonance, the system reaches its highest amplitude (biggest swing). This happens when the driving frequency perfectly matches the system’s natural frequency. It’s like a singer hitting just the right note to make a wine glass vibrate intensely.
2. Systems with less damping (less friction or resistance) can achieve higher amplitudes at resonance. Imagine a swing in outer space with no air resistance – it could go incredibly high with small pushes.
3. For systems with low damping, the resonance peak becomes narrower. This means you need to be more precise with the driving frequency to achieve resonance. It’s like tuning a radio to a very specific frequency to get a clear signal.
4. At resonance, even small driving forces can produce large amplitude responses over time. This is why a child can make a heavy swing go high with small, well-timed pushes.
5. Resonance is when energy transfer from the driving force to the oscillator is most efficient. It’s like when you’re in perfect sync pushing a swing – your effort feels most effective.
6. The “sharpness” of the resonance is measured by the quality factor (Q). A higher Q means a narrower, more pronounced resonance peak. It’s calculated as Q = Δω / ω0, where Δω is the width of the resonance peak at half its maximum height, and ω0 is the natural frequency.