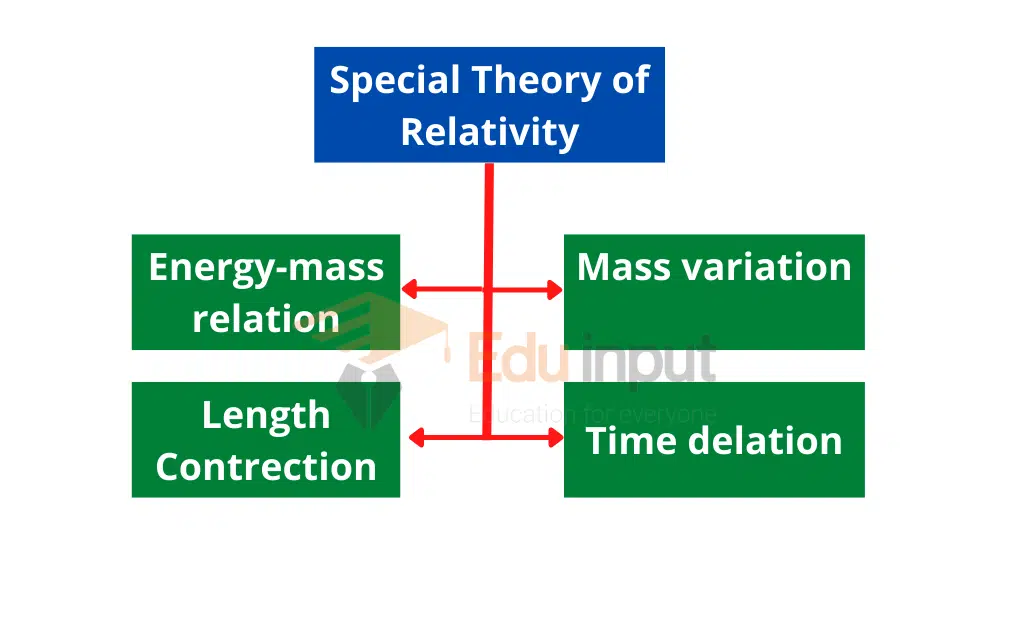
Special Theory of Relativity | Time Dilation, Length Contraction, Mass Variation, and Energy Mass Relation

The special theory of relativity deals with those cases in which observers is a state of relative motion. There are two types of theories

• The general theory of relativity
• The special theory of relativity

General Theory of Relativity

The general theory of relativity deals with the problems involving frames of reference accelerating with respect to one another.

Special Theory Of Relativity

The theory of relativity which deals with the problems involving inertial or non-accelerating frames of reference is called the special theory of relativity.

The special theory of relativity is based upon two postulates.

• The laws of physics are the same in all inertial frames.
• The speed of light in free space is constant and its value is independent of the motion of the source of light or the motion of the observer.

The first postulate is the generalization of the fact that all physical laws are the same in the frame of reference moving with uniform velocity with respect to one another.

If the laws of physic were different for different observers in relative motion, then one could determine from this difference which of them were stationary in space and which were moving.

But such a distinction does not exist, so this postulate shows that there is no way to detect absolute uniform motion.

The second postulate states an experimental fact that the speed of light in free space is the universal constant ‘c’ (c=3×10⁸ ms^–¹).

Some interesting results of the special theory of relativity can be summarized as follow

• Time dilation
• Length contraction
• Mass variation
• Energy mass relation

Time Dilation

According to the special theory of relativity, time is not an absolute quantity. But time depends upon the motion of the frame of reference.

Suppose an observer is stationary in an inertial frame. He measures the time interval between two events called proper time.

Now, if the observer is moving with respect to the frame of reference of events with velocity V or if the frame of events is moving with respect to an observer with a uniform velocity V the time measured by the observer would not be t_o but it would be t given by.

T= t_o / √1-(v²/c²)

As the quantity √1-(v2/c2) is always less than one so t>t_o.

Time has dilated or stretched due to the relative motion of the observer and frame of reference of events.

Length Contraction

The distance from the earth to the star or distance between two points measured by an observer in a moving spaceship would seem smaller than the distance measured by another observer on earth.

This effect is known as length contraction. The distance between two points appears shorter than if it is measured at rest relative to them.

This is known as Lorentz contraction of length. The length contraction happens only along the direction of motion. But no length contraction is observed perpendicular to the direction of motion.

Suppose the length of an object or distance between two points measured by an observer at rest is l_o. This is called proper length.

If an object and an observer are in relative motion with speed V then the contracted length ‘I’ is given by

l= l_o / √1-(v²/c²)

since v/c<1 so l<l_o

Mass variation

According to the special theory of relativity mass of an object is a varying quantity. It depends upon the speed of the object.

The mass of an object measured at rest is m_o, this is called proper mass.

Now when the object moves with speed V the increased mass m of the object is given by

m= m_o /√1-(v²/c²)

As √1-(v²/c²) <1 so m>m_o

Thus increase in mass shows the increase in inertia, when the object has a high speed.

Limiting Speed

 If V approaches C it requires a larger and larger force to change the speed of object.

v=c then-v/c=1

v²/c² =1

If m= m_o /√1-(v²/c²) = m_o /√1-1= m_o/0

m=∞

As mass becomes infinite so infinite force is required to accelerate it. Since infinite force is not possible, so an object cannot be accelerated to the speed of light in free space.

Only light or other waves, which have no rest mass such as photon, can travel with the speed of light. In daily life we deal with extremely small speeds as compared to the speed of light.

The earth’s orbital speed is only 30 km/sec but speed

of light is 300,000km/sec that is why Newton’s laws of motion are valid in everyday situations.

In the case of atomic particles moving with velocities approaching the speed of light, the relativistic effects are not ignored. Thus experimental results cannot be explained without taking Einstein’s equation.

Energy-Mass Relation

According to the special theory of relativity mass and energy are different quantities but are interchangeable. The mass can be converted into energy and energy can be converted into mass.

The total energy E and mass m of an object are related by the relation

E=mc²

m= mass of object in motion which depends on the speed

c= speed of light

If m­_o is the rest mass of the object then rest mass energy is given by

E_o=m_oc²

Since mc² is greater than m_oc² the difference of energy due to motion is(mc²- m_oc²) which is called Kinetic energy of mass

K.E=(m-m_o)c²

if K.E= ΔE=change in energy

Then Δm=(m-m_o)=change in mass

ΔE= Δmc²

Δm= ΔE/c²

As c² is very large so change in mass requires a very large change in energy.

In daily life the energy changes are very small. So no significant change in mass of Object is observed. However, energy and mass changes in nuclear reactions are found to be exactly in accordance with the above equations.

Uses of the special theory of relativity

The results of the special theory of relativity are practically used in the modern system of navigation satellites called NAVSTAR.

The position and speed of an object anywhere on earth can be determined up to an accuracy of about 2cm/sec. However, if relativistic effects are not considered, speed could not be determined any closer than about 20cm/sec.

Using the special theory of relativity the position of an aircraft after an hour of its flight can be predicted with up to 50m accuracy. Without using the relativistic effects this approximation is about 760m.