Law of Conservation of Momentum

Law of conservation of momentum state that the total linear momentum of an isolated system remains constant in an isolated system. According to the Law of conservation of momentum, the quantity called momentum that characterizes motion, never changes in a collection of isolated objects. That is, the total momentum of the system remains constant.

Download the pdf notes of Law of Conservation of Momentum class 11 Physics

What is an Isolated system?

 A material system consisting of a body or a set of bodies whose constitutions can interact with one another but no external agency exerts a force on the system, then it is called an isolated system.

 A system on which no external force acts is called an isolated system.

 Example:

The molecules of a gas enclosed in a glass vessel at a constant temperature constitute an isolated system.

 The molecules can collide with one another because of their random motion but, being enclosed by glass vessels, no external agency can exert a force on them.

An isolated system is not possible in the real physical world But a set of objects whose internal mutual interaction is much greater than their interaction with other objects can be treated as an isolated system. Example:

 In a collision of two marble balls, the collision interaction is larger as compared with the frictional or gravitational forces.

Law of Conservation of Momentum

The total linear momentum of an isolated system remains constant.

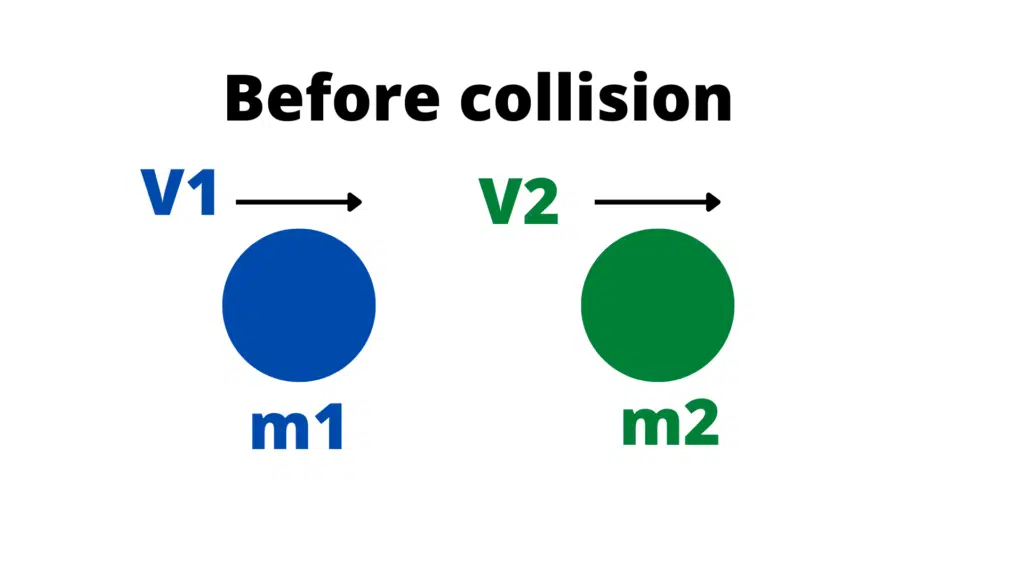
Consider an isolated system of two smooth, hard interacting balls of masses m₁ moving with velocity V₁ and m₂ moving with velocities V₂ respectively along the same straight line in the same direction.

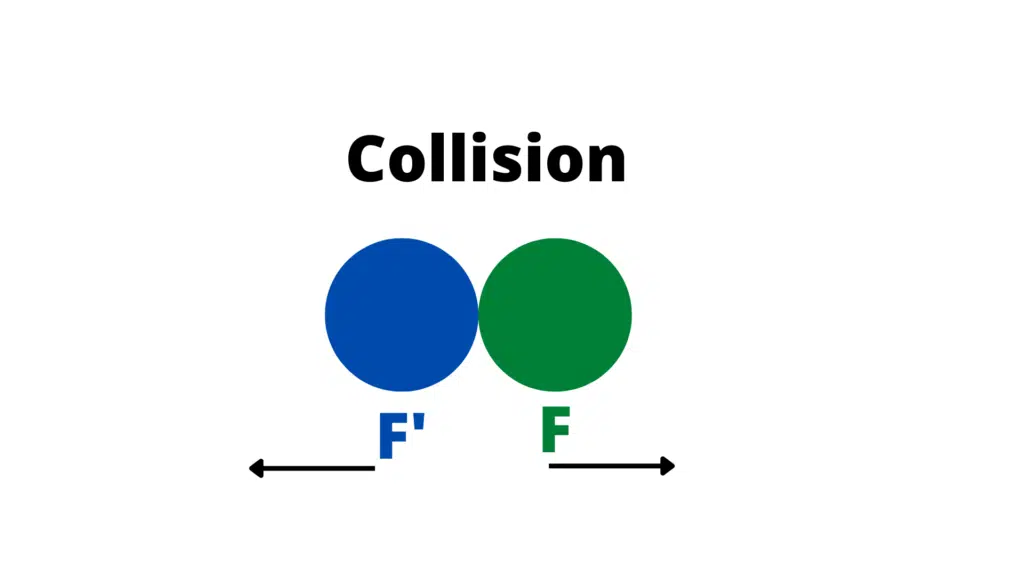
Both the balls collide

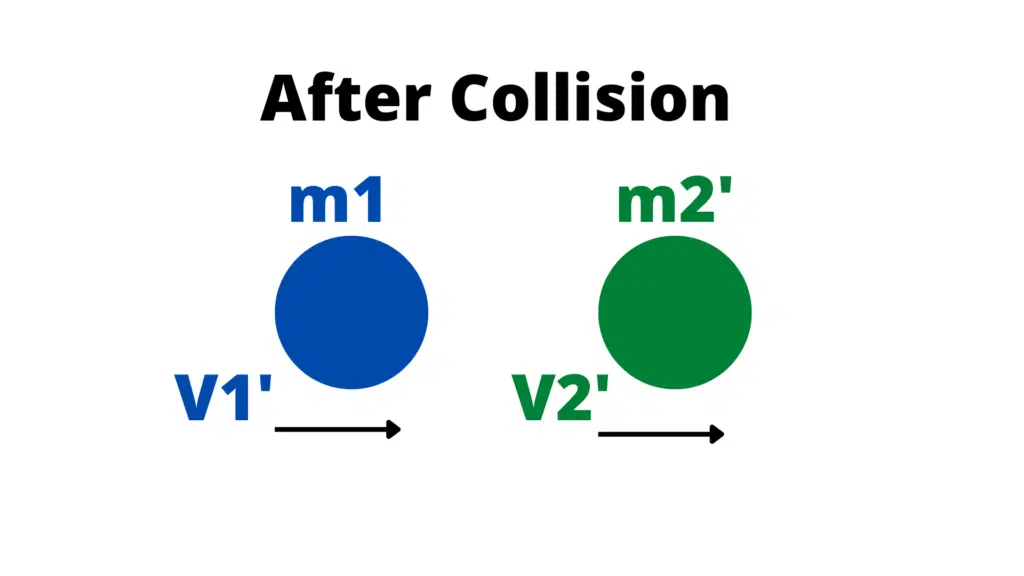
And after the collision, the ball of mass m₁ moves with the velocity v₁’, and m₂ moves with the velocity v2’ in the same direction.

Change in momentum for the ball of mass m₁

Fxt=m₁v₁’-m₁v₁

Change in momentum for the ball of mass m₂

F’xt=m₂v₂’-m₂v₂

(Fxt)+(F’xt)=( m₁v₁’-m₁v₁) + (m₂v₂’-m₂v₂)

(F+F’)xt = ( m₁v₁’-m₁v₁) + (m₂v₂’-m₂v₂)

The action force F is equal and opposite to the reaction force according to Newton’s third law

F’=-F

(F-F)xt=( m₁v₁’-m₁v₁) + (m₂v₂’-m₂v₂)

0 = ( m₁v₁’-m₁v₁) + (m₂v₂’-m₂v₂)

Re-arranging the equation, we get

 ( m₁v₁’+m₁v₂’) = (m₁v₁+m₂v₂)

Result:

The initial momentum of the system before a collision is equal to the final momentum of the system after the collision. The total change in momentum of an isolated system of two balls is zero.

For a group of objects:

If one of the objects within the group experiences a force. There must exist an equal but opposite reaction force on some other object in the same group.

As a result, the change in momentum of the group of objects as a whole is always zero So the law of conservation of momentum can be expressed as the total linear momentum of an isolated system remains constant.

Frequently Asked Questions-FAQs