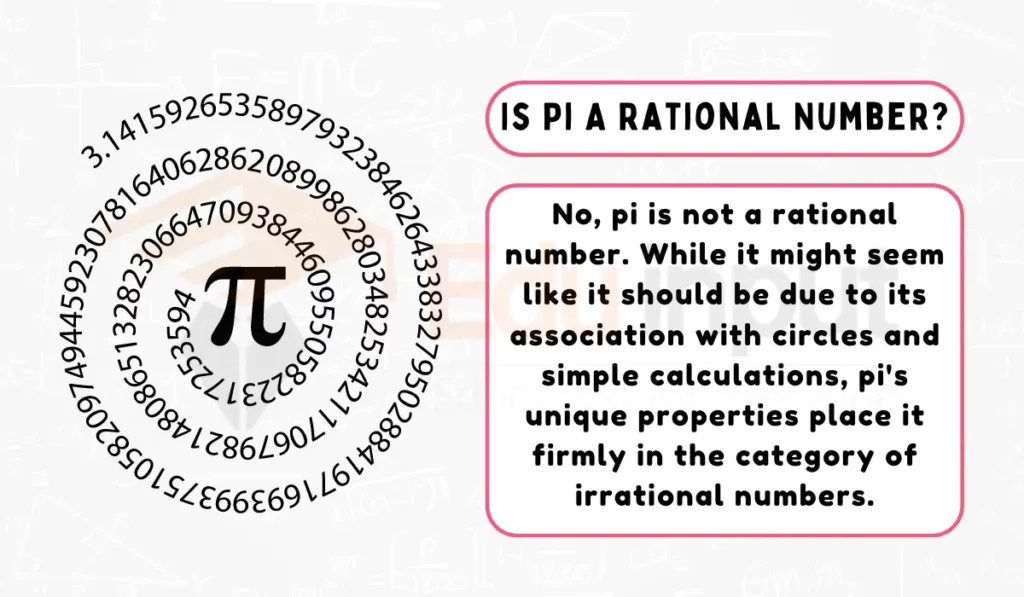
Is Pi A Rational Number?
written by Azhar EjazPi is rational or irrational? Pi (π) is an irrational number, meaning it cannot be expressed as a simple fraction (a ratio of two integers). Its decimal representation is non-terminating and non-repeating, which proves pi is a rational number or irrational number, specifically the latter.
In 1768, Swiss mathematician Johann Heinrich Lambert proved π is irrational, unlike rational numbers (e.g., 22/7), which represent a circle’s circumference-to-diameter ratio.
Read Other Examples of Rational Numbers
Why Is Pi An Irrational Number?
1. Non-Terminating and Non-Repeating Decimal
The decimal representation of π goes on forever without repeating (3.14159…). This violates the definition of a rational number, which must be expressible as a finite decimal or a repeating decimal fraction of two integers. Hence, pi is rational number or not—it is not.
2. Proof by Contradiction
We can assume the opposite-that – that π is rational – and arrive at a contradiction. This proves that our initial assumption must be false.
- If π were rational, it could be expressed as a fractions p/q (where p and q are integers).
- Squaring both sides of the equation, we get π² = p²/q².
- Multiplying both sides by q², we get π²q² = p².
- This means p² must be a multiple of q², which implies p itself must be a multiple of q (since squaring both sides cannot “create” new factors).
- This shows that both p and q have a common factor (q), violating the initial condition that the fraction p/q is in its simplest form.
This would imply that both p and q share a factor, violating the condition that p/q must be in simplest form. Therefore, π is rational or irrational? It’s irrational.
3. Connection to Geometric Shapes
π is fundamentally linked to circles and their properties, such as circumference and area, none of which result in rational values. These geometric concepts inherently involve irrational ratios that cannot be expressed as simple fractions.
These mathematical relationships confirm that pi is a rational number or not—it is not.
FAQs
Is -pi a rational number?
No, -pi is not a rational number. Rational numbers can be expressed as a fraction p/q, where p and q are integers and q is not zero. Since pi itself is irrational (cannot be expressed as such a fraction), its negative, -pi, also cannot be expressed as a simple fraction of two integers.
Is pi irrational?
Yes, pi (π) is an irrational number. This means it cannot be expressed as an exact fraction of two integers (p/q, where p and q are whole numbers and q ≠ 0). Its decimal representation goes on infinitely without repeating.
Is pi non-terminating?
Yes, pi (π) is non-terminating. Its decimal representation continues infinitely and does not end. This is a characteristic of irrational numbers.
Is pi a fraction?
No, pi (π) cannot be expressed as an exact fraction of two integers. While we use fractional approximations of pi (see below), it is fundamentally an irrational number and therefore not equal to any simple fraction.
What are rational approximations of pi?
Rational approximations of pi are fractions that are close to the value of pi but are not exactly equal to it. Some common rational approximations include:
22/7: A widely known approximation, approximately 3.142857.
355/113: A more accurate approximation, approximately 3.1415929.
3.14: A common decimal approximation that can be written as the fraction 314/100.
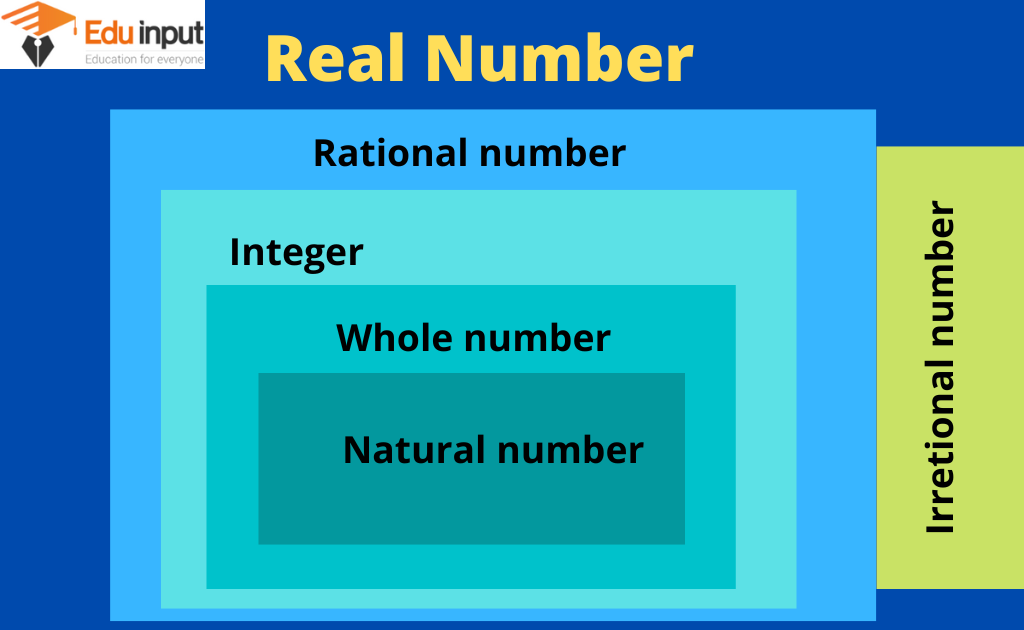
Leave a Reply