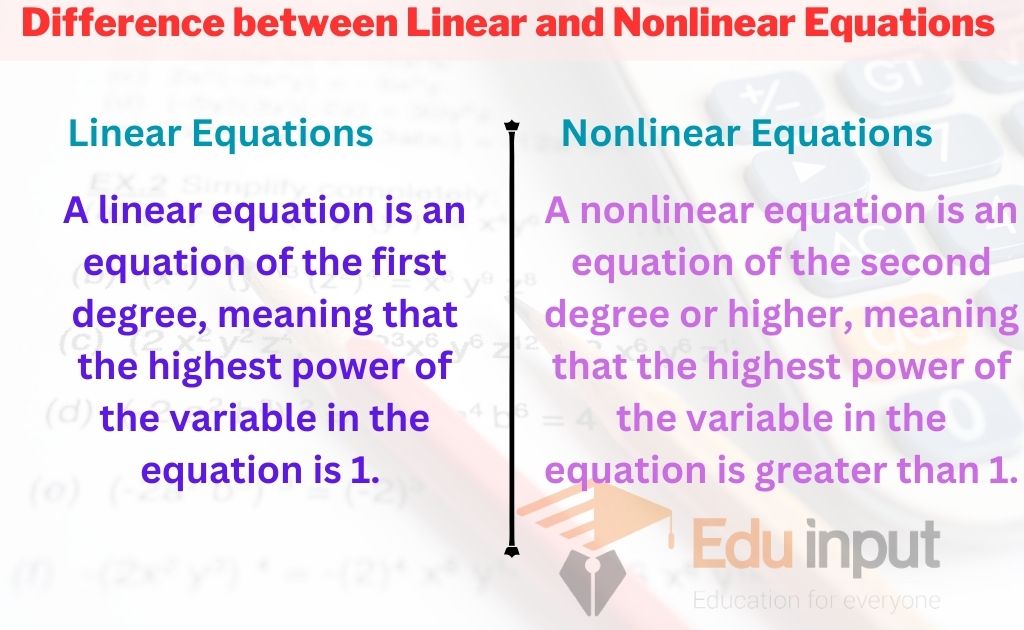
Difference Between Linear Function and Exponential Function

The main difference between linear and exponential functions is their rate of change. Linear functions have a constant rate, while exponential functions have a rate that continuously increases or decreases.

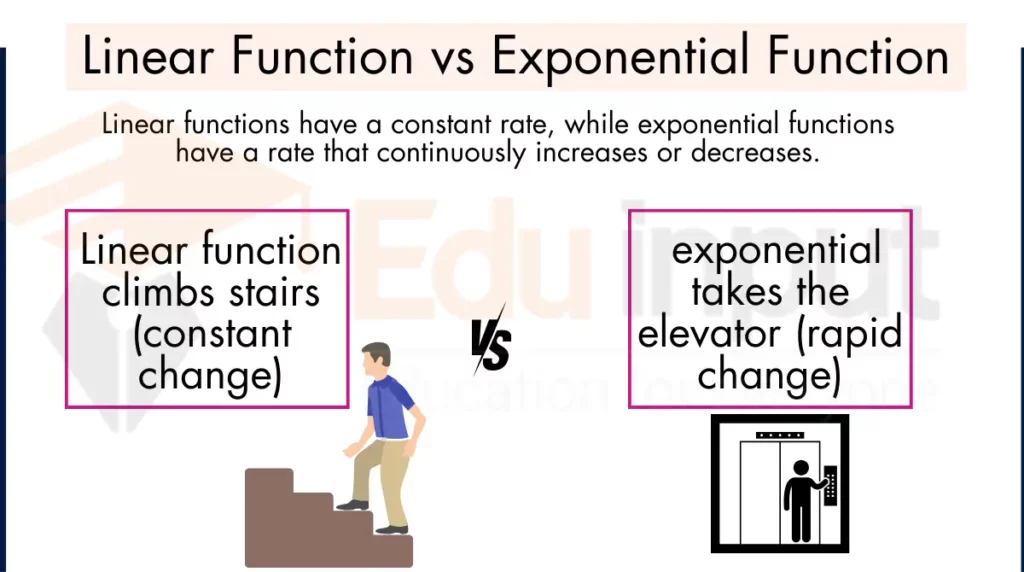
Comparative Analysis of Linear Function and Exponential Function

1: Rate of Change

Linear: In linear functions, the rate of change is constant. This means that for every equal increase in the input (x), the output (y) increases by the same amount. Imagine climbing a staircase – each step takes you the same distance higher.

Exponential: In exponential functions, the rate of change continuously increases or decreases. This means that for every equal increase in the input, the output increases (or decreases) by a larger multiplier. Picture bacteria multiplying or radioactive decay – the change gets faster or slower over time.

2: Visually

Linear function: Graph is a straight line with a constant slope.

Exponential function: Graph is a curve that either bends upwards or downwards, getting steeper or shallower as it progresses.

Examples

Linear: Distance traveled at a constant speed, cost of groceries with a fixed price per item.

Exponential: Population growth with constant birth rate, radioactive decay, and compound interest.

Linear Function vs Exponential Function

Here is a table summary showing Difference Between Linear Function and Exponential Function:

Property	Linear Function	Exponential Function
Definition	f(x) = mx + b	f(x) = a^x, where a is a positive constant and a ≠ 1
Rate of Change	Constant	Increases or decreases exponentially depending on the value of a
Graph	Straight line	Non-linear curve that approaches the x-axis asymptotically as x approaches negative infinity and increases/decreases without bound as x approaches positive/negative infinity depending on the value of a
Examples	y = 2x + 3, distance traveled at a constant speed	y = 2^x, population growth over time
Applications	Modeling linear relationships between variables, representing uniform motion	Modeling exponential growth or decay, representing compound interest, radioactive decay