Transverse Wave- Diagram, Properties, Formula, Applications
Transverse waves oscillate perpendicular to the direction of wave propagation. Examples of transverse waves include electromagnetic waves like radio and light, water surface ripples, and seismic secondary waves.
What is Transverse Wave?
The scientific definition of a transverse wave is a wave in which particles move at right angles to the direction of wave propagation. This property is what is meant by a transverse wave and can be observed in ripples on the water’s surface or vibrations in a string.
To define a transverse wave simply, it is a wave where the particle motion is perpendicular to the wave’s direction of travel. The transverse wave meaning in science is particularly evident when swinging a slinky vertically to create a horizontal wave.
The meaning of a transverse wave in physics lies in the perpendicular displacement of particles relative to the wave’s direction. For instance, swinging a slinky up and down produces a horizontal wave, a classic transverse wave explanation.
Transverse wave diagram
A sine or cosine curve can be used to depict a simple transverse wave. Any point’s distance from the axis is proportional to its amplitude on the curve, which is determined by the sine (or cosine) of an angle. A transverse wave diagram typically illustrates this relationship clearly.
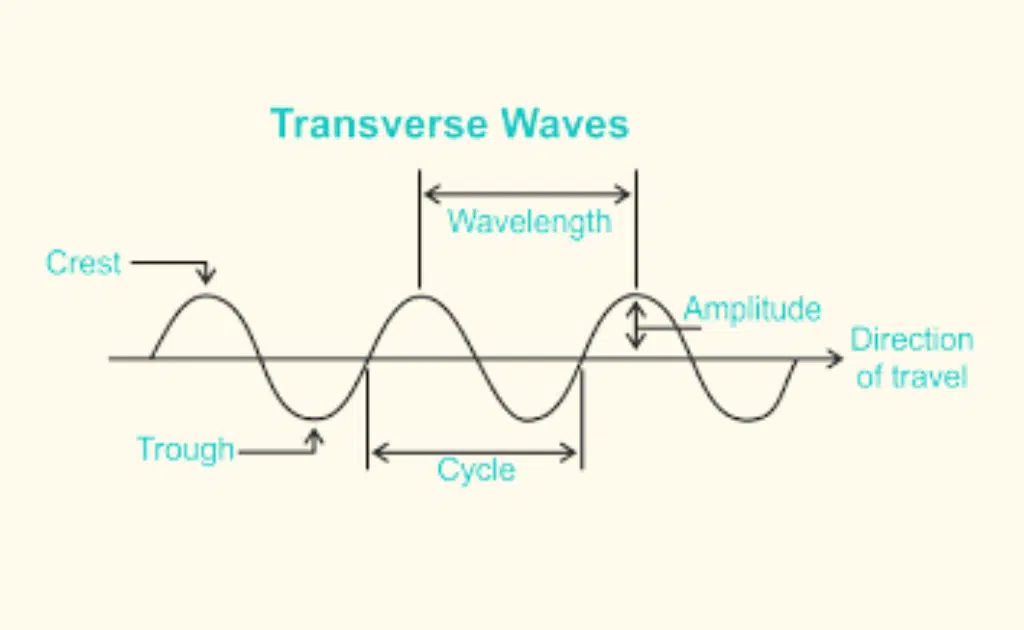
This labeled transverse wave highlights key features such as crests, troughs, wavelength, and amplitude. A drawing of a transverse wave often includes these details for better understanding.
A picture of a transverse wave can capture what a standing transverse wave looks like at different intervals of time, such as 1, 2, 3, 4, and 5.
These curves show what a standing transverse wave can be like at intervals of time (1, 2, 3, 4, and 5). The period of the wave motion is the length of time it takes for a point on the wave to fully oscillate around the axis, and the frequency is the number of oscillations that occur per second.
Characteristics of transverse waves
Here are some key features of transverse waves:
- Particle Motion: One of the important characteristics of transverse waves is that the particles of the medium oscillate or vibrate perpendicular to the direction of wave travel. This means that the displacement of particles occurs at right angles (transverse) to the wave’s direction.
- Crests and Troughs: Transverse waves have alternating high points called crests and low points called troughs. These are essential features of transverse waves, with crests corresponding to the maximum displacement of particles in the upward direction, and troughs representing the maximum displacement in the downward direction.
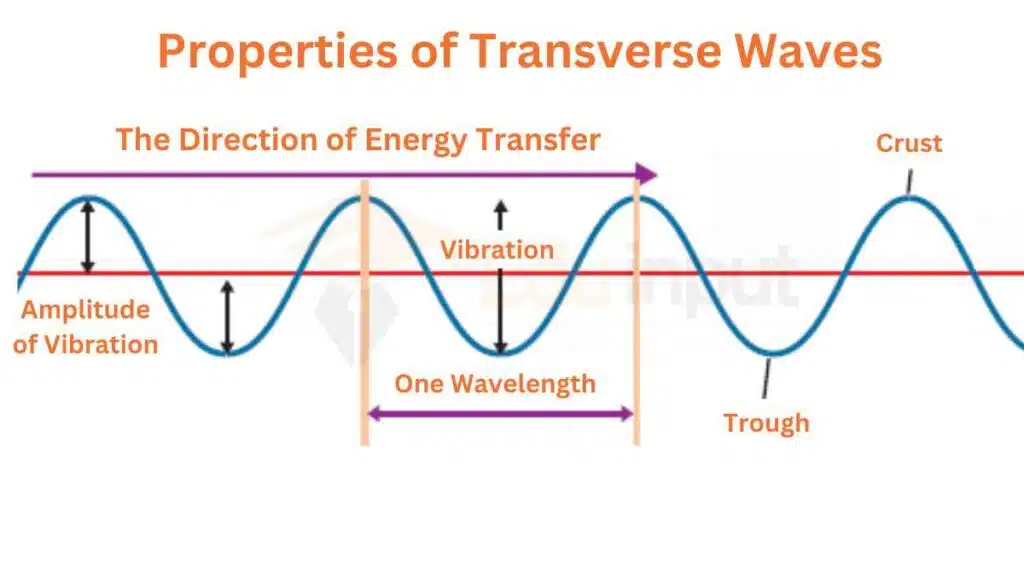
- Amplitude of Transverse Wave: It is one of the key transverse wave properties. The amplitude of a transverse wave refers to the maximum displacement of particles from their equilibrium position. It measures the magnitude or intensity of the wave and is typically measured from the midpoint (equilibrium position) to the crest or trough.
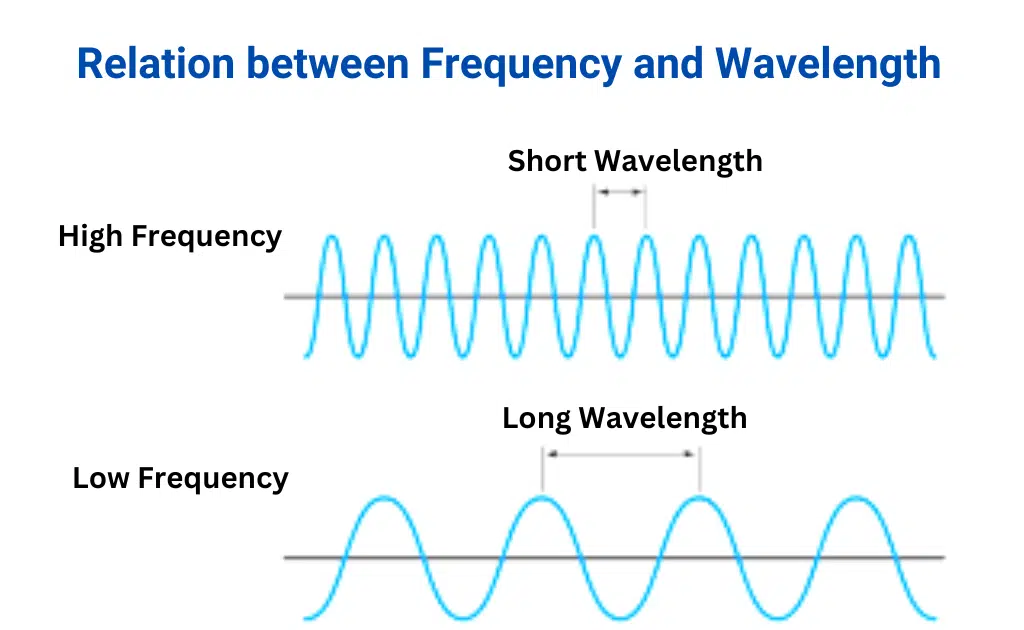
- Wavelength of Transverse Wave: The wavelength of a transverse wave, which is one of the fundamental transverse wave characteristics, is the distance between two consecutive crests or troughs. It measures the spatial extent of one complete cycle of the wave and is typically represented by the symbol λ (lambda).
- Frequency: The frequency of a transverse wave is the number of complete cycles or oscillations passing a given point in one second. It is measured in hertz (Hz) and is inversely proportional to the wavelength. The higher the frequency, the shorter the wavelength, and vice versa.
- Period: The period of a transverse wave is the time taken for one complete cycle or oscillation to occur. It is the reciprocal of the frequency and is typically represented by the symbol T. The unit of period is seconds (s).
- Energy Transfer: Transverse waves transfer energy from one point to another without permanently displacing the medium. As the wave propagates, the particles in the medium oscillate about their equilibrium position, but their overall displacement remains relatively small.
- Polarization: It is a unique characteristic of transverse waves. It refers to the alignment of the wave’s oscillations in a specific plane, perpendicular to the direction of propagation. All particles in the medium vibrate in the same plane during polarization.
Speed Of Transverse Waves
The speed of a transverse wave refers to the rate at which the wave propagates through a medium. It is a measure of how quickly the disturbance or energy travels from one point to another in the direction perpendicular to the wave’s motion. The speed of a transverse wave is determined by the properties of the medium through which it travels, such as the density, elasticity, and tension of the medium.
In general, the speed of a transverse wave can be calculated using the equation:
Speed (v) = wavelength (λ) × frequency (f)
Where:
- Speed is measured in meters per second (m/s).
- Wavelength is the distance between two consecutive crests or troughs of the wave, measured in meters (m).
- Frequency is the number of complete cycles or oscillations passing a given point in one second, measured in hertz (Hz).
It is important to note that the speed of a transverse wave is not dependent on its amplitude or intensity, but rather on the characteristics of the medium through which it propagates. Different mediums can have different speeds of transverse wave propagation, which can impact various phenomena and applications, ranging from the behavior of light and sound to the transmission of information in telecommunications.
Reflection of transverse waves
Reflection of transverse waves occurs when these waves encounter a boundary or an obstacle. During reflection:
- Angle of Incidence and Reflection: The angle at which the wave approaches the boundary (angle of incidence) is equal to the angle at which it reflects away (angle of reflection). This is stated as i=r, where i is the angle of incidence, and r is the angle of reflection.
- Behavior at Different Boundaries:
- At a fixed boundary, transverse waves reflect with a phase change of 180 degrees. This means the wave reflects in an inverted form.
- At a free boundary (like the free end of a rope), the wave reflects without a phase change, maintaining its original orientation.
- Practical Example: A good example to understand this is using a rope. If you send a wave pulse along a rope that is fixed at one end, you will see the pulse reflect back inverted. But if the end is free, the pulse comes back in the same orientation as it was sent.
Transeverse wave formulla
First, the basic transverse wave formula is often expressed as:
y(x,t)=Asin(kx−ωt+ϕ)
Where:
- y(x,t) is the displacement of the wave at point x and time t.
- A is the amplitude, the maximum displacement of the wave from its rest position.
- k is the wave number, related to the wavelength λ by k=2π/λ.
- ω is the angular frequency, related to the frequency f by ω=2πf.
- ϕ is the phase constant, which depends on where the wave starts.
To calculate the speed of the transverse wave, we use the speed of transverse wave formula:
v = fλ
where v is the speed, f is the frequency, and λ is the wavelength.
Example: Imagine we have a wave on a string. Let’s say the wave has a wavelength (λ) of 2 meters and a frequency (f) of 1 Hz (one cycle per second). The amplitude (A) of the wave is 0.5 meters.
Calculations:
- First, calculate the wave number (k): k=2π/λ=2π/2=π rad/m.
- Next, calculate the angular frequency (ω): ω=2πf=2π×1=2π rad/s.
So, our wave equation becomes:
y(x,t)=0.5sin(πx−2πt)
in this Equation:
- At any point x and any time t, this equation tells us the displacement y of the wave.
- When t=0, the equation simplifies to y(x,0)=0.5sin(πx). This is the shape of the wave at the very beginning.
- As time increases, the term −2πt changes the phase of the wave, making it look like it’s moving.
Activity
Let’s do a quick activity. Draw a sine wave on the board or on paper. Mark the amplitude and wavelength. Now, if you move this wave to the right every second, you’re demonstrating how the wave propagates over time, just like our equation describes.
This hands-on visualization helps in grasping the dynamic nature of transverse waves. It’s a fundamental concept, not just in physics, but also in understanding how things like sound, light, and even ocean waves behave. Remember, the beauty of physics lies in how these abstract equations translate into the real motion and behavior we observe in the world around us!
Applications of Transverse waves
Transverse waves have real-world applications. Engineers use knowledge of transverse waves to design buildings that can withstand earthquakes. Doctors use X-rays to look inside your body without surgery. Our entire modern communication system relies on these waves.
Below are some common applications of transverse wave…
Communication Technology
Transverse electromagnetic waves, such as radio waves, microwaves, and light waves, are fundamental to wireless communication technologies. These waves carry signals in various forms of communication devices, from radios to smartphones.
Medical Imaging
In medical fields, transverse waves play a crucial role. For instance, X-rays, a form of high-energy electromagnetic transverse wave, are used extensively for imaging internal structures of the body. Advanced techniques like MRI also rely on transverse wave properties for detailed internal imaging.
Seismology
Seismologists study transverse seismic waves (S-waves) to understand the Earth’s interior. These waves are crucial for earthquake analysis as they travel through the Earth’s crust and provide data on its composition and behavior during seismic events.
Optics
Transverse light waves are fundamental to the field of optics. Understanding the behavior of light as a transverse wave allows for the design of lenses, mirrors, and optical fibers, which are essential in industries ranging from telecommunications to medical equipment.
Material Science
The study of transverse waves in materials helps in understanding the material properties like stress and strain relationships, helping in the development of stronger and more resilient materials.
Remote Sensing
Transverse waves, especially in the electromagnetic spectrum, are used in remote sensing technologies to collect data about the Earth’s surface. This is crucial for environmental monitoring, weather forecasting, and geographical mapping.
Important Questions Related to Transverse Wave
These are some questions related to transverse waves.
Real-life examples of transverse waves
Does wave interference occur with transverse waves?
Can transverse waves be Polarized?
Why is Electromagnetic Wave a Transverse Wave?
Does light have transverse waves?
Difference between Longitudinal and Transverse Wave
Related FAQs
What is a transverse wave in physics?
A transverse wave is a motion in which all points on a wave oscillate along paths at right angles to the direction of the wave’s advance. Surface ripples in water, seismic S (secondary) waves, and electromagnetic waves are examples of transverse waves. transverse wave.
What are 3 examples of a transverse wave?
ripples on the surface of the water.
vibrations in a guitar string.
a Mexican wave in a sports stadium.
What is a transverse wave and how does it move?
Transverse waves are waves that move particles in a direction perpendicular to the direction of a wave. An example of transverse waves is ocean waves in which water moves up and down, but does not move forward with the wave.
Why are waves called transverse?
In physics, a transverse wave is a wave whose oscillations are perpendicular to the direction of the wave’s advance. This is in contrast to a longitudinal wave which travels in the direction of its oscillations. Water waves are an example of a transverse wave.
How are transverse waves formed?
A transverse wave is a wave that vibrates perpendicular to the direction of the wave or path of propagation. Transverse waves are created when a motion perpendicular to the direction of propagation of the wave is induced in the molecules that make up the medium.
What does a transverse wave look like?
A transverse wave appears as a series of crests and troughs, where the particles of the medium vibrate perpendicular to the direction of wave propagation.
Why is a Mexican wave transverse?
A Mexican wave is considered a transverse wave because the motion of the wave is perpendicular to the direction of its propagation.
Transverse wave example in real life?
Here are three examples of transverse waves in real life:
Light Waves
Water Ripples
Vibrating Guitar String

 written by
written by