What is Carnot Engine? | Carnot’s theorem

A Carnot engine is a theoretical engine that works on the basis of a Carnot cycle. The Carnot engine is the most efficient engine so far.

Carnot engine

Sadi Carnot, a French physicist, and military engineer described an ideal engine using only isothermal process and adiabatic processes. He showed that a heat engine operating in an ideal reversible cycle between two heat reservoirs at different temperatures would be the most efficient engine.

Carnot cycle

It consists of the following four steps:

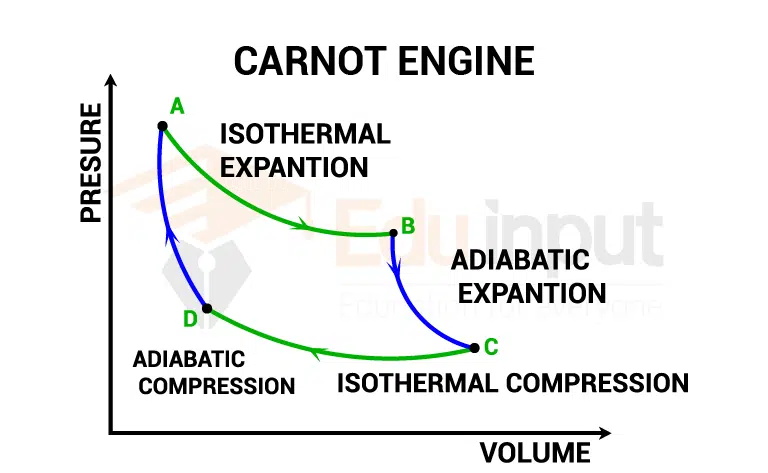
Isothermal expansion

The process a → b is an isothermal expansion at temperature, T₁ in which the gas is placed in thermal contact with a hot reservoir at temper T₁. During this process, the gas absorbs energy Q₁, from the reservoir and does work W_ab in raising the piston.

Adiabatic expansion

In the process b → c the base of the cylinder is replaced by a thermally non-conducting wall and the gas expands adiabatically; That is, no energy enters or leaves the system by heat. During the process, the temperature falls from T₁, to T₂ and the gas does work W_bc in raising the piston.

Isothermal compression

In the process c → d, the gas is placed in thermal contact with a cold reservoir at temperature T₂, and is compressed isothermally at temperature T₂. During this time, the gas expels energy Q₂ to the reservoir, and the work done on the gas is W_cd.

Adiabatic compression

In the final process d → a, the base of the cylinder is again replaced by a thermally non-conducting wall and the gas is compressed adiabatically. The temperature of the gas increases to T₁, and the work done on the gas is W_da.

Carnot engine Efficiency

As the working substance returns to its initial state, there is no change in its internal energy i.e. ΔU=0. The net work done during one cycle equals the area enclosed by the path abcda of the PV diagram. It can also be estimated from net heat ΔQ absorbed in one cycle.

ΔQ=Q₁-Q₂

ΔQ=ΔU+ΔW

ΔW=Q₁-Q₂

η =output (Work)/input (Energy)

η =Q₁-Q₂/Q₁

η =1 – Q₂/Q₁

The heat energies Q₁ and Q₂ are always proportional to the Kelvin temperatures T₂ and T₁ respectively and hence,

Q₂/Q₁=T₂/T₁

η =1 – T₂/T₁

The efficiency is usually taken in percentage, in that case,

η = [1 –T₂/T]

Thus the efficiency depends upon the Temperature of the hot reservoir and the Temperature of the cold reservoir. And does not depend upon the Nature of the working substance.

The larger the temperature difference between the two reservoirs, the greater will be the efficiency. The efficiency of the Carnot engine cannot be 100 % unless the cold reserve is at absolute zero temperature (T₂: 0 K), which is impossible. Thus the efficiency of the Carnot engine is always less than one or 100%.

Carnot’s theorem

As the practical heat engines are not perfectly reversible due to energy losses, Carnot stated a theorem:

No heat engine can be more efficient than a Carnot engine operating between the same two temperatures.

All Carnot engines operating between the same two temperatures have the same efficiency, irrespective of the nature of the working substance.

Practically the cold reservoir is nearly at room temperature, so the efficiency is increased by increasing the temperature of the hot reservoir. All real heat engines like petrol engines are less efficient than Carnot engines due to friction and other heat losses.