What is Motional emf | Faraday Law

The emf induced by the linear motion of a conductor across a magnetic field is called Motional Emf. Faraday’s law state that The average emf induced in a conducting coil of N loops is equal to the negative of the rate at which the magnetic flux through the coil is changing with time.

Motional emf

Consider a conducting rod of length L placed on two parallel metal rays separated by a distance of L. A galvanometer is connected between the ends c and d of the rails. It forms a complete conducting loop abcd. A uniform magnetic field B is applied to the paper.

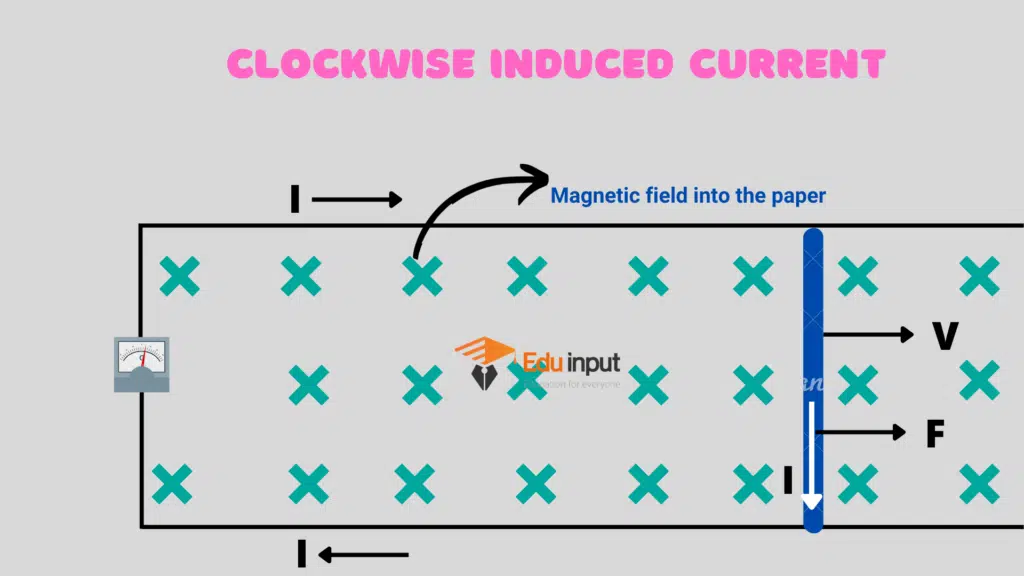
When the rod is at rest galvanometer shows no deflection. If the rod is pulled to the right with constant velocity V the galvanometer indicates the current flowing through the loop.Thus the moving rod is acting as a source of emf;

E=v _b– v _a=∆v

When the rod moves a change q within the rod also moves with the same velocity v in the magnetic field B and experiences a force.

F=q (V*B)

having magnitude.

F=q V B sin

F=q V B

According to the right-hand rule, the direction of F is from a to b. Hence a uniform magnetic field E is induced along the rod having magnitude.

F_e= F_b

q E = q V B

E=v B

The direction of electric intensity is also from a to b. As the electric intensity is equal to the negative potential gradient

E=-∆V/L

E=-ԑ/L

v B=- ԑ/L

ԑ=-vBL

This is the magnitude of motional emf. If θ is the angle between v and B then

ԑ=-v BL sin θ

Factors increasing motional emf:

Motional emf can be increased by;

• Increasing the speed of the rod
• Using a stronger magnetic field

When v=0ԑ =0 no motional emf is developed in a stationary rod.

Due to induced EMF positive charge will flow along the path abcda therefore the induced current is anti-clockwise.

FARADAYS LAW

The average emf induced in a conducting coil of N loops is equal to the negative of the rate at which the magnetic flux through the coil is changing with time.

ԑ=-N Δ ϕ/ Δt

Explanation:

A conducting rod L move from position 1 to position 2 in time Δt and covers a distance

Δx=x₂-x₁

The velocity of the rod is given by

v= Δx/Δt

The emotional emf induced in the rod perpendicular to the magnetic field is

ԑ= -vBL

ԑ= -(Δx/Δt)BL

When the rod covers the distance Δx increase in the area of the loop is

ΔA= Δx .L

And the increase in the flux through the loop is

Δ ϕ =B ΔA

Δ ϕ =B Δx L

Becomes,

                    ԑ= – Δ ϕ/ Δt

For a coil of N, the induced emf will become N time so,

ԑ= -N(Δ ϕ / Δt)

This is called the faradays law of electromagnetic induction. The –ve sign show that the direction of induced emf is such that it opposes the change in flux.