Simple Pendulum-Definition and Motion of Simple Pendulum

A simple pendulum consists of a small heavy mass suspended by a light extensible string fixed at its upper end.

Simple Pendulum

Consider a simple pendulum of mass “m” suspended by a light string of length l which is fixed to rigid support at its upper end. When the bob is displaced from its mean position to position B at a distance x and released. The pendulum starts executing simple harmonic motion under the action of its weight, which is acting vertically downward.

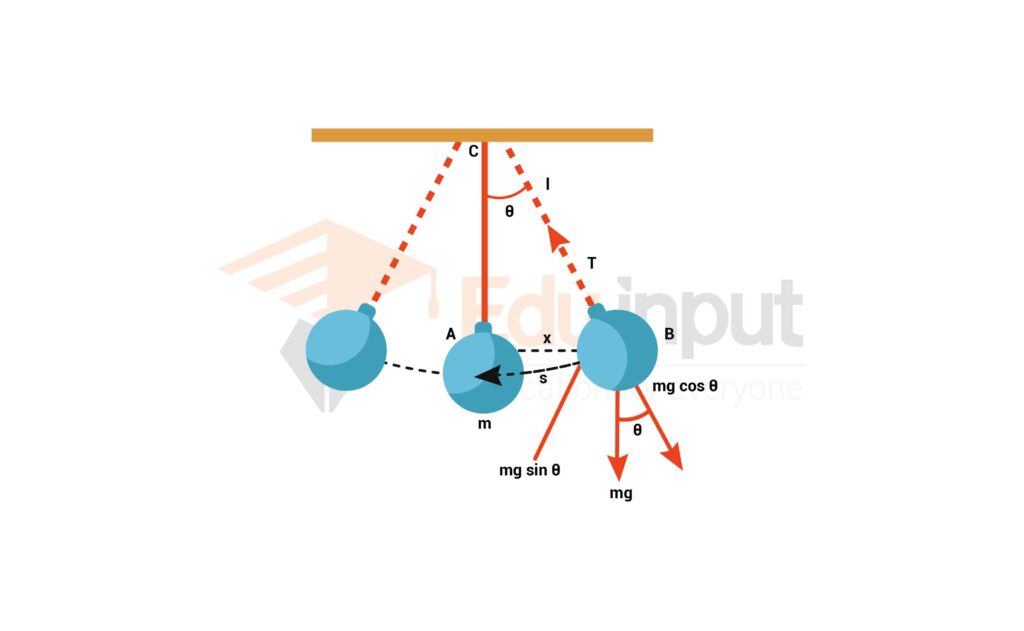
Forces acting on a simple pendulum

Now to find the force responsible for the motion of the pendulum, we must find all the forces that are acting on the pendulum.

Tension on Simple Pendulum

Tension T in the string acting vertically upward along the length of the string.

 The motion of a simple pendulum

 Weight mg acting vertically downward, it is resolved into two parts mgCosθ and mgSinθ.

The component of weight mg cosθ is acting downward opposite to the tension in the string

So these two forces balance each other ie- mgcosθ mg sinθ is acting perpendicular to the string and along the direction of motion of the pendulum, So this component of force is responsible for the motion of the pendulum, so

F=mgsinθ

Since this force is directed towards the mean position, it acts as restoring force, so we assign a negative sign to it

F=-mg sinθ

According to Newton’s Law of Motion

F=ma

ma=-mg sinθ

a = – g sin θ

Since the angle formed by the pendulum is very small, so for small angle sin θ≈ θ

The above equation can be written as

a = – g θ

From the figure it is clear that when θ is very small then Arc AB = 0B=x

θ= (OB)/l = x/l

a=- g x/l

a=-( g/l)x

for a particular place g is constant and for a given pendulum ‘l’ is also a constant. Therefore g/l=constant t

a=-constant x

a ∝ -x

This shows that the acceleration is proportional to the displacement and is directed towards the mean position.

Hence the motion of the simple pendulum is simple harmonic motion.

The time period of Simple Pendulum

The time period of the simple pendulum can be found by using the equation

T = (2π)/ ω

To find ω we have

a=-(g/l) x

Also, we have

a= -ω² x

-ω ²x= – (g/l) x

ω²=g/l

w=√g/l

T=2 π/√g/l

T=2 π√l/g

From this equation, it is clear that the time period of a simple pendulum is proportional to the length I and is independent of the mass of the pendulum.

The factor g the denominator indicates that if the value of g decreases the T will also increase that is why the time period of a pendulum is not the same at sea level or at Mount Everest.