Work Done by a Constant Force

The work done by a constant force is defined as W = F · d. This is the dot product of the force applied to the object and the distance covered in the direction of the force. To understand the concept of work done by a constant force we have to know about work done.

Download the pdf notes of Work Done by a Constant Force

What is work done?

“When a force acts on a body and displaces it through some distance, then work is said to be done.”

Physics measures the transfer of energy that occurs when an object travels a distance by an external force that acts at least partially in the direction of displacement.

If the force is constant, work can be calculated by multiplying the length of the path by the component of the force acting along the path.

Mathematically expressing this concept, work W is equal to force f multiplied by distance d. That is,

 W = fd

If the force is applied at an angle θ to the displacement, the work done is

W = fdcos θ

Example:

A man is applying a force f on a box and it covers some distance d then work is done by the man.

What is the unit of work?

System International unit of work is Nm known as joule (J).

If the 1 N force is applied on a body and the body covers 1m displacement in direction of force then work done is 1joule.

What is the dimension of work?

The dimension of work is [ML²T²]

 Is work a scalar quantity?

Since work is the dot product of two vectors force and displacement. Hence the work is a scalar quantity.

When the work done is positive?

If the angle between force and displacement would be greater than 90 (θ <90°) work is done and is said to be positive work.

When the work done is negative?

If the angle between force and displacement would be less than 90 (θ >90°) then work done is said to be negative work.

When the work done is zero?

If θ =90° then the work done is considered zero. Because the value of Cos 90° is zero.

so work done will also be zero when the force and displacement are perpendicular to each other.

Work Done by a Constant Force

To calculate the work done by a constant force we have to know about constant force.

What is constant force?

“If the magnitude and direction of a force remain the same, then the force is to be a Constant Force.”

Consider a constant force ‘F’ which acts on a body at an angle θ and displaces it from position ‘A’ to ‘B’ through a displacement ‘d’.

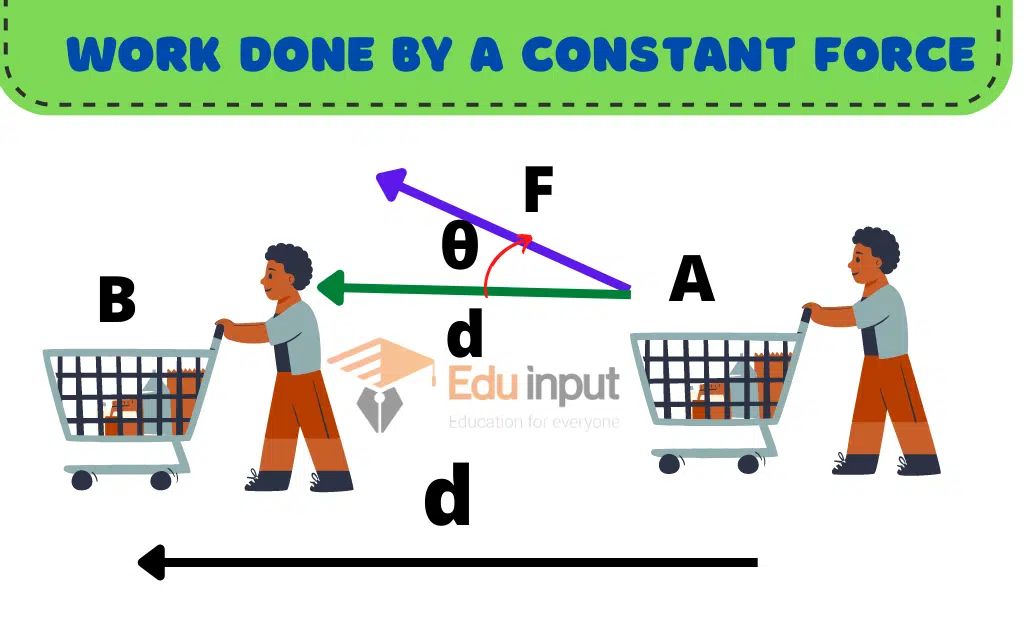
Thus the work can also be defined as:

“The product of the magnitudes of the displacement and the component of the force in the direction of the displacement is called Work.”

then the work done is given by,

W= F.d

W = (F Cos θ) d = Fd Cos θ

The product of magnitudes of force and component of displacement in the direction of the force is called work.

W=F.d

F (d Cos θ) =Fd Cos θ

What is a graphical method to find work?

Consider a graph between force and distance.

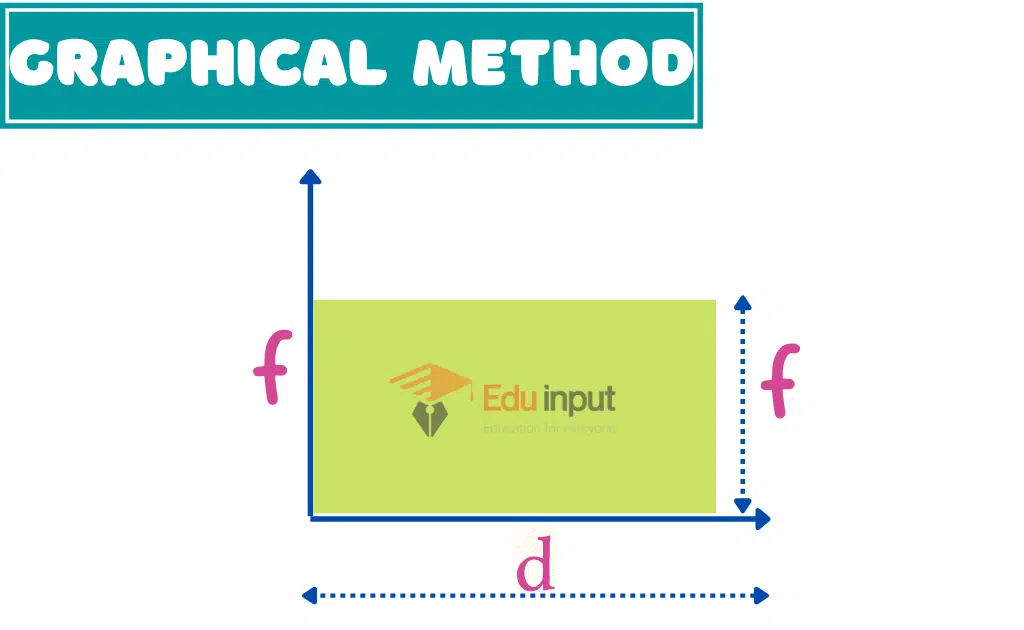
 The distance is normally taken along the x-axis and the force along the y-axis. Since the force is constant so the graph will be a horizontal straight line

 The constant force (Newton) and the displacement d (meter) is in the same direction, then the work done is Fd (joule).

 Also, the shaded area is also Fd. Hence the area under a force-displacement curve is equal to the work done by the force.

 If the force F is not in the direction of displacement, the graph is plotted between FCos θ and d