Collinear Points-Definition, Formula, And Methods To Find Collinear Points

Collinear points are three or more points that lie on the same straight line. The word ‘Collinear’ is a compound word made up of two words: ‘co’, meaning togetherness, and ‘linear’, meaning a line. Collinear points might exist on different planes, but not on different lines. Therefore, as the points are collinear, it is known as collinearity. Non-collinear points are points that do not lie in a straight line.

Collinear Points Definition

The term “collinear” is a combination of two Latin words: “col” + “linear”. “Col” means together and “linear” means line. So, collinear points are points that lie on the same line.

You can see many examples of collinearity in real life, such as a group of students standing in a straight line, or a bunch of apples kept in a row next to each other.

In geometry, two or more points are said to be collinear if they lie on the same line. So the collinear points are the set of points that lie on a single straight line.

Collinear Points in Math

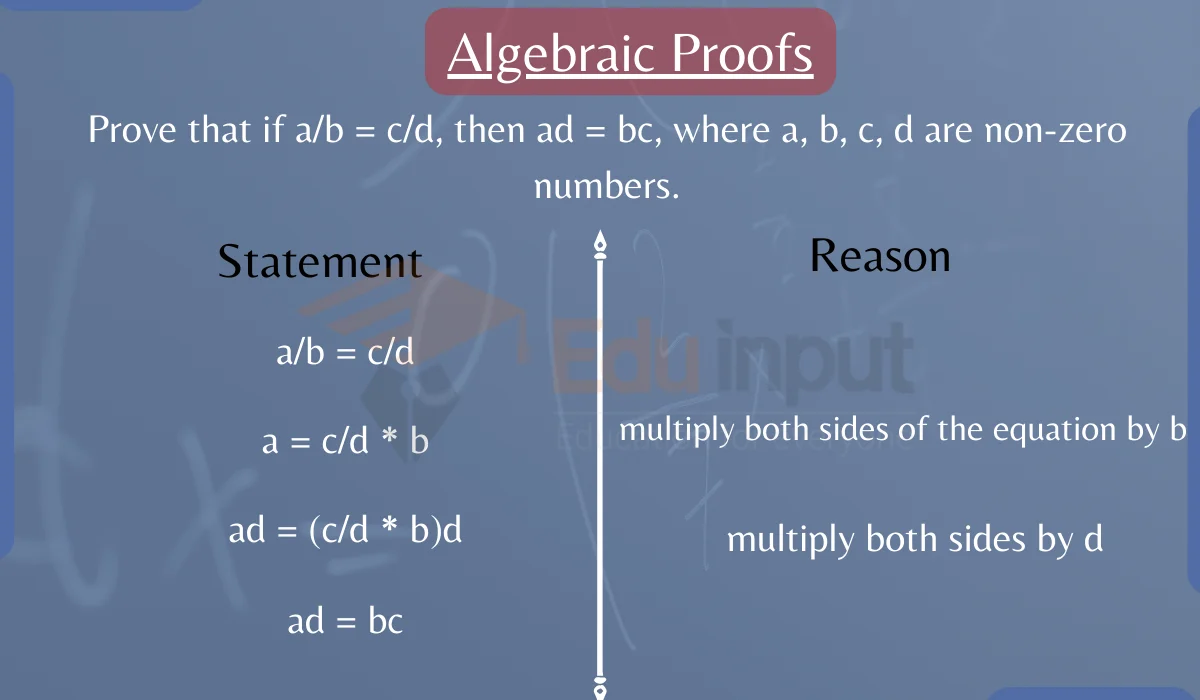
In Mathematics, Collinear points are points that lie on the same straight line. As you can see in the figure below, points P, Q, and R are collinear.

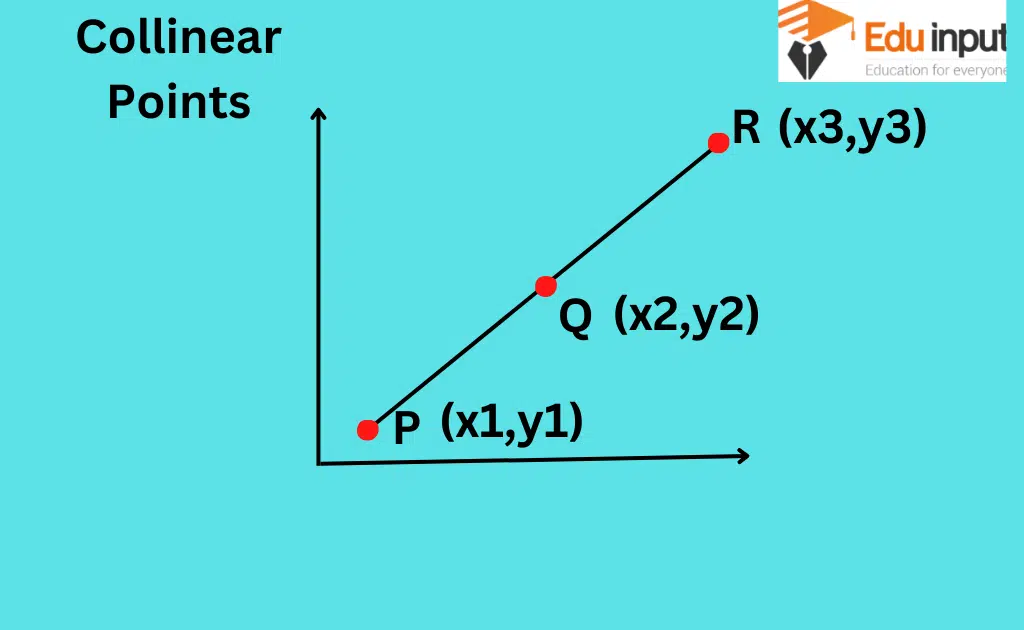
Non-Collinear Points

A non-collinear point is a point that doesn’t lie in a straight line. The example of non-collinear points is given below:

Collinear Point’s Formula

The collinear point’s formula is a mathematical way of determining whether three points are collinear, or in a straight line. There are various methods of calculating this – some more complex than others – but the collinear point’s formula is a reliable way to check whether three points are indeed in a straight line. There are three methods to find the collinear points. They are:

• Area of triangle
• Distance Formula
• Slope Formula

Area of triangle Formula

This method relies on the fact that three collinear points cannot form a triangle. This means that if any three points are collinear, they cannot form a triangle. To check the points of the triangle for collinearity, we use them in the formula for the area of a triangle. If the area is equal to 0, then those points will be considered to be collinear. In other words, a triangle formed by three collinear points will have no area since it would just be a line joining the three points. The formula for the area of a triangle that is used to check the collinearity of points is as follows:

The area of the triangle with the given points (vertices) A(x1, y1), B(x2, y2), and C(x3, y3) is:

A=1/2|(x₁(y₂−y₃)+x₂(y₃−y₁)+x₃(y₁−y₂))|=0

Distance Formula

The three points are collinear if the sum of the distance between the first and second points plus the distance between the second and third points is equal to the distance between the first and third points.

For example, if we take A, B, and C as any three collinear points, then

Distance from A to B + Distance from B to C = Distance from A to C

So, A, B, and C are collinear points.

Now, by the distance formula we know, the distance between two points (x₁, y₁) and (x₂, y₂) is given by;

d=√(x₂−x₁)²+(y₂−y₁)²

Slope Formula

We can use the slope formula to find out whether or not the three points are collinear. If the three points have equal slopes, then they are collinear.

For example, if we have three points X, Y, and Z, they will be collinear only if the slope of line XY is equal to the slope of line YZ is equal to the slope of line XZ. To calculate the slope of the line joining two points, we use the slope formula.

The slope of the line joining points P(x1, y1) and Q(x2, y2) is:

m=y₂−y₁/x₂−x₁

Summary

Three points are said to be collinear if they lie on the same straight line. This property is known as collinearity. Collinear points can exist on different planes.

Frequently Asked Question-FAQs