10 Examples of Statistical Mechanics

Statistical mechanics is a branch of physics that applies statistical methods to understand the behavior of a large number of particles in a system. It provides a bridge between the microscopic world of atoms and molecules and the macroscopic properties of matter.

Examples of Statistical Mechanics

Here are ten examples of statistical mechanics in action.

1. Ideal Gas Law

Statistical mechanics explains the behavior of an ideal gas in terms of the average properties of gas particles, such as their velocity distribution, leading to the ideal gas law, PV = nRT.

2. Heat Capacity of Solids

Statistical mechanics can predict the heat capacity of solids, such as metals and crystals, by considering the vibrational modes of atoms in the lattice structure.

3. Fermi-Dirac and Bose-Einstein Statistics

Statistical mechanics is used to derive Fermi-Dirac statistics for fermions (e.g., electrons) and Bose-Einstein statistics for bosons (e.g., photons), which describe their behavior at low temperatures.

4. Partition Function

The partition function in statistical mechanics summarizes the thermodynamic properties of a system. It’s used to calculate quantities like the Helmholtz free energy, entropy, and internal energy.

5. Maxwell-Boltzmann Distribution

Statistical mechanics provides the Maxwell-Boltzmann distribution, which describes the velocity distribution of particles in a gas and is crucial in understanding diffusion and transport phenomena.

6. Phase Transitions

Statistical mechanics explains phase transitions in matter, such as the transition from a gas to a liquid or a liquid to a solid, by analyzing the behavior of particles and their interactions.

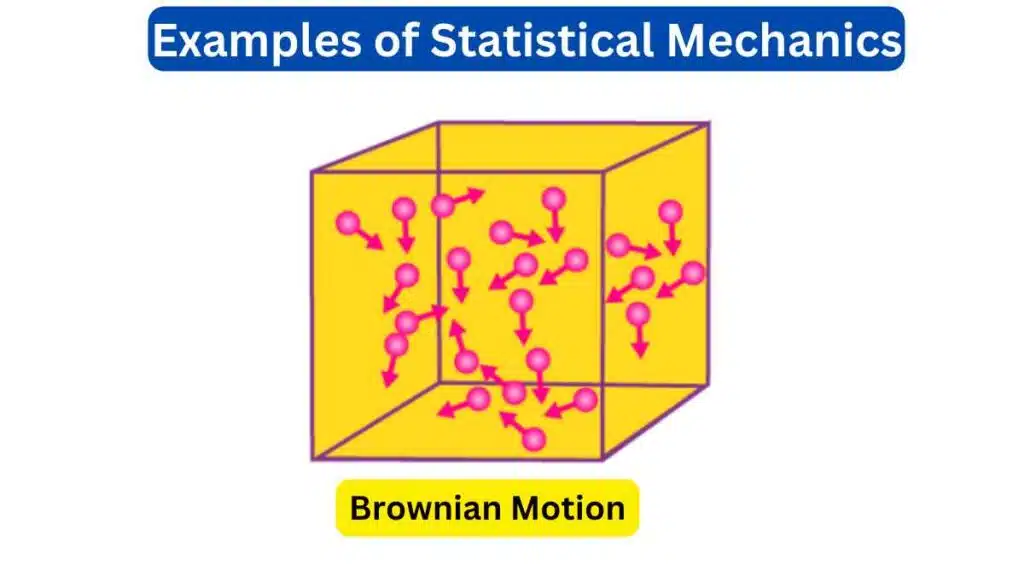
7. Brownian Motion

Statistical mechanics explains Brownian motion, the random motion of particles in a fluid, by considering the collision of particles with solvent molecules.

8. Equipartition Theorem

The equipartition theorem states that each degree of freedom in a system contributes to its energy. Statistical mechanics helps determine the average energy associated with each degree of freedom.

9. Entropy and Thermodynamics

Statistical mechanics provides a microscopic foundation for the concept of entropy, helping to explain the second law of thermodynamics, which states that entropy tends to increase in isolated systems.

10. Fluctuations and Noise

Statistical mechanics accounts for fluctuations and noise in physical systems. This is important in various fields, including electronics, where noise can affect device performance.

These examples illustrate the broad scope of statistical mechanics and its relevance in understanding the behavior of matter at both the microscopic and macroscopic levels. It is a foundational theory that underlies many concepts in physics and chemistry.