Entropy | The Second Law of Thermodynamics in Terms of Entropy
The entropy is a measure of the amount of energy that is unavailable to do the work of an object. Entropy tells us the amount of disorder in a closed system.
Entropy
The degree of disorderliness is called Statistical Entropy. The unavailability of heat energy is called Thermodynamic Entropy.
Temperature and internal energy are both state variables; that is, they can be used to describe the thermodynamic state of a system. Another state variable is entropy S. In this section we define entropy on a macroscopic scale as the German physicist Rudolf Clausius (1822-1888) first expressed it in 1865. Consider a reversible process between two equilibrium states. it Q is the energy absorbed or expelled by the system during some small interval of the path, the change in entropy ΔS is given by
ΔS=ΔQ/T
Entropy Unit:
The unit of entropy is j/K
Entropy ΔS is +ve if heat enters the system and -ve if heat leaves the system.
Law of increase of entropy
The entropy of a thermodynamic system either remains constant (during the reversible process) or increases (during the Irreversible process).
Consider two reservoirs at temperatures T1, and T2 where T1T2 Suppose heat Q flows from the reservoir at high-temperature T1 to the other at low-temperature T2 through a conducting rod.
The entropy of the reservoir at temperature T1=Q/T1
The entropy of the reservoir at temperature T2=Q/T2
As T1>T2
Hence the net change in entropy=ΔQ/T2-ΔQ/T1is positive.
Result:
In all natural processes, there is always a net increase in entropy.
This is another statement of the second law of thermodynamics.
The second law of thermodynamics in terms of entropy:
If a system undergoes a natural process, it will go in a direction that causes the entropy of the system plus the environment to increase.
Measure of disorders
Entropy is also defined as a measure of the disorder in the system.”
Examples:
Hot and cold body:
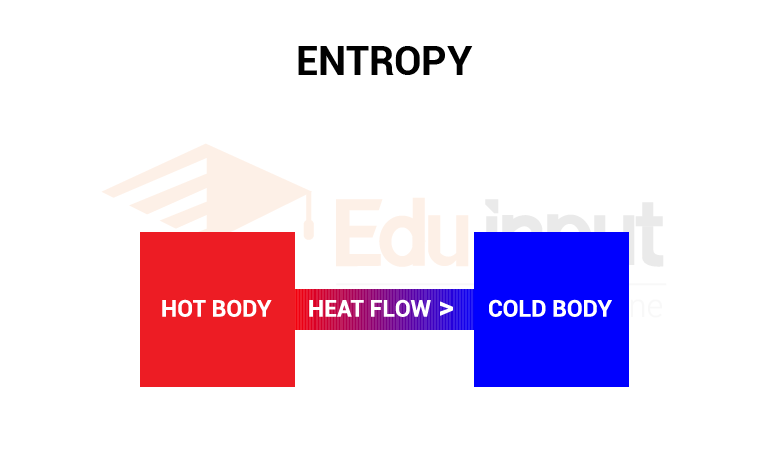
The disorder of the system increases when heat flows from a hot to a cold body. This is because the molecules were initially sorted out in order of hot and cold regions.
The order was lost when the system came to thermal equilibrium.
The motion of molecules:
Free expansion of gas increases the disorder because the molecules have greater randomness of position after expansion than before.
In both the above examples the entropy is said to be increased. For reversible processes the entropy is constant. i.e. change in entropy is zero. For irreversible processes entropy is positive.
Degradation of energy:
Every time when entropy increases then there is less chance of converting heat into work. Suppose we increase the entropy of a system by mixing the hot and cold waters. Now the warm water which we get after mixing cannot be separated into hot and cold water again. Although no energy is lost now the energy is not available for conversion into work.
Therefore increase in entropy mean the degradation of energy. The energy in a sense is degraded going from more orderly from to less orderly and ending up as thermal energy.
Heat death:
The entropy of an isolated system and of the universe as a whole can only increase. Even if the temperature of some systems decreases then there must be a net increase in the entropy of some other system. Applied to the universe as a whole this principle suggests that eventually, all temperature in space becomes uniform resulting in the so-called heat death of the universe.
Thus entropy increases as it changes to water. The increase in entropy in this case is a measure of the increase in the disorder of water molecule that changes from solid to a liquid state.




Leave a Reply