Fundamental Theorem of Arithmetic
Factorization is the process of expressing a number as the product of its factors. The Fundamental Theorem of Arithmetic describes the unique prime factorization of integers. This theorem is a key concept in number theory and has several important applications in mathematics.
In this article, we will discuss the fundamental theorem of arithmetic.
Prime and Composite Numbers
A prime number is an integer greater than 1 that has no positive divisors other than 1 and itself.
For example, 2, 3, 5, 7, 11 are prime numbers.
A composite number is an integer greater than 1 that is not prime. It has at least one positive divisor other than 1 and itself.
For example, 4, 6, 8, 9, 10 are composite numbers.
Prime Factorization
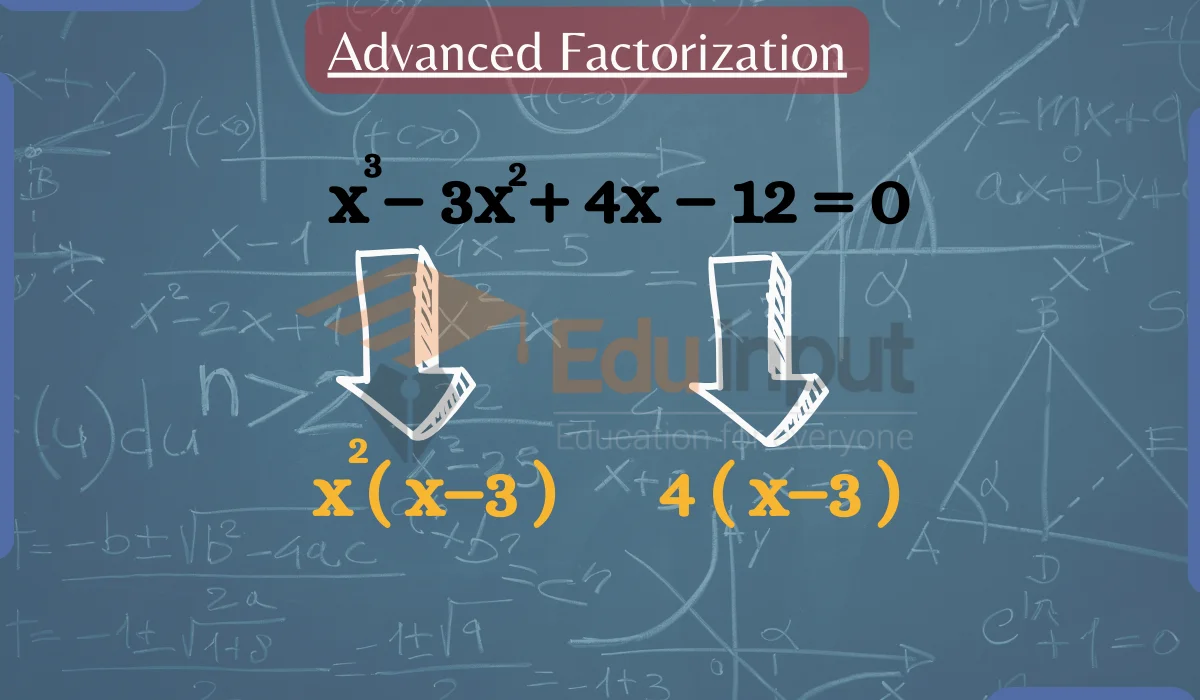
Every composite number can be expressed as the product of prime factors. This is known as the prime factorization of the number.
Example
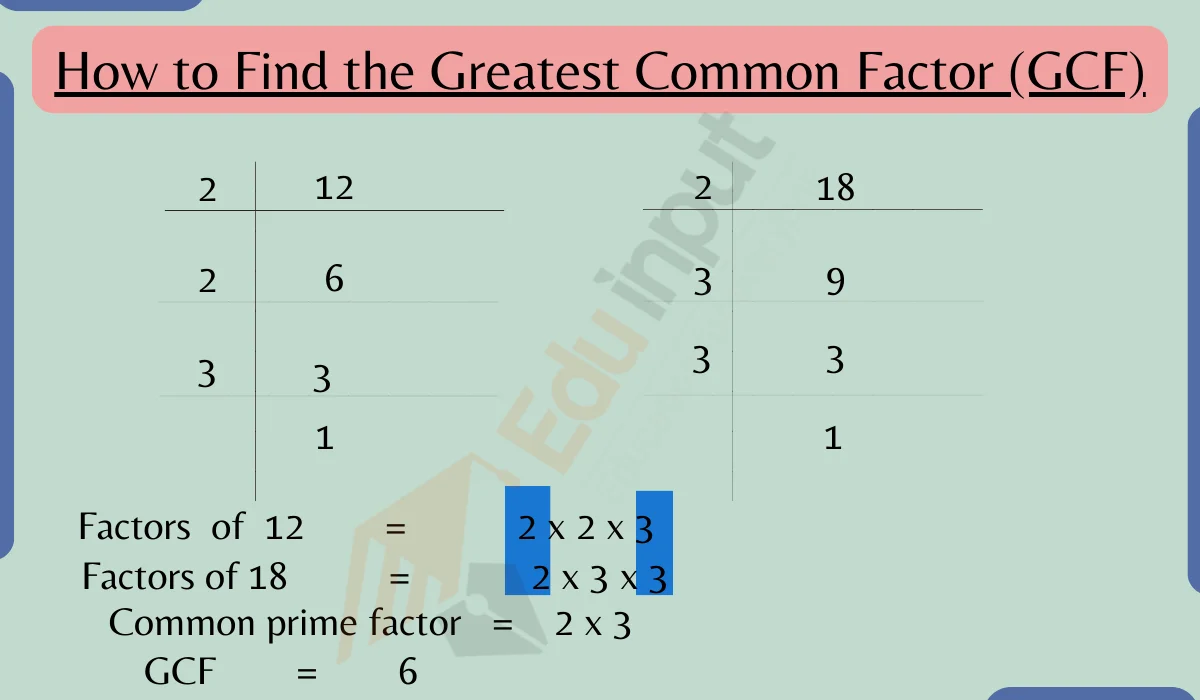
12 = 2 x 2 x 3
Here 2, 2, 3 are prime factors of 12.
Prime factorization can be used to find the greatest common divisor (GCD) and lowest common multiple (LCM) of two numbers easily.
Fundamental Theorem of Arithmetic
The Fundamental Theorem of Arithmetic states that every integer greater than 1 can be represented as the product of prime numbers. Moreover, this representation is unique, apart from the order of the factors.
From this theorem we also see that not only a composite number can be factorized as the product of their primes but also for each composite number the factorization is unique.
In simple words, there exists only a single way to represent a natural number by the product of prime factors. This fact can also be stated as:
The prime factorization of any natural number is said to be unique for except the order of their factors.
In general, a composite number “a” can be expressed as,
a = p1 p2 p3 ………… pn, where p1, p2, p3 ………… pn are the prime factors of a written in ascending order i.e. p1≤p2≤p3 ………… ≤pn.
Example
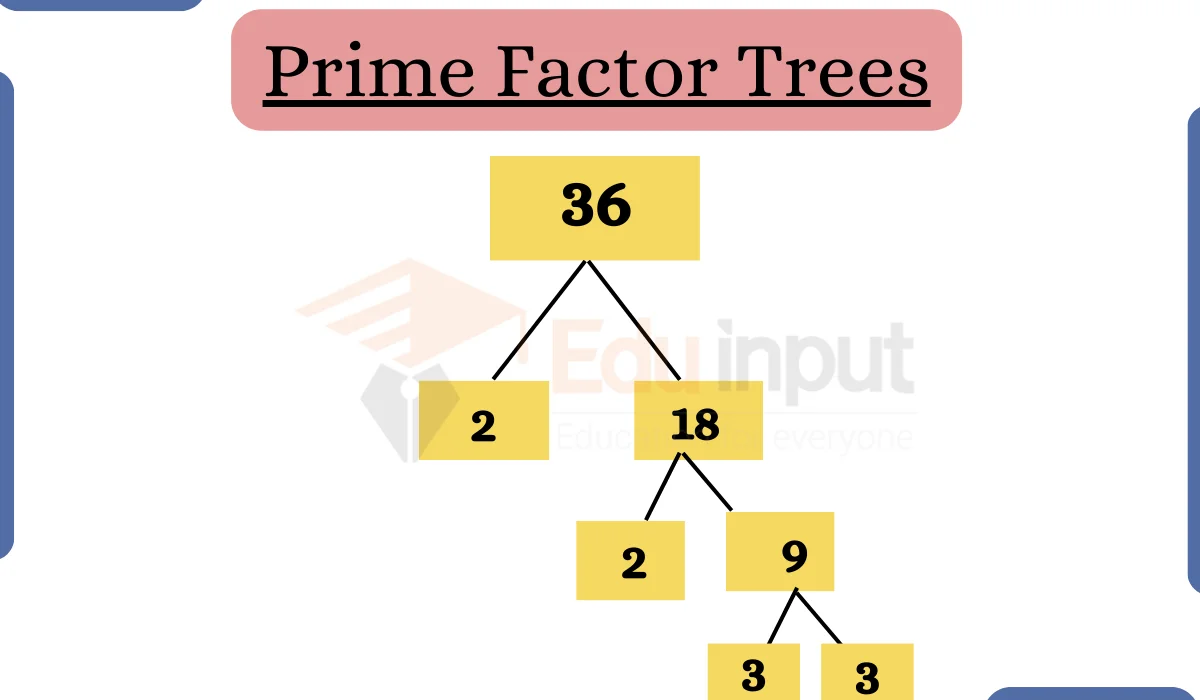
Consider the number 36. By using the Fundamental Theorem of Arithmetic, we can find its prime factorization:
- We divide 36 by 2, which gives us 2 x 18.
- 18 can be divided by 2, resulting in 2 x 9.
- 9 can’t be divided by 2, but it’s a perfect square of 3 x 3.
Now, we have the prime factorization of 36: 2 x 2 x 3 x 3. This is the unique way to express 36 as a product of prime numbers.
FAQs
What is a factor?
A factor is a number that divides evenly into another number. For example, the factors of 12 are 1, 2, 3, 4, 6, 12.
What is the difference between prime and composite numbers?
Prime numbers have exactly two factors – 1 and the number itself. Composite numbers have more than two factors.
What are some examples of prime numbers?
2, 3, 5, 7, 11, 13, 17, 19 are prime numbers. Other examples include 37, 61, 97, 127 etc.
How do you find the prime factorization of a number?
Keep dividing the number by prime numbers successively until you reach the prime factors. For example, the prime factorization of 60 is 2 x 2 x 3 x 5.



Leave a Reply