Simple Harmonic Motion And Circular Motion

There is a relation between Simple Harmonic Motion And circular motion. A body performing the simple harmonic motion will be in circular motion also.

Simple Harmonic Motion

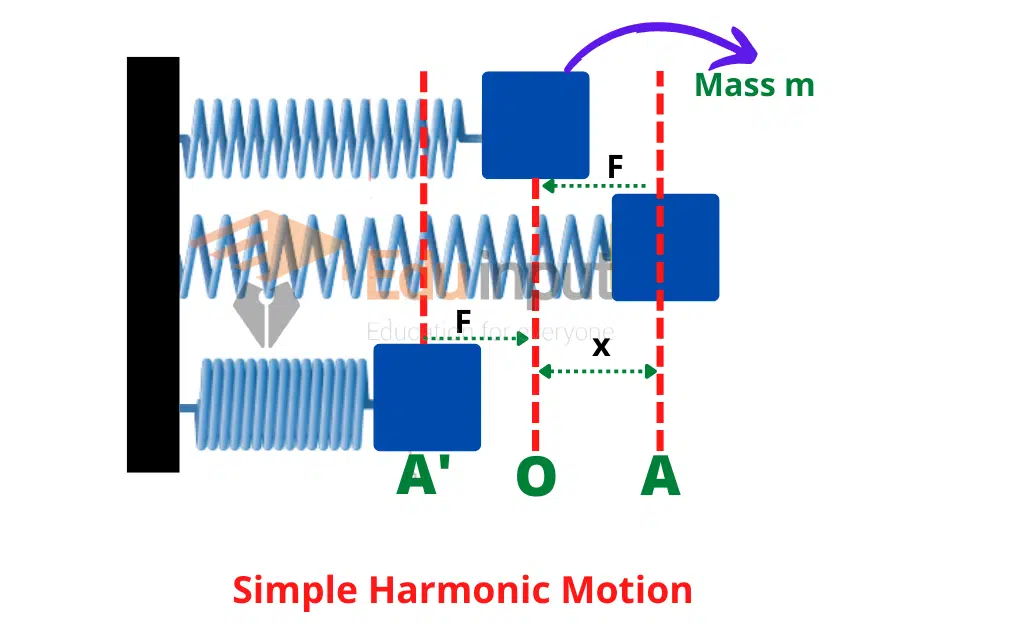
An indirect geometrical method can be used to find mathematical expressions for describing the simple harmonic motion. Let a mass m attached to the end of a vertical spring vibrates simply harmonically with period T, frequency f, and amplitude X0.

The motion of the mass is displayed by the pointer P1 on the line BC with A as the mean position and B and C as the extreme position.

At t= 0, when no force is acting on, the pointer P₁ is at means position A.

The pointer moves between extreme positions B and C in a way that it moves from A to B to A, A to C, and then back to A at time instants T/4, T/2, 3T/4, and T respectively. This will complete one cycle of vibration. During this time, the amplitude of vibration will be x_0.

Simple Harmonic Motion And circular motion

The concept of Simple Harmonic Motion And circular motion is introduced by considering that a point P moves on a circle of radius X₀, with uniform angular frequency ω= 2 π /T. The radius of the circle is the same as the amplitude of vibration of the pointer’s motion. Draw a perpendicular PN on the vertical diameter of the circle and point N is called the projection of the point P on the diameter DE which is parallel to the line of vibration of the pointer.

The points D and E are at the same level as the points B and C. As point P describes the uniform circular motion with a constant angular velocity ω, N oscillates to and fro on the diameter DE with the time period T. The position of P at t= 0 is 0₁ on the circle and N is at the mean position O.

After time T/4 T/2 3T/4 and T the point N will be at D, O, E, and O respectively.

Comparing the motions of N and pointer P₁, it is clear that both are identical.

Instantaneous Displacement

It is the distance of the projection of point N from the mean position 0 at any instant. As it is clear from the figure that point P makes angle <O₁OP= θ then angle NPO is also θ. ON/OP=sin θ

X/X0=sin θ

x=x₀ sin θ

x=x₀sin ω t

This will also be the displacement of the pointer P₁at the instant t.

 The phase of Vibration:

The value of x is the function of θ. it draws a similar waveform that was traced experimentally but here it has been traced theoretically by linking SHM with circular motion.

When θ =0 radian then x= 0

When θ =90⁰= π /2radianthen x=x₀

When θ =180° = π radian then x = 0

When θ =270⁰=3 π/2 radian then x=-x₀

When θ= 360°= 2 π radian then x = 0

Here θ is called the phase of vibration. This phase is also related to the circular motion aspect of SHM.

Instantaneous velocity

The linear velocity of point p at any instant of time t will be directed along the tangent to the circle and its magnitude will be

 v_p =r ω

r=x₀

v_p=x₀ ω

At the mean position where x=0, velocity is maximum

V= ω √x_o²-x²= ω √x_o²-0= ω √x_o²= ω x_o

At the extreme position where x=x_o , velocity is minimum

V= ω √x_o²-x²= ω √x_o²-x_o²= ω √x_o²=0