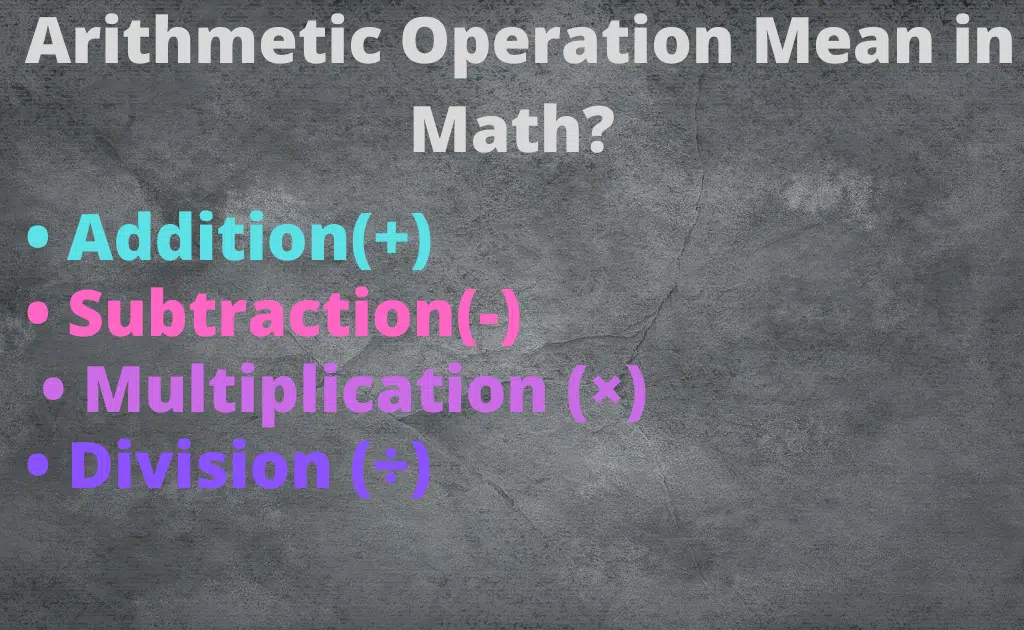
What is the Geometric Mean?

The geometric mean of a variable X is the nth positive root of the product of the x1,x2,x3,……….,xn observations. in symbols, we write

G.M=(x₁.x₂.x₃……,x_n)^1/n

The geometric mean of series containing n observations is the nth root of the product of values.

G.M=(x₁.x₂.x₃……,x_n)^1/n

The above formula can also be written by using a logarithm

1. For Ungroup Data

G.M=Antilog (sigma logX/n)

1. For Group Data

G.M=Antilog (sigma f logX/sigma f)

For example:

Find the geometric mean of the observation 2, 4, 8, by using the basic formula

Solution: given observations 2, 4, 8

n=3

Using formula

G.M=(x₁,x₂,x₃,……,x_n)^1/n

G.M=(2x4x8)^1/3

G.M=(64)^1/3

G.M=(4³)^1/3

G.M=4

For example: Find the geometric mean of observations 2, 4, 8 using the logarithmic formula for ungrouping data.

Solution: Given observations 2, 4, 8

X	log
2	0.3010
4	0.6021
8	0.9031
total	Sigma log X=1.8062

Using formula:

G.M=Antilog (sigma logX/n)

 G.M=Antilog (1.8062/3)

G.M= Antilog (0.6021)

G.M= 4.00003

G.M=4

For example: – Find the geometric mean for the following group data.

Marks in percentage	Frequency /no. of students
33–40	28
41–50	31
51–60	12
61–70	9
71–75	5

Solution: we proceed as fellows

classes	f	X	Log(X)	f(log)
33–40	28	33+40/2=36.5	Log(36.5)=1.562293	43.7442
41–50	31	41+50/2=45.5	Log(45.5)=1.658011	51.39835
51–60	12	51+60/2=55.5	Log(55.5)=1.744293	20.93152
61–70	9	61+70/2=65.5	Log(65.5)=1.816241	16.34617
71–75	5	71+75/2=73	Log(73)=1.863323	9.316614
	Sigma f =85			Sigma  f(logX) =141.7369

Using formula:

G.M=Antilog (sigma f logX/sigma f)

G.M=Antilog (141.7369/85)

G.M=Antilog (1.66749)

G.M=46.50% marks