Roots of a Quadratic Equation | Discriminate of Quadratic Equation
We will discuss here the different cases of discriminant to understand the nature of the roots of a quadratic equation.
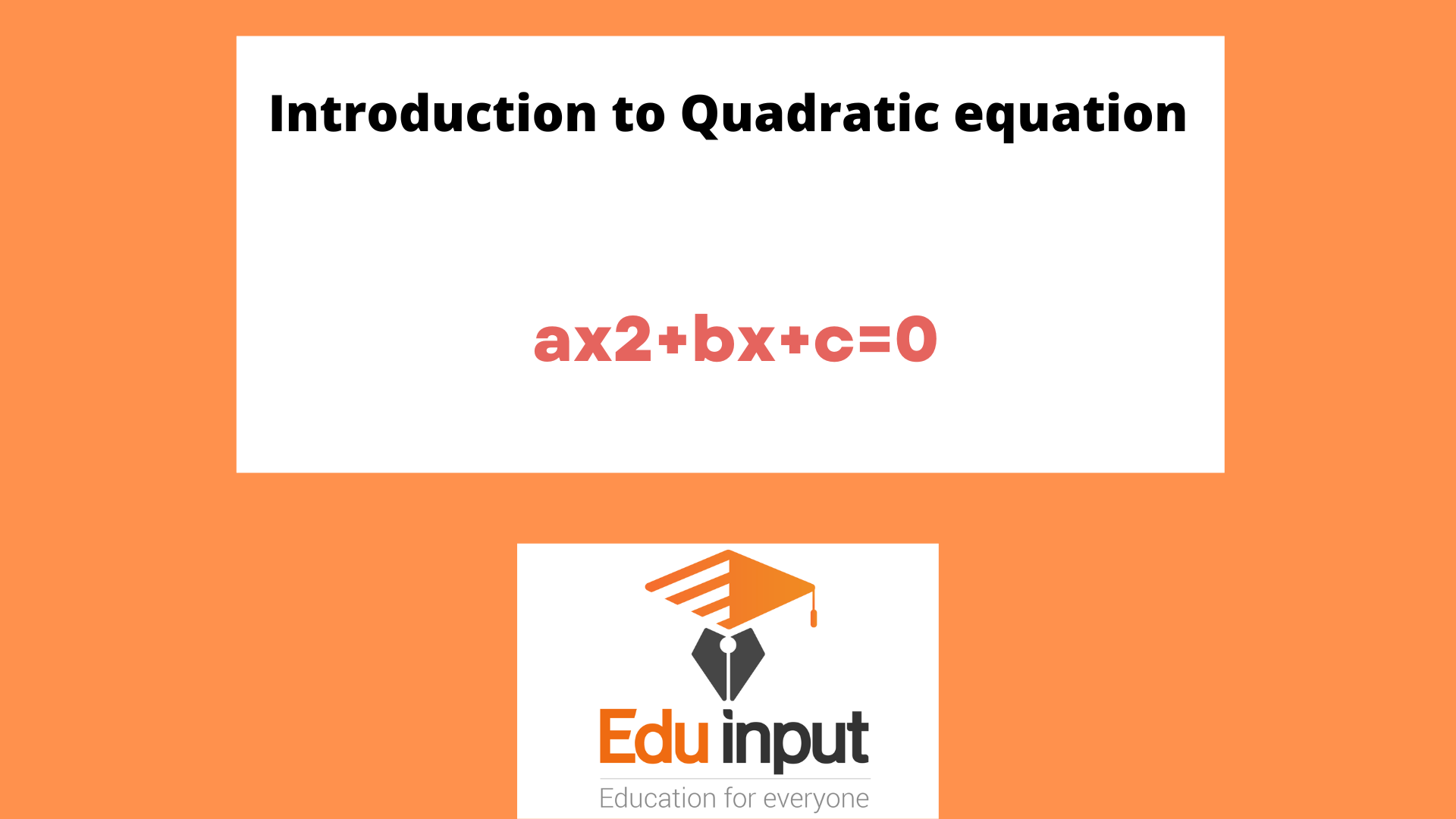
We know that the roots of the quadratic equation ax2+bx+c=0 where a≠0 are given by the quadratic formula as:
x=-b±√(b^2-4ac/2a
We see that there are two possible values for x, as discriminated by the parts of the formula
±√ (b^2-4ac).
The nature of the roots of an equation depends on the value of the expression b2-4ac, which is called its discrimination.
Discriminate of Quadratic Equation
The expression (b2-4ac) is called the discriminant of the quadratic equation.
Generally denoted by discriminant of the quadratic equation, D or ∆
Therefore,
Discriminant =∆= b2-4ac
We shall discuss the following cases about the nature of the roots of the quadratic equation ax2+bx+c=0
When a,b, and c are real numbers,a≠0
Case:1.When discriminant is equal to zero(b2-4ac=0)
If the discriminant is equal to zero (b2-4ac=0), then the roots of a quadratic equation are real and repeated equally.
For example:- find the nature of the roots of the quadratic equation .9x2-12x+4=0 by solving them
Solution: 9x2-12x+4=0 comparing with ax2+bx+c=0
We have, a=9, b=-12, c=4
Discriminant =∆=b2-4ac
Discriminant =∆= (-12)2-4(9)(4)
Discriminant =∆= 144-144
Discriminant =∆=0
Clearly, the discriminant of the given quadratic equation is zero.
Therefore, the roots of the given quadratic equation are real and equal.
Solve the equation:
9x2-12x+4=0
9x2-6x-6x+4=0 ∴ by using the factorization method
3x (3x-2)-2(3x-2) =0
(3x-2)(3x-2)=0
3x-2=0 or 3x-2=0
3x=2 3x=2
X=2/3 x=2/3
The given equation has two solutions: 2/3 and 2/3
Solution set ={2/3,2/3}
The solution of an equation is also called its roots.
Clearly, the roots of a given quadratic equation are real and equal.
Case:2.When discriminant is less than zero or negative (b2-4ac<0)
If the discriminant is negative (b2-4ac<0) then√(b^2-4ac will be imaginary so the roots of a quadratic equation are complex/imaginary and distinct/unequal.
For example: Discuss the nature of the roots of a quadratic equation.x2+2x+3=0
Solution:
x2+2x+3=0 comparing with ax2+bx+c=0,we have
a=1 ,b=2,c=3
Discriminant =∆=b2-4ac
Discriminant =∆=(2)2-4(1)(3)
Discriminant =∆=4-12
Discriminant =∆=-8
Clearly, the discriminant of the given quadratic equation (2nd-degree polynomial) is negative.
Therefore, the roots of the given quadratic equation are imaginary and distinct/unequal.
Solve the equation:
x2+2x+3=0
Comparing the standard form of quadratic equation
a=1,b=2,c=3
By using the quadratic formula
x=-b±√(b^2-4ac/2a
Put the value a, b and c
x=-(2)±√(2^2-4(1)(3)/2(1)
x=-2±√(4-12/2
x=-2±√(-8/2
x=-2±√(4X2 i/2
x=-2±2√(2 i/2
x=2(-1±√(2 i)/2
x=-1±√2 i
Clearly, the roots of the given equation are imaginary and unequal
Case:3. When discriminant is greater than zero or positive (b2-4ac>0)
If the discriminant is positive (b2-4ac>0) then √(b^2-4ac will be real .then the roots of a quadratic equation are real and distinct/unequal.
For example:- Discuss the nature of the roots of quadratic equation 2x2+5x-1=0
Solution:
2x2+5x-1=0 comparing with ax2+bx+c=0 we have
a=2,b=5,c=-1
Discriminant =∆=b2-4ac
Discriminant =∆= (5)2-4(2)(-1)
Discriminant =∆=25+8
Discriminant =∆=33
Clearly, the discriminant of a given quadratic equation is positive.
Therefore, the roots of the given quadratic equation are real and distinct /unequal.
Case:4. When discriminant is greater than zero and perfect square (b2-4ac>0 )
If the discriminant is greater than zero (b2-4ac>0) or positive and perfect square then the roots of a quadratic equation are real, rational, and unequal.
For example: Discuss the nature of the roots of the quadratic equation (p+q) x2-px-q=0
Solution:
(p+q) x2-px-q=0 comparing with ax2+bx+c=0 we have
a=(p+q) ,b=-p ,c=-q
Discriminant =∆=b2-4ac
Discriminant =∆=(-p)2-4(p+q)(-q)
Discriminant =∆=p2+4pq+4q2
Discriminant =∆=p2+2(p)(2q)+(2q)2
Discriminant =∆= (p+q) 2
Which is a perfect square
Hence the roots of a given quadratic equation are
Real, rational, and unequal
For example: x2+4x+3=0
Solution:
x2+4x+3=0 comparing with ax2+bx+c=0 we have
a=1 ,b=4 ,c=3
Discriminant =∆=b2-4ac
Discriminant =∆=(4)2-4(1)(3)
Discriminant =∆=16-12
Discriminant =∆=4
Discriminant =∆=(2)2 >0
Which is a perfect square
Solve the equation:
x2+4x+3=0
By using the factorization method
X2+3x+x+3=0
X(x+3)+1(x+3)=0
(x+3)(x+1)=0
X+3=0 or x+1=0
X=-3 x=-1
Solution set={-3,-1}
Clearly, the discriminant is given an equation greater than zero and a perfect square.
Clearly, the roots of the given equation are real, rational, and unequal.
Case 5:- When the discriminant is greater than zero and not a perfect square (b2-4ac>0 )
If the discriminant is positive but not a perfect square then the roots of the quadratic equation are real, irrational,unequal
For example: Discuss the nature of the roots of quadratic equations.4x2+6x+1=0
Solution:
4x2+6x+1=0 comparing with ax2+bx+c=0 we have
a=4 ,b=6 ,c=1
Discriminant =∆=b2-4ac
Discriminant =∆= (6)2– 4(4)(1)
Discriminant =∆=36-16
Discriminant =∆= 20>0 but not a perfect square
Clearly, if the discriminant of the given quadratic equation is greater than zero but not perfect square the roots of the quadratic equation are real, unequal, and irrational.

 written by
written by