Methods for Factoring Quadratic Equations
Factoring quadratic equations is an important skill in algebra that helps us solve a variety of mathematical problems.
In this article, we’ll discuss several methods for factoring quadratic equations.

What is a Quadratic Equation?
A quadratic equation is a polynomial equation of the form:
ax2+bx+c=0
Where a, b, and c are constants, and x is the variable . Quadratic equations often represent parabolic curves and can have two real solutions, one real solution, or no real solutions, depending on the discriminant b2 – 4ac.
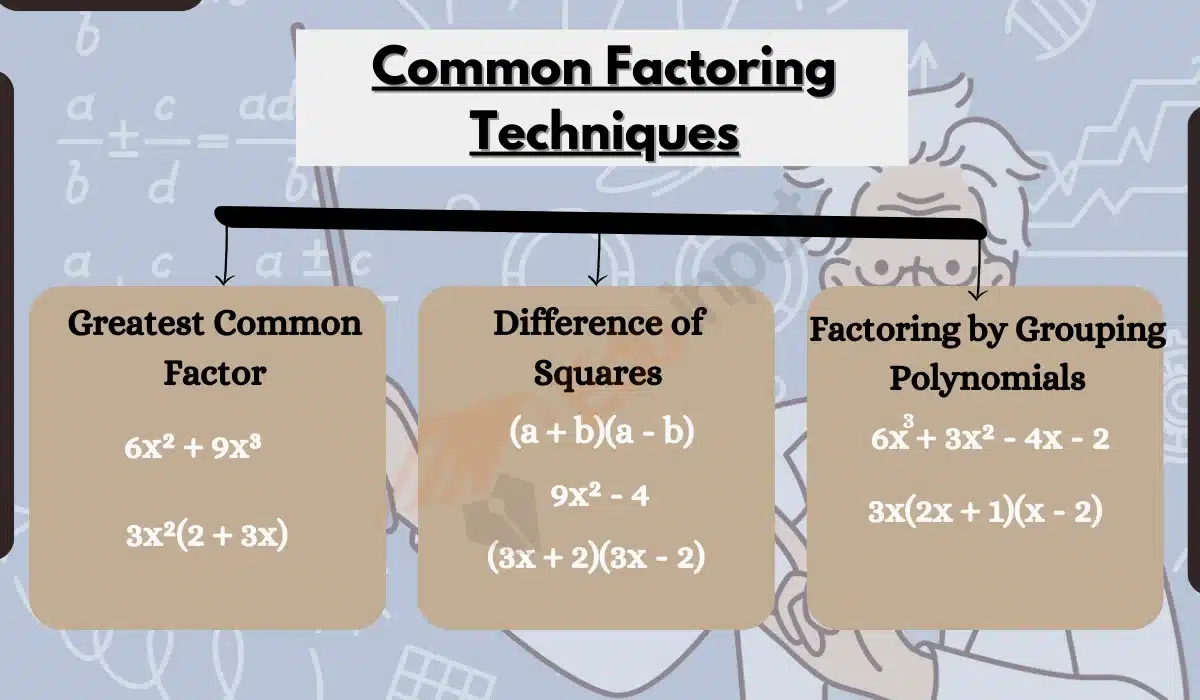
Methods for Factoring Quadratic Equations
Factoring by Grouping
One of the simplest methods for factoring quadratic equations is factoring by grouping. This method works when you group terms within the equation.
- Group terms in pairs.
- Factor out the greatest common factor from each group.
- Look for a common factor in the resulting expressions.
- Factor out the common factor from both groups.
- You now have a factored quadratic equation.
Example
Factor 3x2 – 6x + 2x – 4.
Step 1: Group terms:
(3x2 – 6x) + (2x – 4)
Step 2: Factor out the greatest common factor from each group:
3x(x – 2) + 2(x – 2)
Step 3: Look for a common factor: (x – 2) is common.
Step 4: Factor out the common factor:
(x – 2)(3x + 2)
Step 5: The factored equation is (x – 2)(3x + 2).
Factoring with the Quadratic Formula
When factoring by grouping is not applicable, you can use the quadratic formula to factor quadratic equations.
The quadratic formula is:
x = [-b ± √( b2 – 4ac)]/ 2a
- Calculate the discriminant ( b2 – 4ac).
- Find the values of (x) using the quadratic formula.
- The factored equation is (x−Root 1)(x−Root 2), where Root 1 and Root 2 are the solutions for x.
Example
Factor (x^2 – 5x + 6).
Step 1: Calculate the discriminant:
b2 – 4ac = (-5)^2 – 4(1)(6) = 25 – 24 = 1
Step 2: Find the values of (x):
x = −(−5) ± √1/ 2(1)
= 5 ± 1/ 2
Step 3: The factored equation is (x – 2)(x – 3).
Factoring by Completing the Square
Another method for factoring quadratic equations is completing the square. This method is especially useful when the equation is not easily factorable.
- Rewrite the equation in the form ax2 + bx = -c.
- Complete the square by adding and subtracting (b/2a)2 to the equation.
- Rewrite the equation as a perfect square trinomial.
- Solve for (x) by taking the square root of both sides.
- The factored equation is ((x – h)^2 = k), where ((h, k)) are the coordinates of the vertex.
Example
Factor (x2 – 4x + 4).
Step 1: Rewrite the equation:
x2 – 4x = -5
Step 2: Complete the square:
x2 – 4x + 4 – 4 = 0
Step 3: Rewrite as a perfect square:
(x – 2)2 = 0
Step 4: Solve for (x):
x – 2 = 0
x = 2
Step 5: The factored equation is ((x – 2)2 = 0).
FAQs
What if the discriminant is negative when using the quadratic formula?
If the discriminant is negative, there are no real solutions, and the equation does not factor easily. You can use complex numbers to find the solutions.
Are there other methods to factor quadratic equations?
Yes, there are other methods, like factoring by substitution and factoring using special patterns (e.g., the difference of squares). These methods can be used depending on the equation’s form.
Why is factoring quadratic equations important?
Factoring helps us solve equations, find roots, and understand the behavior of quadratic functions. It is a fundamental skill in algebra and has practical applications in various fields, including physics and engineering.