Obtuse Angle- Examples, Degree, And Obtuse Angle Triangle

An obtuse angle is an angle that is greater than 90 degrees and less than 180 degrees. In other words, it is an angle between a right angle and a straight angle. Obtuse angles are named after the Latin word obtusus, which means “blunted” or “stupefied”.

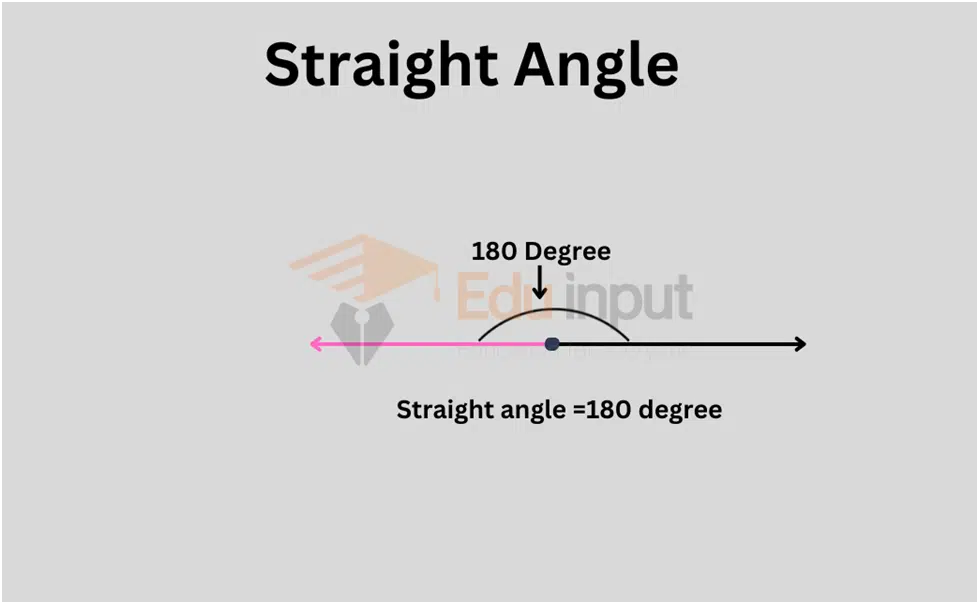
Other types of angles include acute angles, which measure less than 90 degrees, reflex angles, which measure greater than 180 degrees but less than 360 degrees, straight angles, which measure exactly 180 degrees, and full angles, which measure exactly 360 degrees. These angles have unique properties and use in geometry and other fields.

In this article, we will discuss the obtuse angle, examples, degree, and triangle, which will be very helpful for you.

Key Points

1. An obtuse angle measures more than 90 degrees but less than 180 degrees and is wider than a right angle. 2. It gets its name from the Latin word obtusus, meaning “blunted” or “stupefied”. 3. Examples of obtuse angles include 165°, 135°, 110°, 179°, and 91°. 4. In a triangle, if one of its angles is greater than 90 degrees, the triangle is called an obtuse triangle and the side opposite the obtuse angle is always the longest. 5. In an obtuse-angled triangle, the sum of the squares of the two shorter sides is always less than the square of the longest side. 6. Real-life examples of obtuse angles include the angle between the hour and minute hand of a clock at 4 o’clock, the angle between the base of an open laptop and its screen, and the angles formed by the blades of a ceiling fan.

---

Examples of Obtuse Angle

Examples of obtuse angles are given below.

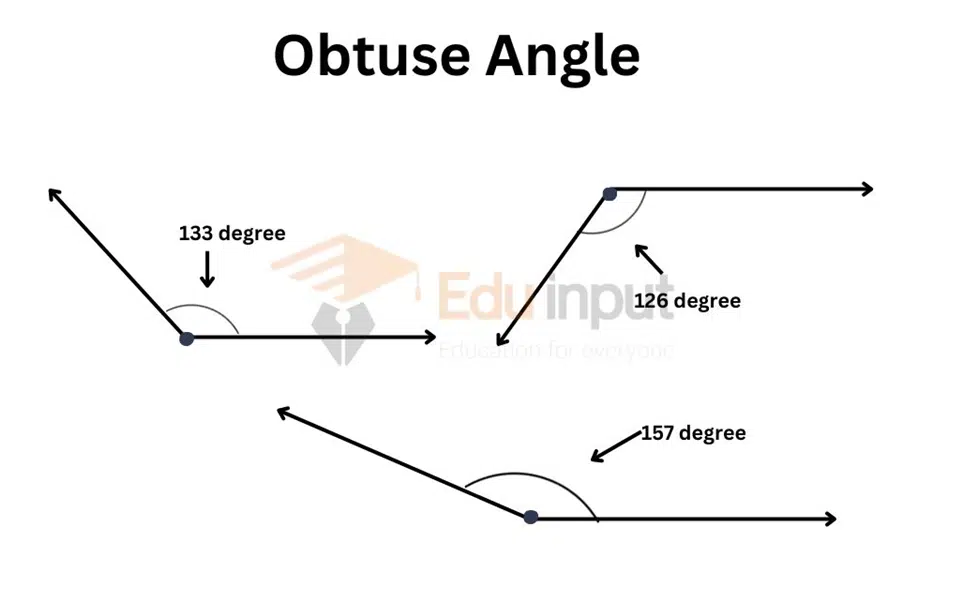
Obtuse Angle Degree  

An angle that measures less than 180 degrees but more than 90 degrees is classified as an obtuse angle. Some examples of obtuse angles would be 165°, 135°, 110°, 179°, 91°, and so on.

So basically, if the degree of an angle is anywhere within the range of 90° to 180° then it can be classified as an obtuse angle.

Obtuse Angle Triangle

A triangle is considered obtuse when one of its angles is greater than 90 degrees. Obtuse triangles can be either isosceles or scalene, but never equilateral since an equilateral triangle contains three angles that are each 60 degrees, making the total angle sum 180 degrees – which means there can be no angles greater than 90 degrees.

The side opposite the obtuse angle in a triangle is always the longest side of that triangle. Additionally, a triangle can never be both a right angle and an obtuse angle simultaneously – this is due to the angle sum property of triangles which states that the sum of a triangle’s angles must equal 180 degrees.

If one of the angles of a triangle is obtuse, then the other two angles of the triangle must be acute angles.

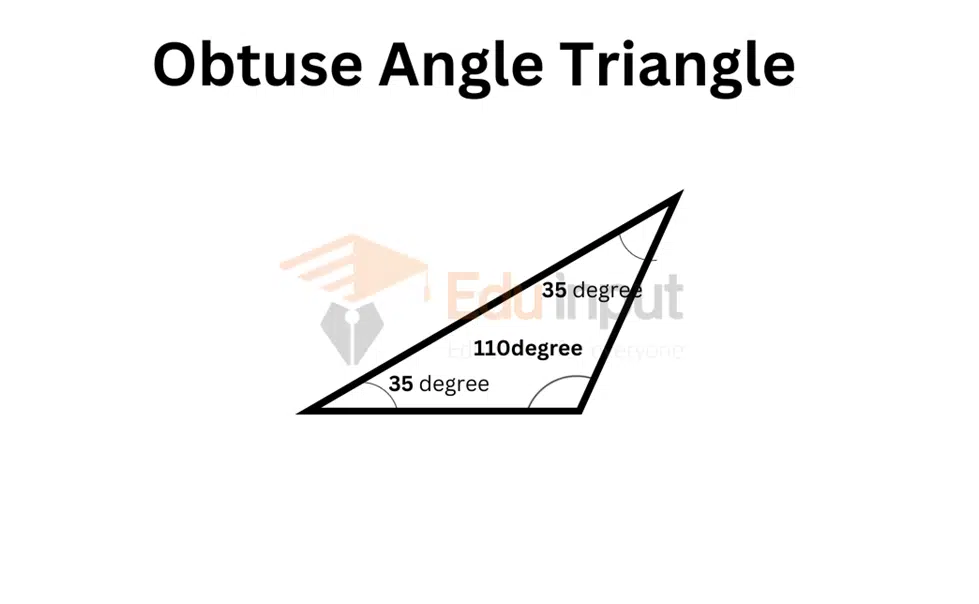
The triangle above has one angle that is greater than 90° and as a result, they are classified as obtuse-angled or obtuse triangles. In an obtuse-angled triangle, the sum of the squares of the two shorter sides is always less than the square of the longest side.

For example, in ΔABC where the sides measure a, b, and c with c being the longest side, we would have a² + b² < c². Put another way, if in any triangle, a² + b² < c², then that triangle is considered an obtuse triangle.

Real-Life Example of Obtuse Angle

Here are some examples of obtuse angles that you might see in real life:

• The angle between the hour and minute hand of a clock at 4 o’clock.
• The angle between the base of an open laptop and its screen.
• Angles are formed by the blades of a ceiling fan.

Acute and Obtuse Angle

There are different types of angles based on measurement. Acute angles measure less than 90 degrees, while obtuse angles measure greater than 90° but less than 180°. See the image below for examples of acute and obtuse angles, followed by their difference.

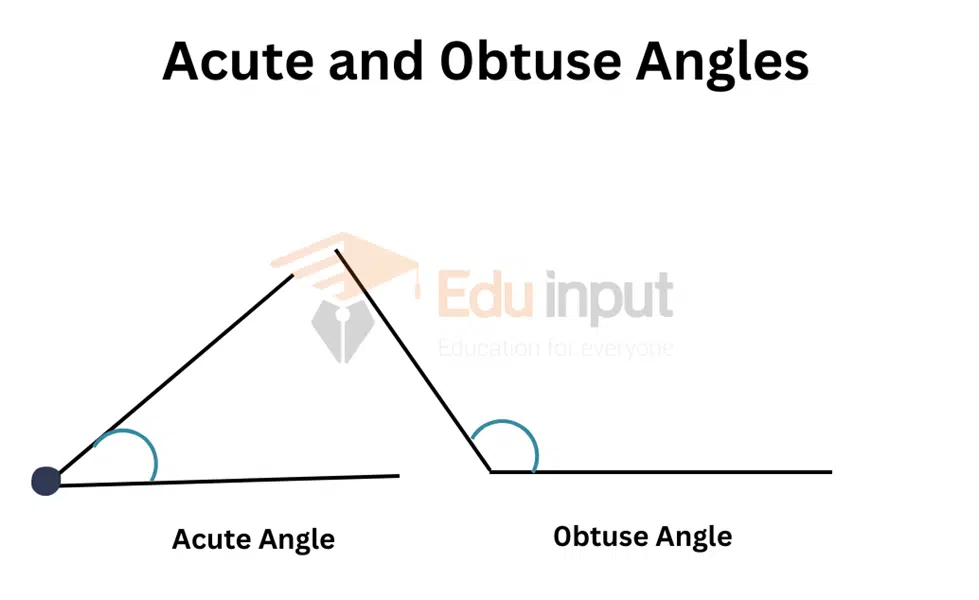
All angles that measure more than 90° and less than 180° are classified as obtuse angles. In an obtuse angle triangle, the sum of the squares of the two shorter sides is always less than the square of the longest side.

Frequently Asked Question-FAQs