Derivative of a Product Rule-Product Rule Formula

Product rule in calculus is a method to determine the differentiation or derivative of a given function in the form of the product of two differentiable functions.

That means, we can apply the product rule, or the Leibniz rule, to calculate the derivative of a function of the form given as: f(x)·g(x), such that both f(x) and g(x) are differentiable. The product rule follows the concept of derivatives and limits in differentiation directly.

Let us understand the product rule formula, its proof using solved examples in detail in this article.

What is a product rule?

Product rule in calculus is a method used to determine the derivative of any function given in the form of a product obtained by the multiplication of any two differentiable functions. The product rule in words that the derivative of a product of two differentiable functions is equal to the sum of the product of the second function with differentiation of the first function and the product of the first function with the differentiation of the second function. That means if we are given a function of the form: f(x)·g(x), we can calculate the derivative of this function using the product rule derivative as

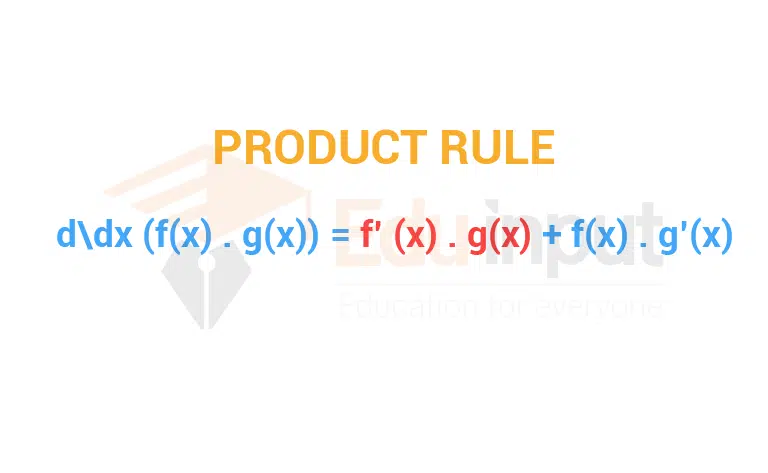
Product rule formula:

We can evaluate the differentiation or find the derivative of the product of two functions using the product rule formula in Calculus. The product rule formula is given as

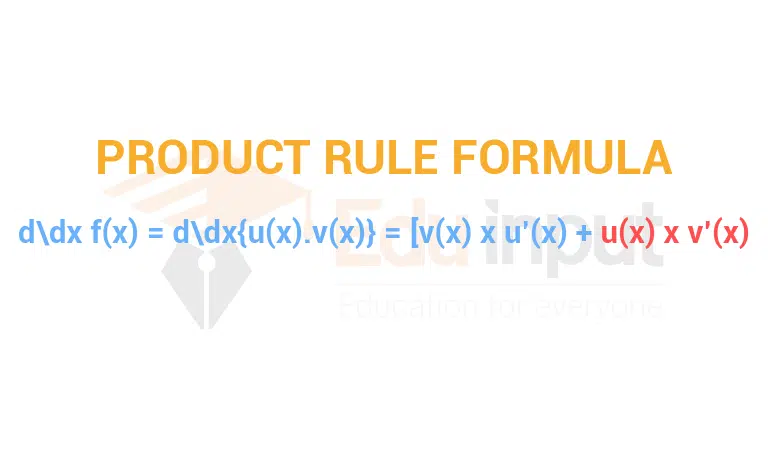
Where,

• f(x) = Product of differentiable functions u(x) and v(x)
• u(x)= Differentiable functions
•  v(x) = Differentiable functions
• u'(x) = Derivative of function u(x)
• v'(x) = Derivative of the function v(x)

Derivative of a Product Rule

In the previous section, we understand the product formula to determine the derivatives of the product of two differentiable functions. For any two functions, the product rule may be given in

 Lagrange’s notation as

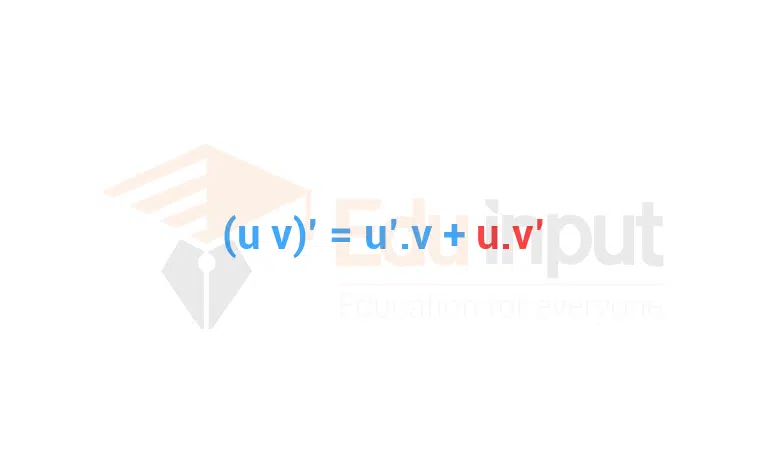
or in Leibniz’s notation as

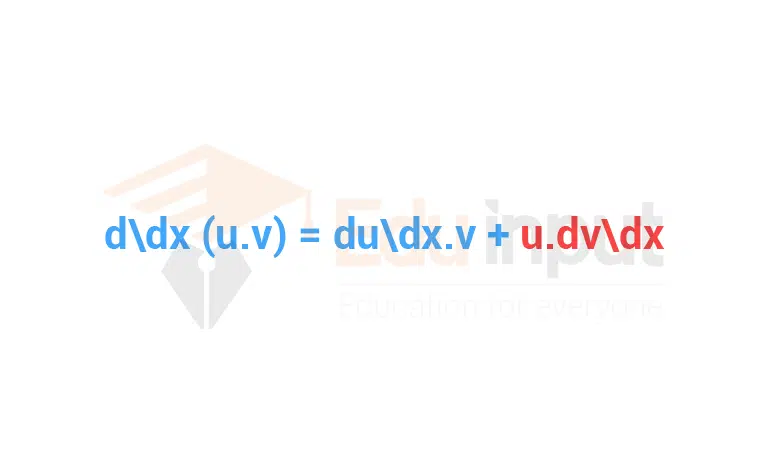
the proof of the product rule formula here. There are distinct methods to prove the product rule formula, given as,

• using the chain rule
• using the first principle