Difference Between Factors and Multiples

Understanding the difference between factors and multiples lays the foundation for more complex concepts in mathematics.

In this article, we’ll discuss these fundamental ideas, unraveling their meanings and exploring their applications.

What are the Factors?

A factor is a number that divides evenly into another number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12.

To find the factors of a number, systematically test all numbers from 1 up to that number to see which divide evenly.

What are Multiples?

The multiples of a number are the numbers in its times table. The multiples result from multiplying that number by 1, 2, 3, and so on.

For example, the multiples of 4 are 4, 8, 12, 16, 20, and so on.

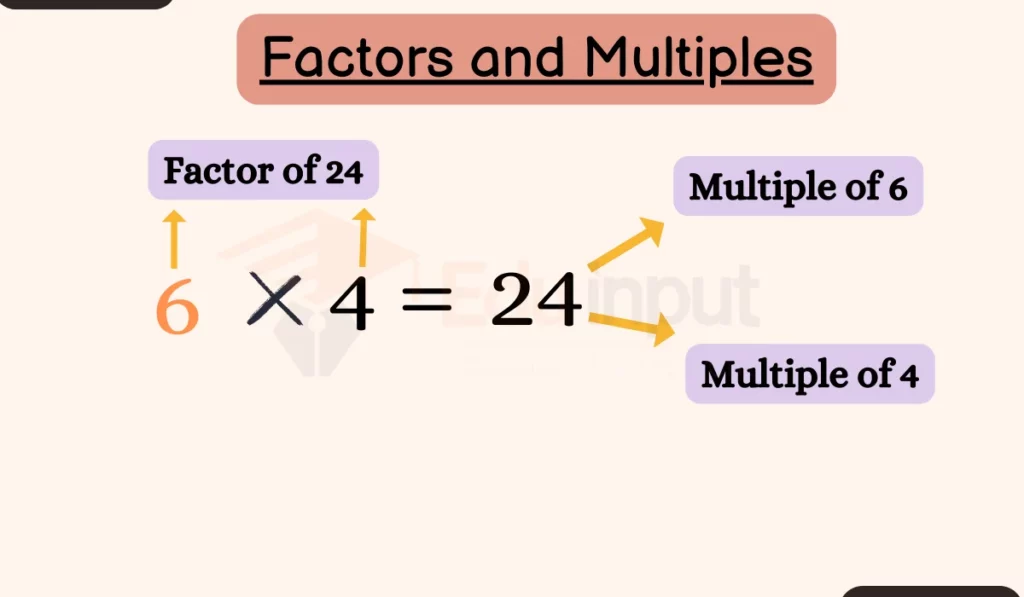
Difference Between Factors and Multiples

S.No	Factors	Multiples
1	Factors are exact divisors of a number.	Multiples are numbers obtained by multiplying others.
2	The number of factors is finite.	The number of multiples is infinite.
3	The operation used to find factors is division.	The operation used to find multiples is multiplication.
4	The outcome of factors is less than or equal to the given number.	The outcome of multiples is greater than or equal to the given number.

How to Find Factors and Multiples

Finding Factors:

• List all the factors of the given number.
• Check which numbers divide evenly, leaving no remainder.

Finding Multiples:

• Start with the given number.
• Multiply it by consecutive integers to find multiples.

Examples of Finding Factors

Let’s find the factors of 24:

1 – divides 24

2 – divides 24

3 – divide 24

4 – divides 24

6 – divides 24

8 – divides 24

12 – divides 24

24 – divides 24

So the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24.

Examples of Identifying Multiples

Let’s look at the multiples of 6:

6 x 1 = 6

6 x 2 = 12

6 x 3 = 18

6 x 4 = 24

The multiples of 6 are 6, 12, 18, 24, 30, etc.

You can also think of multiples in terms of “counting”: 6, 12, 18 , 24, 30, 36, 42, 48, etc.

Common Factors and Multiples

Two numbers share a common factor if they have any of the same factors besides 1.

For example, 12 and 18 have common factors of 2, 3, and 6.

Two numbers share a common multiple if they are both multiples of the same number.

For example, common multiples of 4 and 6 include 12, 24, 36, etc.

Finding common factors and common multiples is useful for simplifying fractions and finding equivalent fractions.

Solved Examples:

Example 1

Find the factors of 18.

Factors of 18: 1,2,3,6,9,18

These numbers divide 18 without leaving any remainder.

Example 2

Find the first three multiples of 5.

Multiples of 5: 5,10,15

These are obtained by multiplying 5 by 1, 2, and 3.

FAQs