Factorization of Algebraic Expressions: Key Concepts

Factorization is the process of expressing a number or algebraic expressions as a product of its factors. It involves breaking down a complex expression into simpler, multiplicative components.

In this article, we will discuss some key concepts of factorization of algebraic expressions.

Prime Factorization

This involves expressing a number as the product of its prime factors.

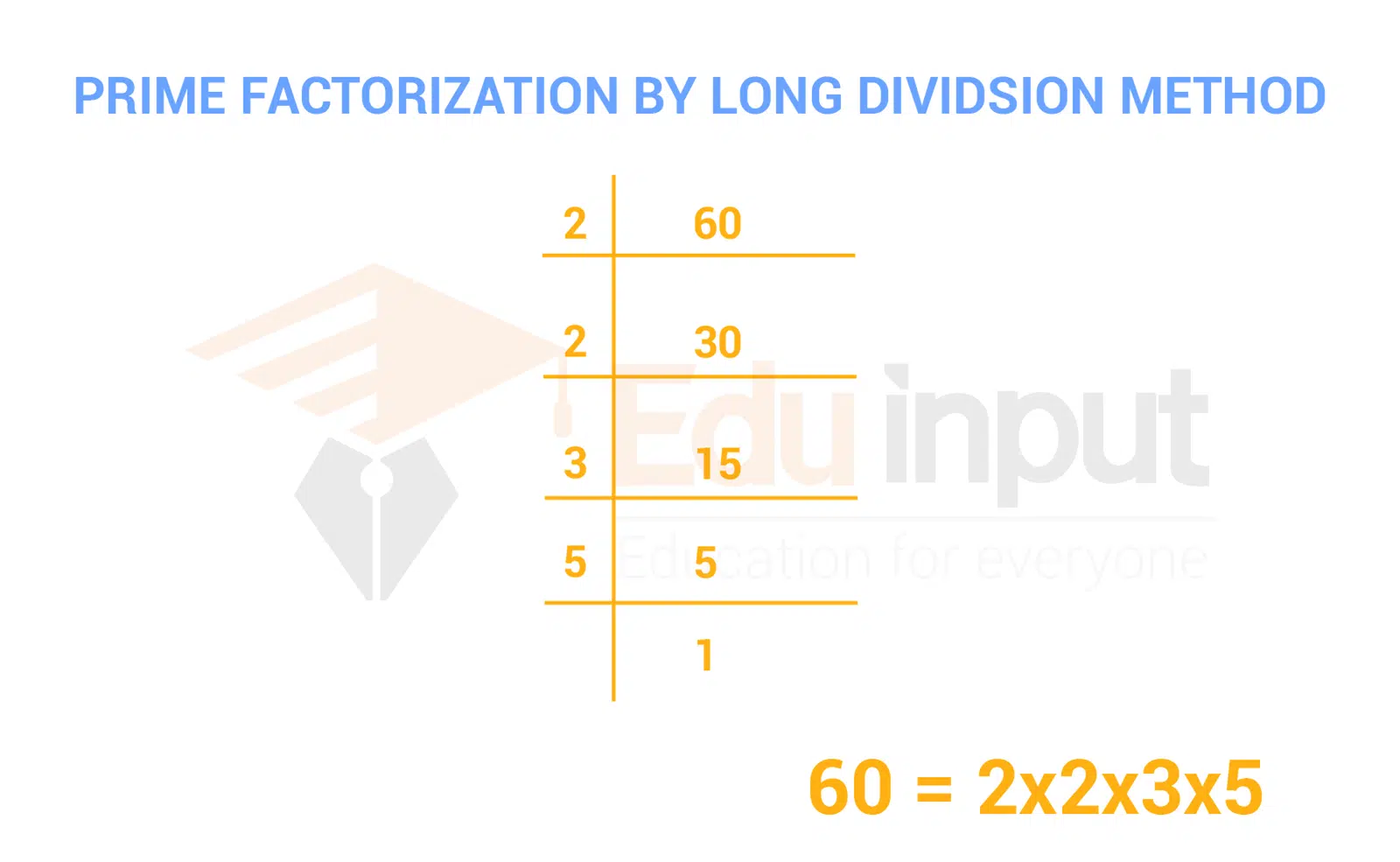
For example, the prime factorization of 60 is:

60 = 2 x 2 x 3 x 5

The prime factors of 60 are 2, 2, 3 and 5.

Greatest Common Factor

Greatest common factor (GCF) of two or more expressions is the largest factor common to all the expressions. Finding the GCF helps simplify fractions and factor expressions.

For example, the GCF of 12 and 18 is 6.

GCF of 12 and 18 = 6

Factoring Quadratics

Quadratic expressions like x² + 5x + 6 can be factorized into the product of two binomials, using various methods like taking out the common factor, grouping, completing the square, etc.

For example,

x² + 5x + 6 = (x+2)(x+3)

Factoring quadratics allows us to solve quadratic equations or simplify expressions.

Difference of Squares

Expressions having the form a² – b² can be factorized as:

a² – b² = (a + b)(a – b)

For example,

9 x² – 4 = (3x + 2)(3x – 2)

This factorization pattern is very useful in simplifying algebraic fractions.

Factoring Cubics

Cubic polynomials like x³ + x² + 3x + 1 can sometimes be factorized into binomials when they have a common factor.

For example,

x³+ 3x² + 3x + 1 = x(x+1)(x+1)

Solved Examples

Example 1

Factorize completely: 24xy – 18x

Solution

24xy – 18x

= 6x(4y – 3)

Example 2

Factorize: x^2 – 9

Solution

x² – 9

= (x + 3)(x – 3)

Example 3

Factorize: x³ – 8x

Solution:

x³ – 8x

= x(x² – 8)

= x(x – 2)(x + 4)

FAQs