Four Fourth Roots Of Unity

The number 1 has four fourth roots: 1, -1, I, and -i. You should find that if you raise any of these to the power four you get 1.

Proof:

Let x be the fourth roots of unity

X=∜1

X= (1) 1⁄4

Taking power 4 on both side

X⁴=((1)1/4)⁴

X⁴=1

X⁴-1⁴=0

((x) ²)²-((1)²)²=0

By using

a² – b²= (a – b) (a + b)

(X² – 1)(X² + 1)=0

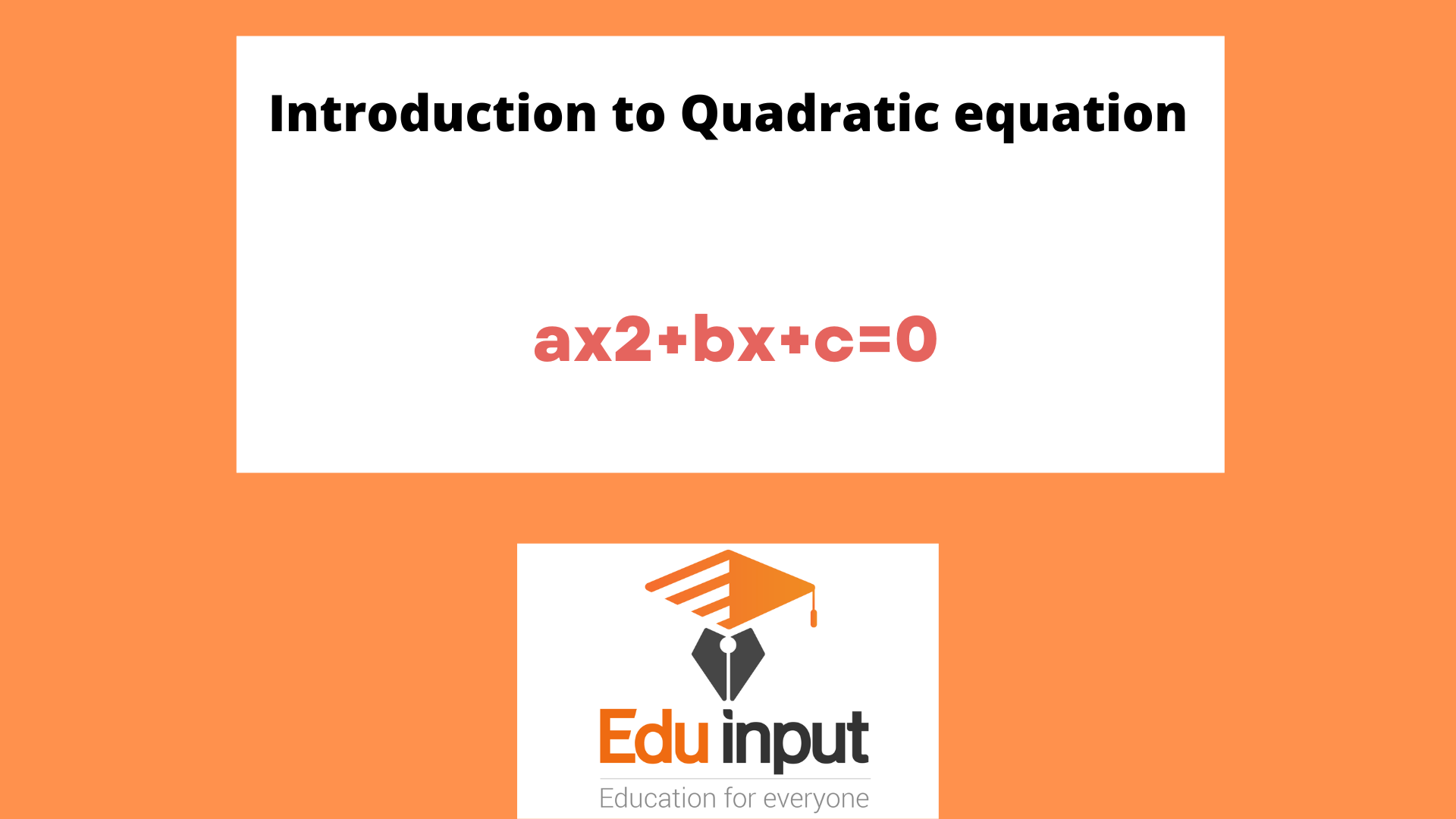
X²-1=0                                x²+1=0

X²=1                                    x²=-1

Square roots on both side

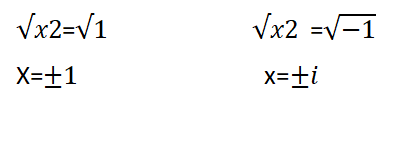
Hence four fourth roots of unity are:

+1,-1, +i , -i

±1 are called real roots of the fourth roots of unity

±i are called complex four-fourth roots of unity

Properties of fourth roots of unity

• The Sum of the fourth roots of unity is zero.

(1)+ (-1) + (i) + (-i) =0  

• Each complex roots of the four fourth roots of unity are conjugate of each other

I and-i are complex fourth roots of unity which are obviously conjugate of each other.

• Each complex root of four fourth roots of unity is the additive inverse of each other.

(i)+ (-i) =0

(-i) + (i) =0

• Real roots of four fourth roots of unity are additive inverse of each other

(1) + (-1) =0

(-1) + (1) =0

• Each complex roots of four fourth roots of unity is the multiplicative inverse of each other.

(i)(-i)=-i²=-(-1) =1

(-i)(i)= –i²=-(-1) =1               i²=-1

• Product of four fourth roots of unity is -1

(1)(-1)(i)(-i)= (-1) (-i²) = (-1) (-(-1))

(1)(-1)(i)(-i)= (-1) (1) =-1

Example:

           Find four fourth roots of unity of 16

Solution:

Let x be the four fourth roots of 16

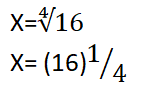
Taking power four on both side

X⁴= ((16) 1⁄4 ⁴

X⁴=16

X⁴-16=0

X⁴-4²=0

((x²)) ²-4²=0

By using

a ²– b²= (a-b) (a+b)

(X²-4)(X²+4)=0

X²-4=0                     x²+4=0

X²=4                         x²=-4

Square roots on both side

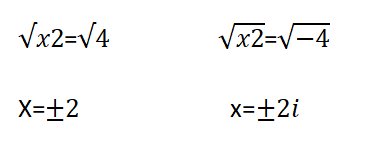
Hence four roots of 16

2,-2, 2i,-2i

Frequently Asked Question -FAQs