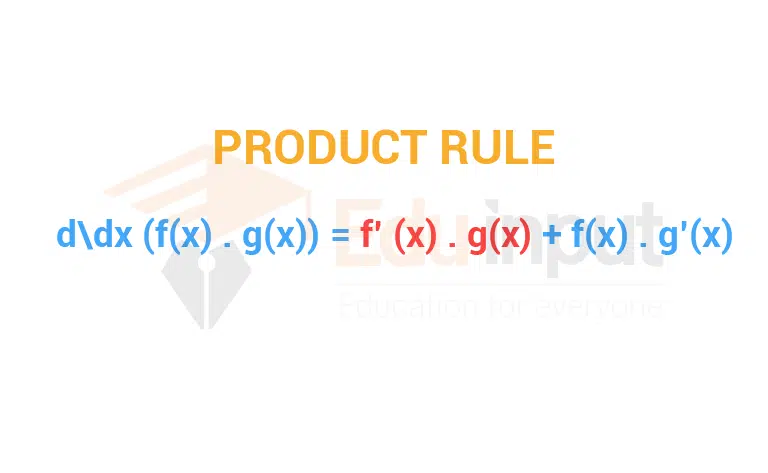
How to Apply the Product Rule of Differentiation?

In order to calculate the derivative of the function of the form h(x) = f(x)g(x), both f(x) and g(x) should be differentiable functions. We can apply the following given steps to calculate the derivation of a differentiable function h(x) = f(x)g(x) using the product rule.

Step 1: not down the values of f(x) and g(x)

Step 2: calculate the values of f’(x) and g'(x)

And apply the product rule formula

For example:

Find f'(x) for the following function f(x) using the product rule: f(x) = x·log x.

Solution:

Here, f(x) = x·log x

u(x) = x

v(x) = log x

⇒u'(x) = 1

⇒v'(x) = 1/x

⇒f'(x) = [v(x)u'(x) + u(x)v'(x)]

⇒f'(x) = [log x•1 + x•(1/x)]

⇒f'(x) = log x + 1

Result: The derivative of x log x using the product rule is log x + 1.

For example:

Y=sinx.cosx differentiated by using the product rule

Solution:

Y=sinx.cosx

Let u=sinx

V=cosx

By using the product rule

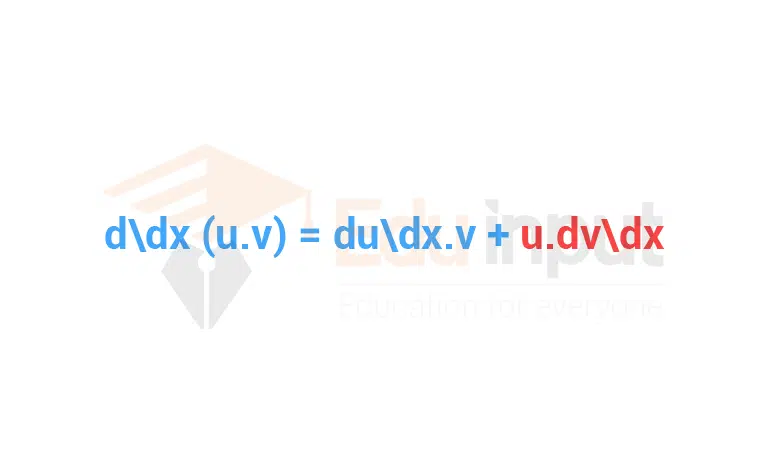
now ,

calculate the value du/dx and dv/dx

dv/dx=d/dx(v)

dv/dx=d/dx(cosx)

dv/dx=-sinx

du/dx=d/dx(sinx)

du/dx=cosx

put the value du/dx and dv/dx and u,v

dy/dx=sinx(-sinx)+cosx(cosx)

dy/dx=-sin²x+cos²x

dy/dx=cos²x-sin²x

Result: The derivative of sinx.cosx using the product rule is cos²x-sin²x