Mechanical Properties of Solids | Stress, and Strain

Mechanical properties of solids elaborate the characteristics such as the resistance to deformation and their strength.

Deformation of Solids

When an external force is applied to a body, it produces a change in shape, length, or volume. This change is called deformation.

When a soft rubber ball in hand is squeezed, the shape or value of the ball changes. If we stop squeezing the ball and open our hands, the ball will return to its original spherical shape.
If a rubber sting is held in hand and force is exerted at its ends, its length increases. This increase in length depends upon the applied force.

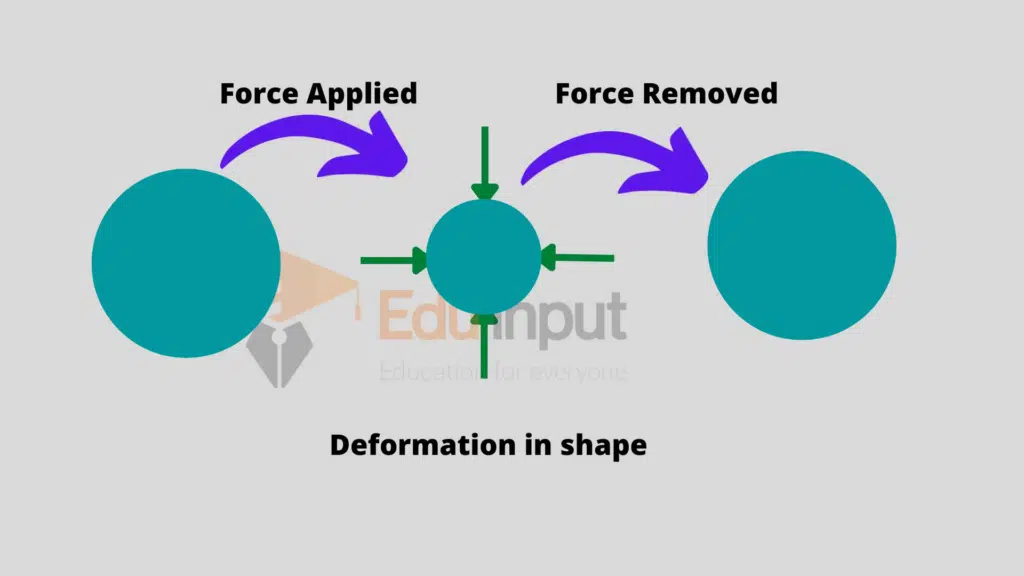
On removing the applied force the string will return to its original shape. This deformation is produced when an external force is applied to a body.

In crystalline solids, atoms are usually arranged in a certain order. Due to interatomic cohesive forces, these atoms are held about their equilibrium positions.

When the external force is applied to such a body, a distortion results because of the displacement of the atoms from their equilibrium position, and the body is said to be in a state of stress.

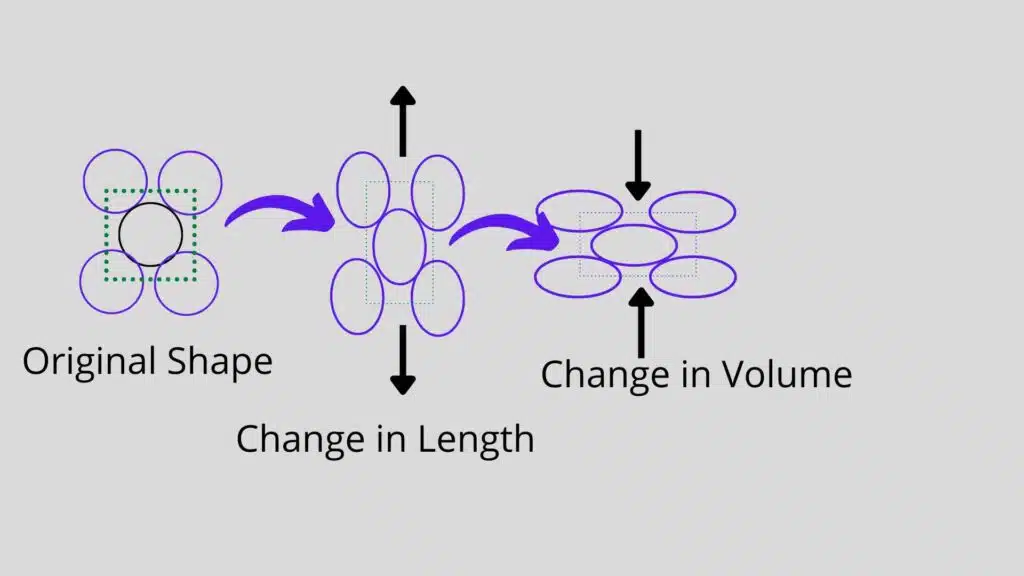
Due to this stress, there is a deformation in the body i.e. there is a change in the shape, length, or volume of the body.

When the external force is removed the atoms return to their equilibrium position, and the body regains its original shape. This regaining of the original shape depends that the applied force does not exceed the binding forces.

What is Elasticity?

The ability of a body to return to its original shape is called elasticity. The results of mechanical tests are usually expressed in terms of stress and strain. The stress is defined in terms of applied force and strain in terms of resulting deformation.

What is Stress?

It is defined as the force applied to the unit area to produce the change in the dimensions of a solid.

The change may be lengthwise or volume-wise in a body or shape.

Stress= Force/Area

σ=F/A

Unit of Stress

 The SI unit of stress is Nm^-2. It is also called Pascal (Pa).

Its dimension is [ML^-1T^-2].

There are many types of stress.

Tensile Stress

When stress changes the length of a body it is called tensile stress.

Volume Stress

When stress changes the volume of a body it is called volume stress or Compressional stress.

Shear Stress:

When stress changes the shape of a body it is called shear stress.

What is Strain?

Due to stress, there is a definite change in the shape of the body.

The degree of the change in shape is measured by strain and in the case of deformation in one dimension it is defined as a “fractional change in length”.

 If Δl is the change in length and l is the original length then mathematically it is given by

Strain = change in length/original length

ε= Δl/l

Since it is the ratio of the lengths, so the strain is dimensionless.

Tensile Strain:

If the strain is due to tensile stress, it is called tensile strain.

Volumetric Strain:

In case when the applied stress changes the volume, then the change in volume per unit volume is known as volumetric strain.

It is also called compressional strain. so the volumetric strain is

Volumetric strain= Change in Volume/Original Volume

Shear Strain:

When the stress is applied then due to this shear stress the shear strain is produced and is given by

γ=Δa/a=tanθ

For smaller values of θ as tan θ ≈ θ (radian)

So

γ = θ

Elastic constants:

The ratio of stress to strain is constant for a given material, provided that the force is not too great. This is called the modulus of elasticity.

Modulus of Elasticity=Stress/Strain

Since strain is a dimensionless quantity, so the units of modulus of elasticity are the same as that of stress i.e. Nm^-2 or Pa

Young’s Modulus:

The ratio of the tensile (or compressive) stress to the tensile (or compressive) strain is called Young’s modulus.

Mathematically

Young’s Modulus = (F/A)/ (Δl/l)

Bulk Modulus:

It is used for three-dimensional deformations, the ratio of the applied stress to the volumetric strain is called Bulk modulus.

Mathematically

K=(F/A) /(ΔV/V)

Here ΔV is the change in volume and V is the original volume.

Shear Modulus:

The ratio of the shear stress to shear strain is called the shear modulus.

Mathematically

G= (F/A) /tanθ