Rational Numbers and Irrational Numbers – Definition and Example
Rational numbers include integers, fractions, or decimals that end or repeat. An irrational number is any number that cannot be converted to a fraction.
Rational numbers
Those numbers which can be put into the form P/q where p, q ∈ Z and q≠0.the number √16, 3.7,4 are rational numbers. √16 can be reduced to the form p/q where p, q ∈Z and q≠0 because √16=4=4/1.rational numbers denoted by Q.
Q= {x|x=p/q, where p, q ∈ Z and q≠0.}
Irrational numbers
Those numbers which cannot be put into the form p/q where p, q ∈ Z and q≠0.the numbers
√2, √3 7/ (√5) are irrational numbers. Irrational number denoted by Q’.
Q’ = {x| x ≠p/q where p, q ∈ Z and q≠0.}
Decimal representation of rational and irrational Numbers:
The are four types of Decimal representation of rational and irrational Numbers:
Terminating decimals
A decimal which has only a finite number of digits in its decimal parts, is called a terminating decimal .thus 203.05, 0.00415, 1.456 are examples of terminating decimals.
Since a terminating decimal can be converted into a common fraction, so every terminating decimal represents a rational number.
Recurring decimals
This is another type of rational numbers.in general, a recurring or periodic decimal is a decimal in which one or more digits repeat indefinitely.
It well be show that a recurring decimal can be converted into a common fraction.so every recurring decimal represents a rational number.
Non -terminating decimals
A decimal which has an infinite number of digits in its decimal parts is called a non-terminating decimals. Thus 1.4235……, 0.013246…. , 2.33333333…, are examples of non-terminating decimal.
Non- recurring decimals
In general a non –recurring decimals is a decimal in which do not repeat digits in decimal part. Thus 1.5673….. are example of terminating decimal.
A non-terminating, non-recurring decimal is a decimal which neither terminates nor is it recurring.
It is not possible to convert such a decimal into a common fraction.
Thus a non-terminating and non-recurring decimal represents an irrational number.
Example
- O.34=34/100 is a rational number.
- 0.3333….=1/3 is recurring decimal, it is rational number
- 0.142857142857…..=1/7 is a recurring decimal is a rational number.
- 1.4142135… is an irrational number.
- 7.3205080…..is an irrational number.
- 3.141592……. is an important irrational number called
(pi) which denotes the constant ratio of the circumference of any circle to the length of diameter i.e.,
π =circumference of any circle /length of its diameter.
An approximate value of π (pi) is 22/7, a better approximation is 355/113 and a still better approximation is 3.14159… the value of π correct to 5 lac decimal places has been determined with help of computer .
Prove that √2 is irrational number
Proof: suppose that √2 is rational number.
Than,
√2=p/q → (a)
Where p, q ∈ Z and q≠0. Also let p/q is lowest form.
Squaring both sides equation (a) we get,
2=p2/q2
2q2= p2→ (1)
P2 is even number.
P x p is even number.
(Even x even=even)
(Odd x odd=odd)
So, p is an even number. Than it can be written as
P=2m → (2)
Using (2) in (1)
2q2= (2m) 2
2q2=4m2
q2=2m2 → (3)
This implies that q2 is an even number.
So, q also an even number. We can write it as
q=2n → (4)
From (2) and (4)
P/q=2m/2n
Which is not in lowest form. This is contradiction to our supposition that p/q in lowest form. Therefore,√2 is not rational number .it is irrational number.
Using the same method we can prove the irrationality of √3, √5, √7,…….√n where n is any prime number,
Prime number is denoted by P.
P= {2, 3, 5, 7, 11,…….}
Theorem:
Prove that sum of rational and irrational number is irrational number.
Proof: suppose that
rational + irrational =rational
P/q +x=m/n →(1)
∴ by definition rational and irrational number.
From (1)
X=m/n-p/q
X=mq – np/nq
X= (integer-integer)/integer
Where p, q, m, n ∈z
X=integer/integer
X must be rational number.
Which is contradiction to our supposition is wrong.
So, sum of rational and irrational number must be is irrational number.
Frequently Asked Question-FAQs
What are rational numbers? Give Examples.
A rational number is a number that can be expressed as a fraction p/q, where p and q are integers and q is not equal to 0.Some examples of rational numbers include 1/3, 2/4, 1/5, 9/3, and so on.
Is 0 a rational number?
Yes, 0 is a rational number because it is an integer that can be written in any form such as 0/1, 0/2, where b is a non-zero integer. It can be written in the form: p/q = 0/1.
Therefore, we can conclude that 0 is a rational number.
What is the denominator of the rational number?
The denominator of a rational number can be any real number except 0.
what is an irrational Number?
An irrational number is any real number that cannot be expressed by a rational number. It is a number that cannot be represented as a finite or terminating decimal.
Is Pi(π) a rational number?
No, Pi (π) is not a rational number because its value equals 3.142857…

 written by
written by 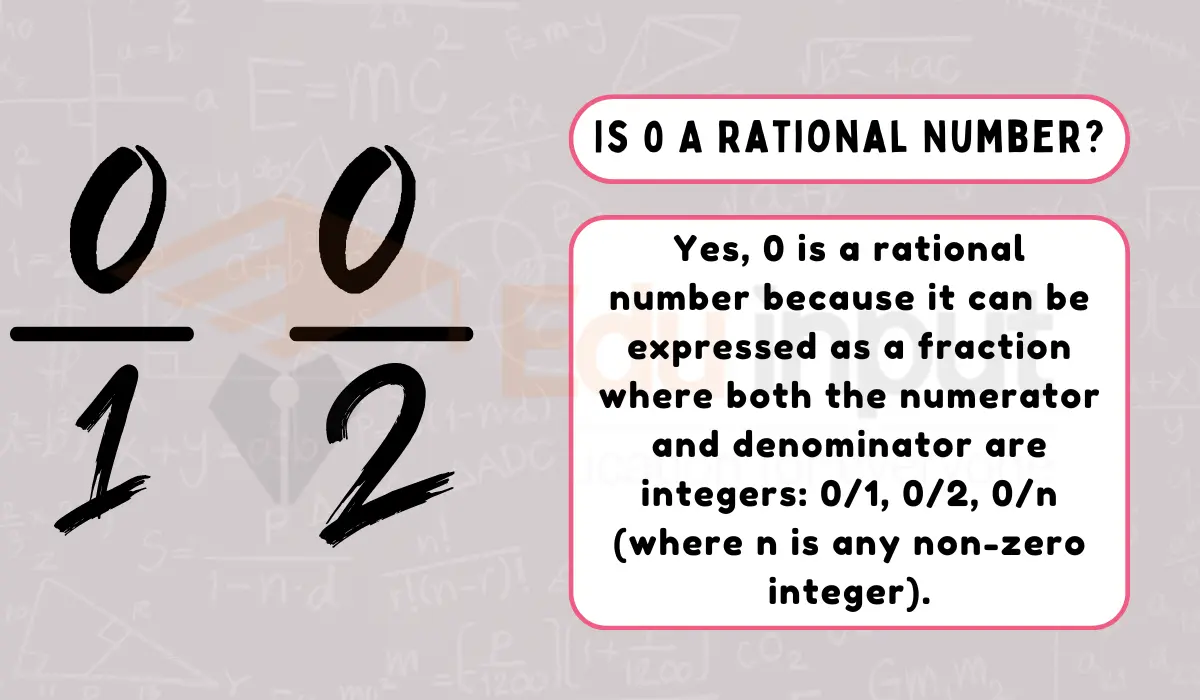





Leave a Reply