Geometrical Representation of Complex Numbers
We have seen that there is a (1, -1) correspondence between the elements (ordered pairs) of the Cartesian plane R x R and the complex numbers.
Therefore, there is a (1, -1) correspondence between the points of the coordinate plane and the complex numbers. We can, therefore, represent complex numbers by points of the coordinate plane.
How do we represent a complex number geometrically?
In this representation, every complex number will be represented by one and only one complex number. The components of the complex number will be the coordinates of the points representing it.
In this representation, the x-axis is called the real axis and the y-axis is called the imaginary axis. The coordinate plane itself is called the complex plane or z-plane.
By way of illustration, several complex numbers have been shown below in fig.
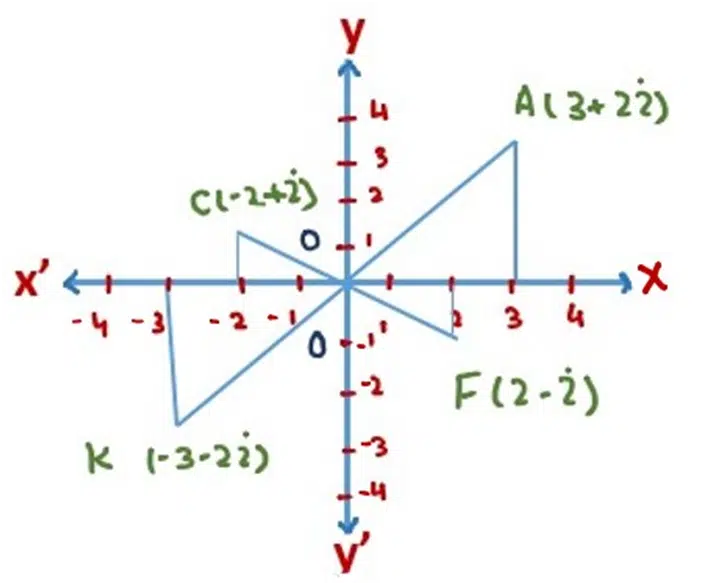
The figure representing one or more complex numbers on the complex plane is called an argand diagram. Points on the x-axis represent real numbers whereas the points on the y-axis represent imaginary numbers.
x and y are the coordinates of a point.
It represents the complex number x + iy. The real number√(a2 + b2) is called the modulus of the complex number a + ib.
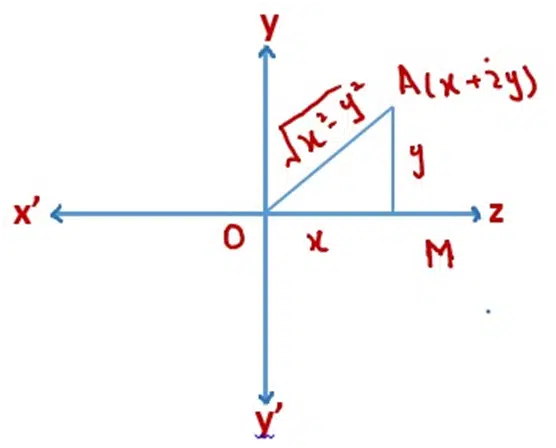
In the figure:
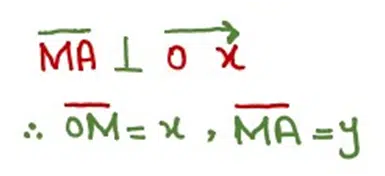
In the right-angled triangle OMA, we have, by Pythagoras theorem.
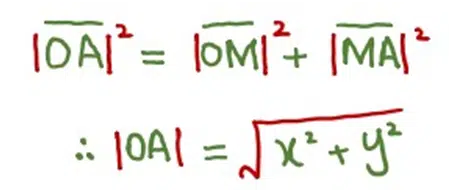
Thus |OA| bar represents the modulus of x + iy. In other words: the modulus of a complex number. The modulus of a complex number is generally denoted as |x + yi| or |(x,y)|. For convenience, a complex number is denoted by z.
If z = x + iy + (x,y), then
|z| = √x2 + y2
The polar form of a Complex number
Consider adjoining representing the complex number z = x + i.
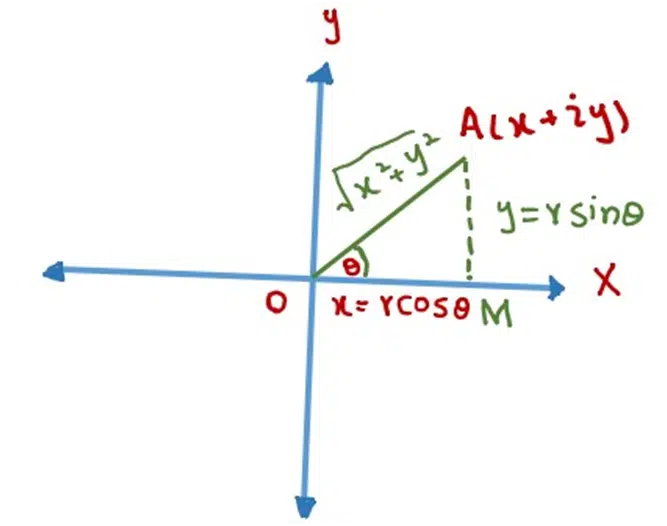
From the diagram, we see that x = rcosθ and y = rsinθ where r = |z| and θ is called arguments of z.
Hence
x + iy = rcosθ + irsinθ … (i)
where
r = √(x2 + y2) and θ = tan-1y/x
Equation (i) is called the polar form of the complex number z.
Express the complex number 1 + i√3 in polar form.
Step-I:
put rcosθ = 1 and + rsinθ = √3
Step-II:
r2 = (1)2 + (√3)2
r2 = 1 + 3 = 4
r = 2
Step-III:
θ = tan-1√3/1
θ = tan-1√3 = 60o
thus
1 + i√3 = 2 cos 60o + i2sin60o

 written by
written by