Centripetal Force And Centripetal Acceleration

Centripetal force and centripetal acceleration are two important concepts in physics that are related to circular motion. Centripetal force is the force that keeps an object moving in a circular path, and centripetal acceleration is the acceleration that an object experiences when it is moving in a circular path.

Download the pdf notes of centripetal force and centripetal acceleration class 11 physics

What is Centripetal Force?

The force that causes the body to follow a curved path is called centripetal force. Its direction is always orthogonal to the movement of the body and the fixed point at the center of the momentary curvature of the path.

Types of Centripetal Force

There are many different types of centripetal force, but some of the most common ones include:

The tension force in a string, which keeps a ball on a string moving in a circular path.
The gravitational force, which keeps the moon in orbit around the Earth.
The frictional force, which keeps a car from skidding around a curve.

Centripetal Force Examples

•  Force acting on an electron in fixed orbits around the nucleus.

• Force acting on satellites around the earth.

• Force acting on Earth around the sun.

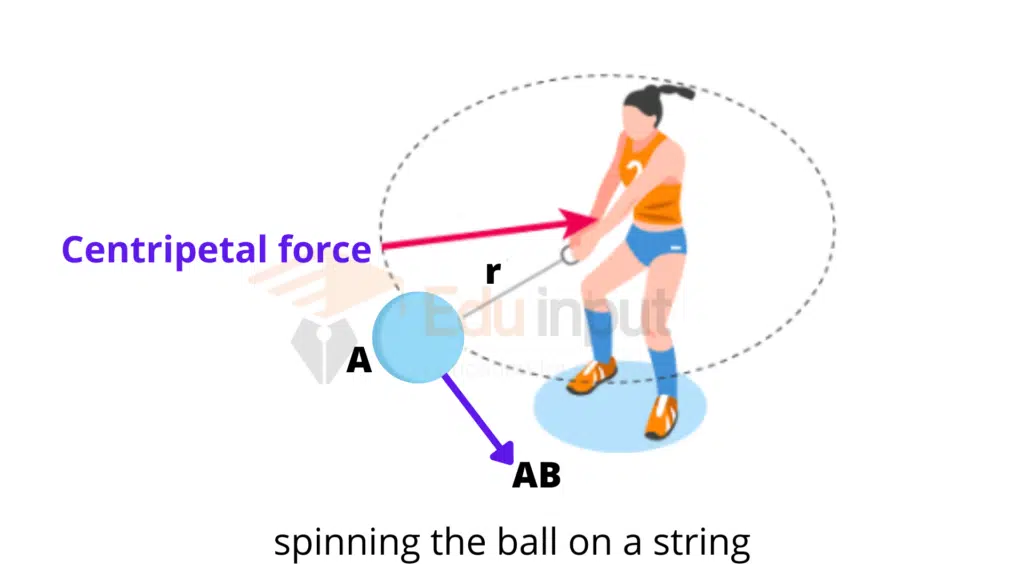
Consider a ball attached at one end of a string and whirled in a horizontal circle. If the string snaps, the ball will not continue its motion in a circular path.

It will go straight along the tangent to the circle. If the string snaps, when the ball is at point A, then the ball will follow the straight line path AB.

This proves that a force is provided by some mechanism, which keeps the ball (body) moving along the circular path. This force is known as centripetal force.

The force needed to bend the normally straight path of the particle into a circular path is called the centripetal or center-keeping force.

Centripetal Force Formula

F_c=ma_c= mv²/r

Fc is the centripetal force
m is the mass of the object
v is the speed of the object
r is the radius of the circle

But according to the relation between angular and linear velocities

v=rω

So

F_c=mr²ω²/r

It is directed towards the center of the circle in the direction of centripetal acceleration.

What is Centripetal Acceleration?

Centripetal acceleration is the acceleration that an object experiences when it is moving in a circular path. This acceleration is always directed towards the center of the circle, and it is equal to the square of the speed of the object divided by the radius of the circle.

Centripetal acceleration formula

Consider a particle moving along a circle with a uniform speed V. If the particle moves from point A to B then the magnitude of its velocity remains the same but the direction of velocity changes.

Acceleration of the particle is given by

a= Δv/Δt

Where Δt is the time taken by the particle to travel from point A to B.

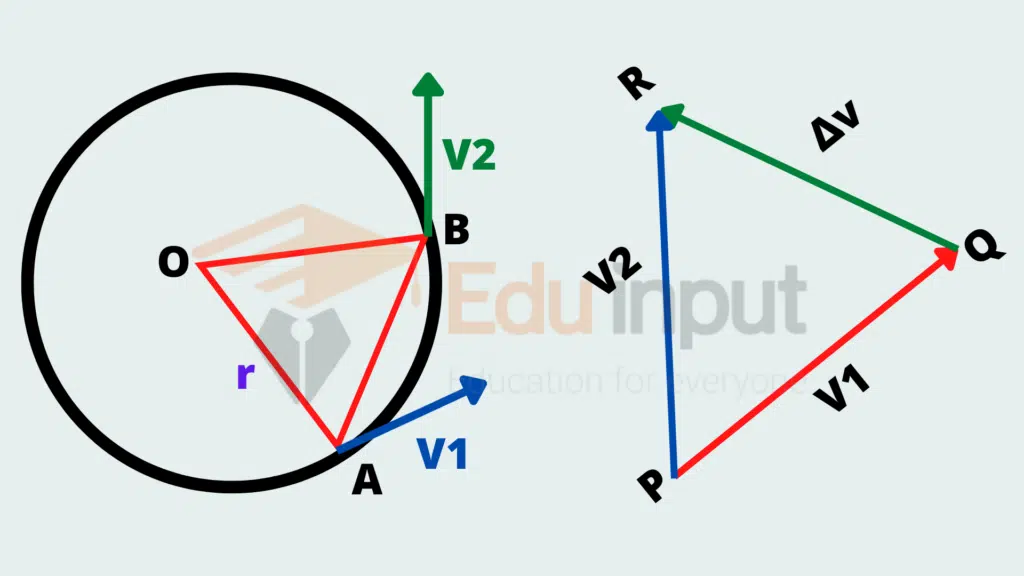
Let v₁ and v₂ be the velocities of particles at A and B respectively but

|v₁|=|v₂|=|v|=v

Then time taken by particle to cover distance ‘s’ is given by;

Δ t= S/V

a= Δv/s/v =V(Δv/s)

let us now draw a triangle PQR such that PQ is parallel and equal to v₁ and PR is parallel and equal to v₂.

As OA is perpendicular to v₁ and OB is perpendicular to v₂.

Therefore angle AOB and angle QPR are equal. Both triangles are isosceles. Because V₁=V₂=V And OA=OB

And angles between their equal arms are equal. Hence triangle OAB and triangle PQR are similar.

Δv/v= AB/r

Now if Δ t→0 then point B approaches to point A and arc AB becomes nearly the same in length as line AB.

AB=s

Δv/v= s/r

Δv=v(s/r)

As

a=v (Δv/s)

So

a= (v/s)v(s/r)

a=v²/r

As this acceleration is caused by centripetal force so it is known as centripetal acceleration and is denoted by a_c

SO

a_c=v²/r

The direction of Centripetal Acceleration

 As PQ is perpendicular to OA and PR is perpendicular to OB thus QR is perpendicular to AB. Hence Δv is perpendicular to QR and is directed toward the center of the circle. Also when ABà0 the centripetal acceleration is direct along the radius in the direction of Δv. Hence a is also directed towards the center of the circle.

Related FAQs