10 Examples of Common Denominators
In mathematics, finding a common denominator is an important skill. A common denominator is a number that is a multiple of the denominators in a set of fractions, allowing for easier addition, subtraction, and comparison of fractions.
In this article, we will discuss ten examples of common denominators in mathematics.
Examples of Common Denominators
These are 10 examples of common denominators.
1: Adding and Subtracting Fractions
When adding or subtracting fractions, a common denominator is needed.
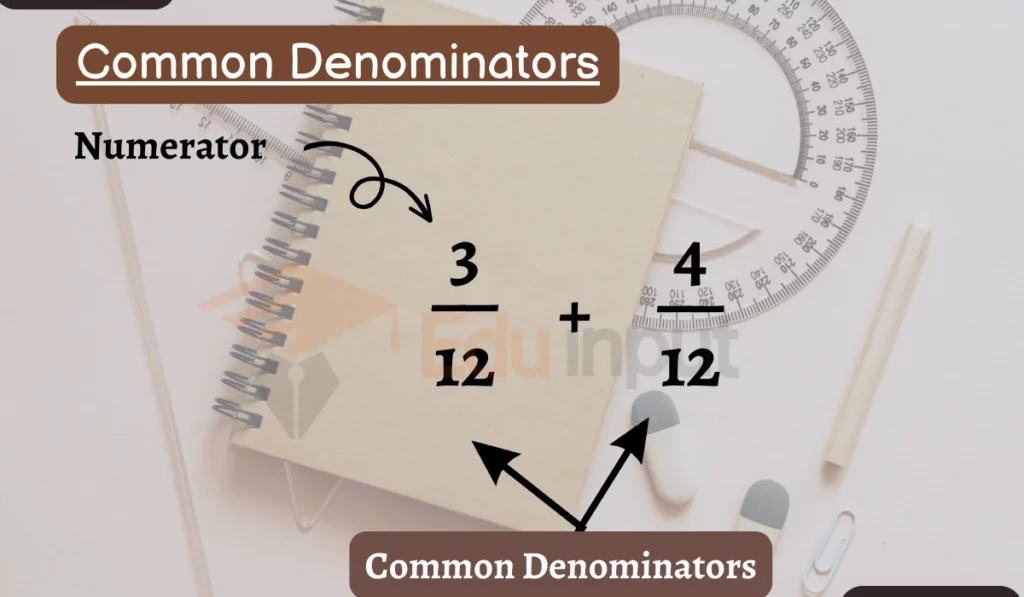
For example, to add 1/4 and 1/3, you need to find a common denominator. In this case, the common denominator is 12, so 1/4 becomes 3/12, and 1/3 becomes 4/12, making the addition straightforward,
3/12 + 4/12 = 7/12
2: Comparing Fractions
To compare fractions with different denominators, finding a common denominator is essential.
For example, to compare 3/5 and 2/7, you can find a common denominator, such as 35, to see which fraction is larger.
3: Fractional Conversion
In everyday life, you need to convert fractions with different denominators into fractions with a common denominator.
For example, in a recipe that calls for 1/4 cup and another that requires 1/3 cup, you convert both to 12ths for consistency.
4: Dividing Fractions
When dividing fractions, it can be helpful to express them with a common denominator.
For example, dividing 1/3 by 2/5 is simplified by finding a common denominator, making it 5/15 divided by 6/15.
5: Equivalent Fractions
Equivalent fractions are fractions that represent the same value but may have different denominators.
For example, 1/2 and 3/6 are equivalent fractions because they share a common denominator of 6.
6: Math Tests and Homework
Common denominators are frequently encountered in math tests and homework. Where students are required to perform operations on fractions.
7: Proportions and Ratios
Proportions and ratios often involve comparing fractions, which requires finding a common denominator.
8: Fractional Measurements
In real-world measurements, common denominators are essential.
For example, when measuring lengths in inches and feet, it’s important to convert to a common denominator.
9: Financial Calculations
In financial calculations, dealing with fractions is common. Finding a common denominator simplifies interest rate calculations.
10: Engineering and Construction
In engineering and construction, measurements and calculations frequently involve fractions.



Leave a Reply