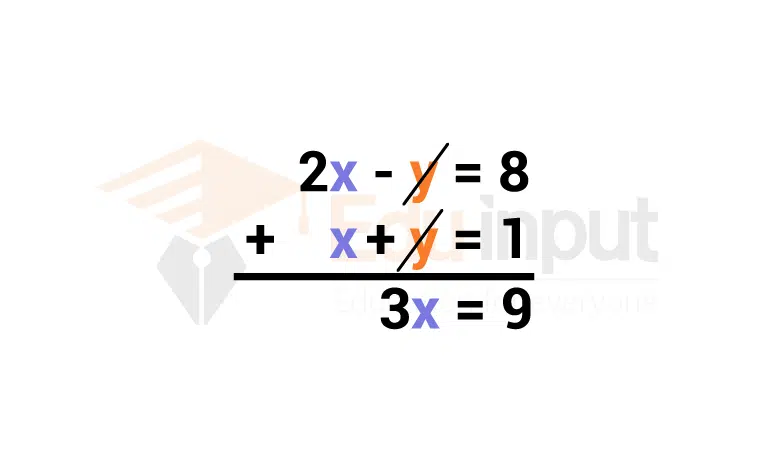Substitution Method Mean in Math?

One of the methods to solve a system of linear equations in two unknown variables algebraically is the substitution method. In this method, we find the value of any one of the unknown variables by isolating it on one side and taking every other term on the other side of the equation.

Then we substitute that value in the second equation. It includes simple steps to find the values of variables of a system of linear equations by the substitution method. Let’s this article learn about it in detail.

What is the substitution method?

The substitution method is a simple method to solve a system of linear equations algebraically and find the solutions to the unknown variables.

 As the name offer, it includes finding the value of the x-variable in terms of the y-variable from the first equation and then substituting or putting the value of the x-variable in the second equation.

In this method, we can solve and find the value of the y-variable. And at last, we can put the value of variable y in any of the given equations to find the value of variable x this method can be interchanged as well where we first solve for x and then solve for.

Definition of substitution method

The substitution method is one of the algebraic way to solve a system of linear equations. It includes substituting the value of any one of the unknown variables from one equation to another equation.

 The other two algebraic methods of solving linear equations are the cross multiplication method and the elimination method. Apart from the algebraic process, we can also solve a system of linear equations graphically.

For example: solving two linear equations x+2y=7and x+y=1

 Using a substitution method.

Solution:

Substitution Method

x+2y=7…>(1)

x+y=1…>(2)

From equation (2)

X=1-y

Substitute this value equation (1)

1-y+2y=7

Y=7-1

Y=6

Substitute this value equation (2)

X+6=1

X=1-6

X=-5

X=-5 and y=6

Solving the system of linear equations by substituting the method

The steps to the substitution method or application to solve a system of linear equations are given below:

Step 1: Simplify the given linear equation for two variables by expanding the parenthesis if needed.

Step 2: Solve any one of the linear equations for any one of the variables. You can use any unknown variable based on the ease of calculation.

Step 3: Substitute the obtained value of x or y to solve any one of the linear equations in the other linear equation.

Step 4: Now, the new equation is obtained using arithmetic operations and solving the equation for one variable.

Step 5: Now, substitute the value of the unknown variable from Step 4 in any of the given equations to solve for the other variable.

For example:

3X+2Y=8…>(1)

X+y=4..>(2)

From equation 2

X=4-y

Substitute this value equation (1)

3(4-y)+2y=8

12-3y+2y=8

-y=8-12

-y=-4

Y=4

Substitute this value equation (2)

X+4=4

X=4-4

X=0

X=0 and y=4