EXPRESSION FOR ENERGY OF ELECTRON | kinetic energy of electron formula

The total energy ‘E’ of an electron is the sum of kinetic energy and potential energy.

                            E = K.E + P.E

                            K.E = ½ mv²

The expression for the potential energy can be calculated by integrating the amount of force of attraction between the nucleus and the electron. This integration is done from infinity to r. The constant factors are taken on L.H.S. of integration sign.

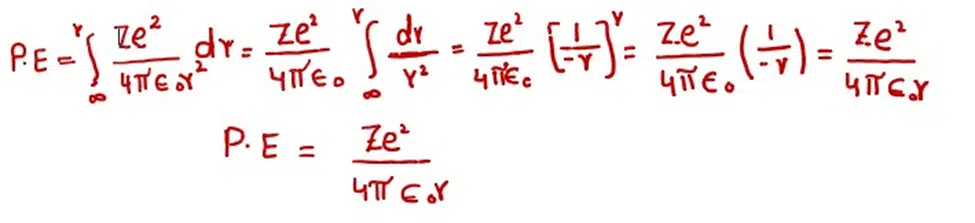
During integration, we have imagined that the electron is at infinity from the nucleus. When it is brought near to the nucleus up to the distance ‘r’ then certain amount of energy is evolved by the electron. This energy is given by equation.

Substituting equation (12) and (13) in equation (11)

 E = ½ mv² + (-ze²/4π ∈_or)

E = ½ mv² – ze²/4π ∈_or —> (14)

Equation (14) can only be useful, when we know the factor v² (velocity), which is impossible to determine. We eliminates the factor of velocity from this equation by using equation (4)

             mv² = ze²/4π ∈_or —> (4)

Multiply equation (4) by ½ on both sides

       ½ mv² = ze²/8π ∈_or —> (15)

Now put equation (15) into (14)

     E = ze²/8π ∈_or – ze²/4π ∈_or

       E = – ze²/8π ∈_or —> (16)

According to equation (16) the energy of the moving electron is the negative inverse of radius of the orbit. So, when the radius of the orbit increasing the energy also increase.

Let us put the expression for radius from equation (7) in equation (16)

           r = n²h²∈_o /πmZe² —> (7)

           E_n = z^ee⁴m/8∈_o²n²h²

           E_n = – e⁴m/8∈_o²h² x (z²/n²) —> (17)

All those parameters which are outside the brackets are constant. We can calculate this collection of constant by putting the values of e, m, ∈_o and h.

  e = 1.602 x 10^-19C, m = 9.1 x 10^-31kg, π= 3.1416

  h = 6.625 x 10^-34Js, ∈_o = 8.854 x 10^-12 C²J^-1 m^-1

       e⁴m /8∈_o²h² = 2.18 x 10^-18J

      E_n = -2.18 x 10^-18 (z²/n²) J

For hydrogen atom

              E_n = – 2.18 x 10^-18 (1/n²) J —— (18)

According to equation (18), the energy of electron in hydrogen atom is the negative inverse of n², It means that the greater the value of n, greater the energy of the electron.

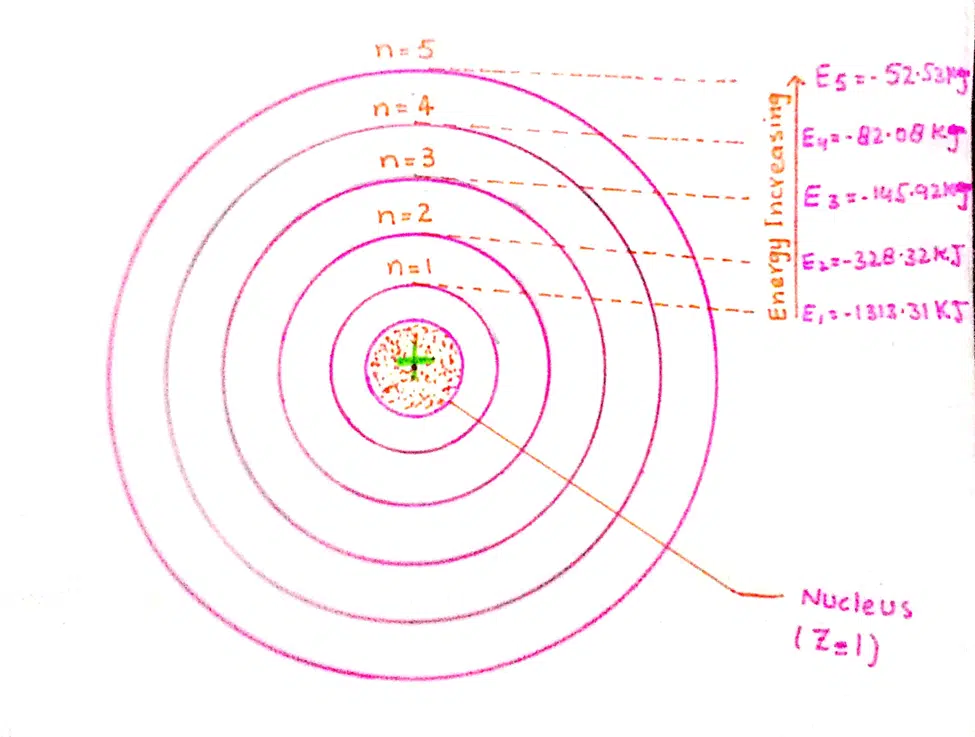
Energies of first five orbit of hydrogen atom

E₁ = – 2.18 x 10^-18 (1/1²) J = – 2.18 x 10^-18J

E₂ = – 2.18 x 10^-18 (1/4) J = – 0.54 x 10^-18J

E₃ = – 2.18 x 10^-18 (1/9) J = – 0.24 x 10^-18J

E₄ = – 2.18 x 10^-18 (1/16) J = -0.14 x 10^-18J

E₅ = – 2.18 x 10^-18 (1/75) J = – 0.08 x 10^-18J

The amount of energy associated with the electron goes on increasing (it becomes more and more less negative).

It is clear from these values that the energy difference between adjacent levels goes on decreasing for the hydrogen atoms.

E_{2 –} E₁ > E₃ – E₂ > E₄ – E₃ > E₅ – E₄ …

From these energy values and their energy differences, we can very easily guess the amounts of energies that are required to shift the electrons between any two orbits. The shifting of electrons from n = 1 to n = 2 requires approximately five times more energy than from n₂ to n₃.

ENERGY CALCULATIONS IN kJ MOL^-1

Equation (18) gives the energy of the electron when it is moving around the nucleus. The value of energy is negative and shows that the electron is bounded by the nucleus. In other words, the electron is under the force of attraction of the nucleus.

The value of the energy of an electron is in joules atom^-1. If this quantity is multiplied by Avogadro’s number and divided by 1000, the value of E_n is in kJ molJ^-1

E_n = 2.18 x 1^-18 x (6.02 x 10²³ / 1000.n²) kJ mol^-1

E_n = (-1313.315/n²) kJ mol^-1

This energy is associated with one mole of hydrogen atoms i.e. 1.008 g.

Calculations of Energy for various Orbits

When we substitute the value of ‘n’ as 1, 2, 3, 4, 5,… etc. in the above equation, then, values of energies in various orbits are calculated.

These values of energies (6.02 x 10²³) are for the atoms of H.

E₁ = 1313.315/1² = – 1313.315 kJ mol^-1

E₂ = 1313.315/2²= -328.32 kJ mol^-1

E₃ = 1313.315/3² = 145.92kJ mol^-1

E₄ = 1313.315/4² = -82.08kJ mol^-1

E₅ = 1313.315/5² = -52.53 kJ mol^-1

Eα = 1313.315/α² = 0kJ mol^-1 (Energy when an electron is free from the nucleus) When we put the number of orbits as infinity, it means that the electron is free from the nucleus.

The value of energy differences between adjacent orbits are as follows:

E₂ – E₁ = (-328.32) – (1313.315) = 984.99 kJ mol^-1

E₃ – E₂ = (-145.92) – (-328.32) = 182.40 kJ mol^-1

E₄ – E₃ = (-82.08) – (-145.92) = 63.84 kJ mol^-1

These differences go on to decrease in higher orbits. The energy level becomes closely spaced.

E₂ – E₁ > E₃ – E₂ > E₄ – E₃

The energy difference between the first and the infinite level is

E∞ – E1 = 0 – (- 1313.315) = 1313.315 kJ mol^-1

Ionization potential hydrogen

This 1313.315 kJ mol^-1 is the ionization energy of hydrogen. This is just the same as determined experimentally. So, Bohr’s model of the hydrogen atoms can justify the ionization potential of hydrogen.

FORMULA OF ENERGY DIFFERENCE

The formula of energy difference can be calculated as follow. Let the energy associated with the electron in any lower level (n₁) is E₁ and any higher level (n₂) be E₂. (It is not necessary that these two levels are adjacent to each other).

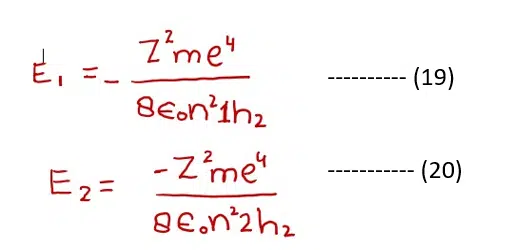
Subtracting equation (19) from (20)

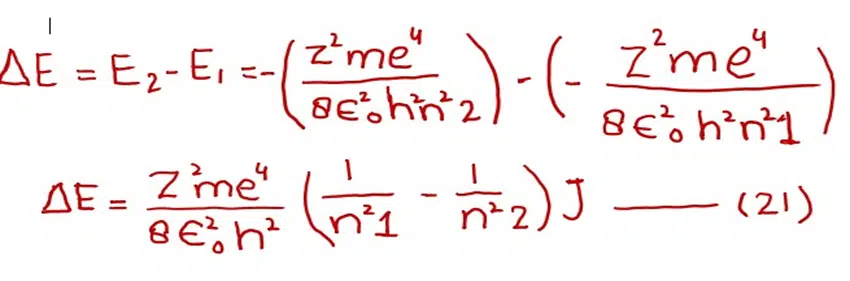
We know that

Remember, the energy difference of any two orbits will be positive.

With the help of equation (22), we can calculate the energy difference between any two levels. n1 is the lower level and n2 is the higher level. This equation convinces us that the energy difference between adjacent levels goes on decreasing from the lower to the higher levels. When we multiply 2.18 x 10-18 J with Avogadro’s number (6.02 x 1023) and divide by 1000, we get the factor for one mole of H-atoms. So

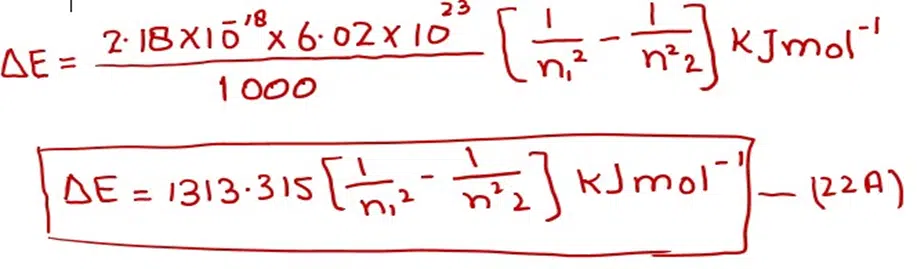
By putting various values of n₁ andn₂, one can get the energy difference for one mole of hydrogen for any two orbits.

IONIZATION POTENTIAL OF HYDROGEN

If sufficient energy is given to 1 mole of hydrogen atoms to ionize all the atoms of hydrogen then the electrons go from n₁ = 1 to n₂ = ∞. By putting these values in equation (22A)

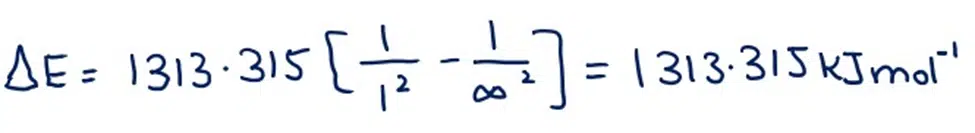
This 1313.315kJ.mol^-1 is the theoretical value of the ionization potential of hydrogen, which is very close to the experimental value of 1313.315kJ mol^-1.

CALCULATION OF THE FREQUENCY OF PHOTON

Whenever the electron jumps in the hydrogen atom from one orbit to the other orbit, then a photon is emitted or absorbed. If we want to determine the frequency of the photon then equation (21) may be changed as follows: Since

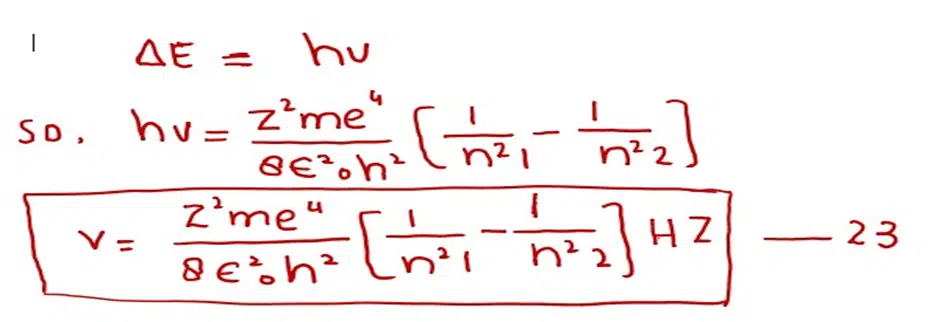
After doing the calculations of the factors outside the brackets in equation (23) we get (putting Z= 1 for H- atom). The following equation

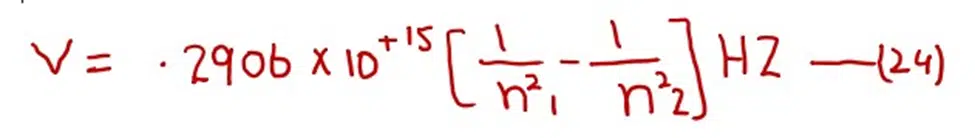
Frequency of the photon is measured in Hertz. The values of frequencies of photons emitted or absorbed go on decreasing among the higher orbits as compared to the lower orbits.

CALCULATION OF THE WAVE NUMBER OF PHOTON

In order to know the wave number of the photon, which is emitted or absorbed, let us modify equation (22)

Since,

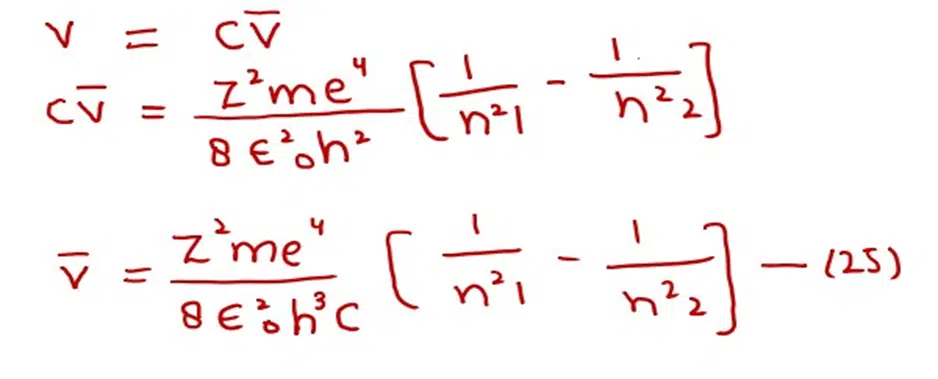
Where

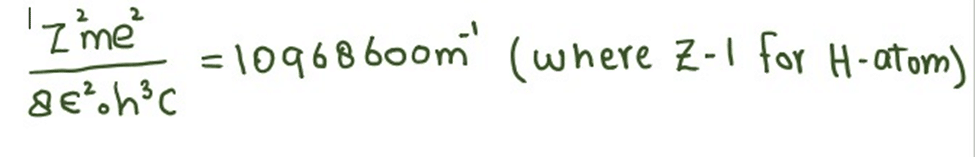
The unit of calculated factor is m^-1

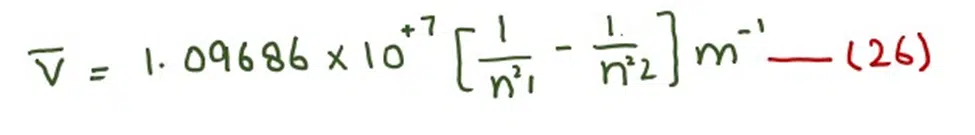
According to equation (26), we can calculate the wave number of all those photons which are emitted or absorbed during the jumping of electrons.

Reated articles

DISTRIBUTION OF ELECTRONS IN PERIODS AND GROUPS OF THE PERIODIC TABLE

HEISENBERG’S UNCERTAINTY PRINCIPLE-In depth explanation

Experimental Verification of dual Nature of Matters