Quantum numbers-Principle, Azimuthal, Magnetic and Spin
What are quantum numbers?
Quantum numbers are the sets of numerical values which give a well-behaved solution to the Schrodinger wave equation.
Quantum numbers are used to identify various energy levels in which the electron can exist. The values of quantum numbers are specified, when the Schrodinger equation is solved for the hydrogen atom.
There are four quantum numbers, which are required to completely specify the character of an electron:
(i) Principle quantum number (n)
(ii) Azimuthal quantum number (I)
(iii) Magnetic quantum number (m)
(iv) Spin quantum number (s)
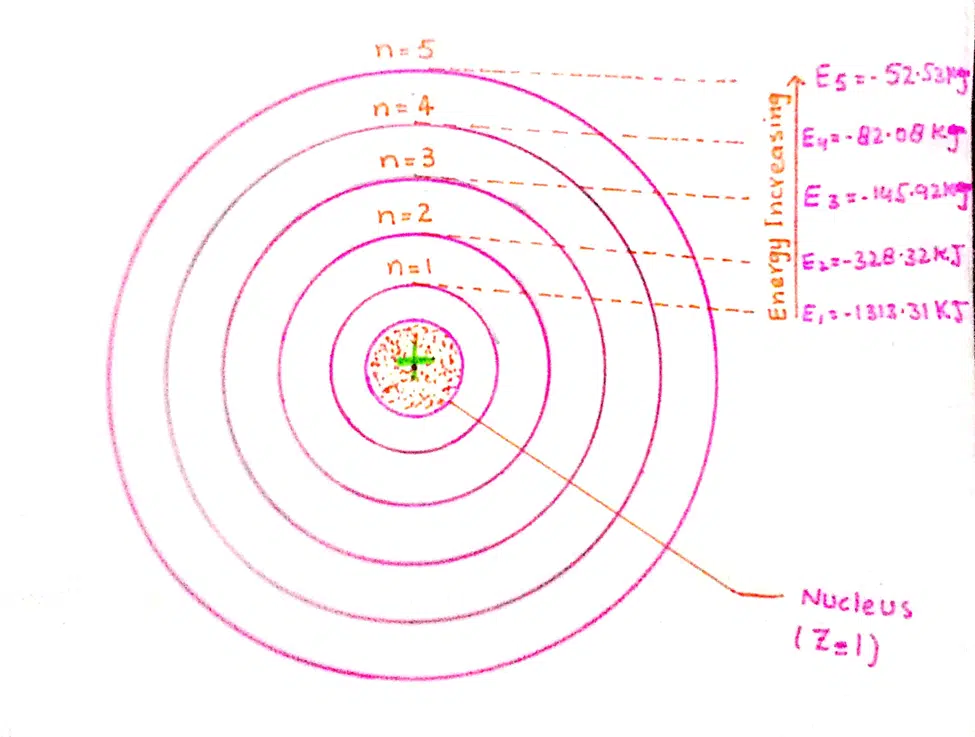
PRINCIPAL QUANTUM NUMBER
This quantum number tells us the distance of the electron from the nucleus and the energy of the electron.
Its value is
n = 1, 2, 3, 4 …∞
When we study Bohr’s atomic model, we represent the energy level by n. This value of ‘n’ gives us the value of the radius of the orbit.
r = 0.529(n2) Ao
Its means the value of ‘n’ in the Schrodinger wave equation tells us the distance of the electron from the nucleus.
When the Schrodinger wave equation is solved for H- atom, and it is supposed that motion is circular this equation for radius comes out to the same as given by Bohr.
The energy formula of Bohr’s model also involves ‘n’.
E = -2.18 x 10-18 (1/n2) J
The greater the value of ‘n’, the greater the value of the energy of the electron.
AZIMUTHAL QUANTUM NUMBER
This quantum number tells us the shape of the orbital.
It is represented by values as
ℓ = 0, 1, 2, 3, 4, 5 …. (n -1)
There are four types of orbitals and they have four different shapes.
| Value of l | Orbital | Shape |
| ℓ = 0 | s- orbital | spherical |
| ℓ = 1 | p- orbital | Dumb-bell |
| ℓ = 2 | d- orbital | sausage shape |
| ℓ = 3 | f- orbital | more complicated |
The names of orbitals are based on the types of lines in atomic absorption. So, stands for sharp, p for principle, d for diffuse, and f for fundamental.
Relationship between principal and azimuthal quantum number
The relationship between principalal and azimuthal quantum number is as follows:

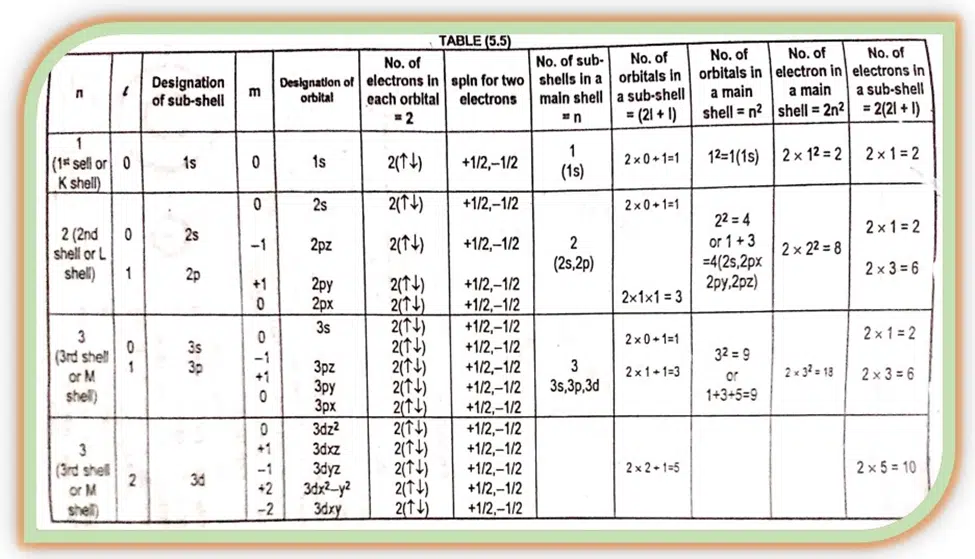
The values of ‘ℓ’ enable us to calculate the total number of electrons in a given subshell. The formula for electrons in a given ℓ value is 2 (2l + 1).
When
| ℓ = 0 | total electrons= 2 | for s- orbital |
| ℓ = 1 | total electrons= 6 | for p- orbital |
| ℓ = 2 | total electrons= 10 | for d- orbital |
| ℓ = 3 | total electrons= 14 | for f- orbital |
MAGNETIC QUANTUM NUMBER
This quantum number tells us the orientation of the orbital in space.
It is represented by ‘m’. The word orientation means the relative position of one with respect to the other. The possible values of ‘m’ depend upon the nature of the orbital.
When
| ℓ = 0 | s- orbital | m = 0 |
| ℓ = 1 | p- orbital | m = -1, 0, +1 |
| ℓ = 2 | d- orbital | m = +2, +1, 0, -1, -2 |
| ℓ = 3 | f- orbital | m = +3, +2, +1, 0, -1, -2, -3 |
The above relationship shows that for a given value of ‘ℓ’ the total number of ‘m’ values are (2l + 1). The table helps us to understand the relation of all these quantum numbers.
The symbol s, p, d, and f, stands for sharp, principle, diffused, and fundamental.
These are spectroscopic terms and stand for the types of spectral lines of atoms.
The different values of ‘m’ for a given value of ‘ℓ’ gives us the total number of different ways in which a given s, p, d, f sub- shells can be arranged in space along the x, y, and z axis.
This arrangement is done in the presence of a magnetic field. It gives us the total number of different space orientations to the given s, p, d, and f sub-shells.
This is also called the orbital orientation quantum number.
For s sub- shell ℓ= 0 so m= 0. It implies that s sub- shell of an energy level has only one space orientation. It can be arranged in space in the only way along the x, y, and z axis. So s- sub- shell has only one orbital.
For p sub-shell ℓ= 1, m can have three values (0, +1). It implies that a p sub- shell of any energy level has three space orientations.
It can be arranged in space in three different ways along the x, y, and z- axis, they are designated as px, py, pz with m= 0, +1, and -1, respectively. All the three p- orbitals are dumbbell-shaped.
Each has two egg-shaped p- orbitals and hence directional character. It gives geometry and properties to the molecule produced when orbitals overlap with orbitals of their atoms.
For d sub- shell ℓ = 2, m has five values (0, +1, +2). It has five space orientations, and named as dxy (m= -2), dxy (m= -1), dxy (m +1) dx2– y2 (m = +2) dz2(m= 0)
Similarly, the f sub- shell has ℓ= 3, and ‘m’ has seven space orientations.
SPIN QUANTUM NUMBER
This quantum number tells us the spin of the electron whether clockwise or anti-clockwise. It is represented by ‘s’. Actually, the electron has two types of motions. The orbital motion is around the nucleus and spin motion around its own axis.
This motion around its own axis is called self-rotation. Due to the spin motion, the electron creates a magnetic field and hence the magnetic moment. The spinning of electrons in clockwise and anti-clockwise directions produces an opposite magnetic field.

Clockwise spin motion is represented by 1 or +1/2.
Anti-clockwise spin motion is represented by 1 or -1/2.